Xenon oscillation analysis in Monte Carlo burnup calculation
-
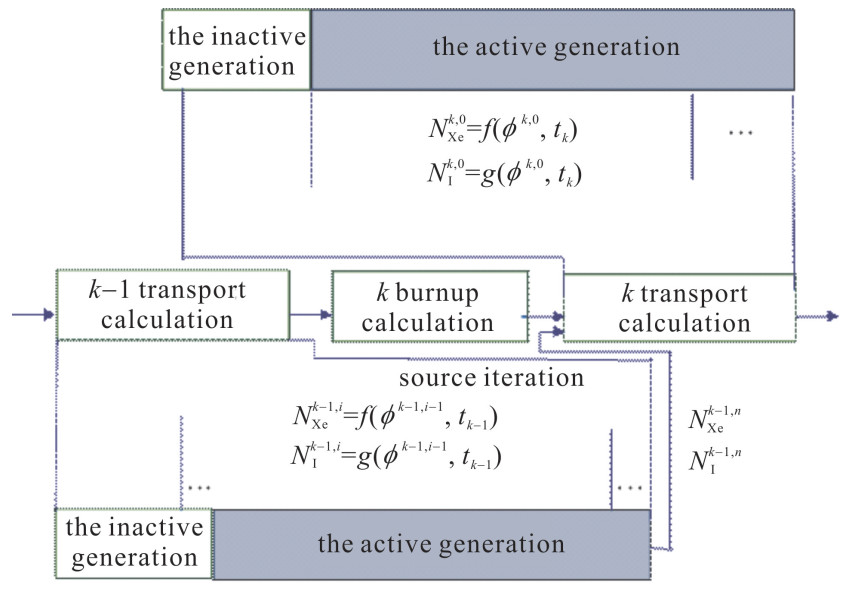
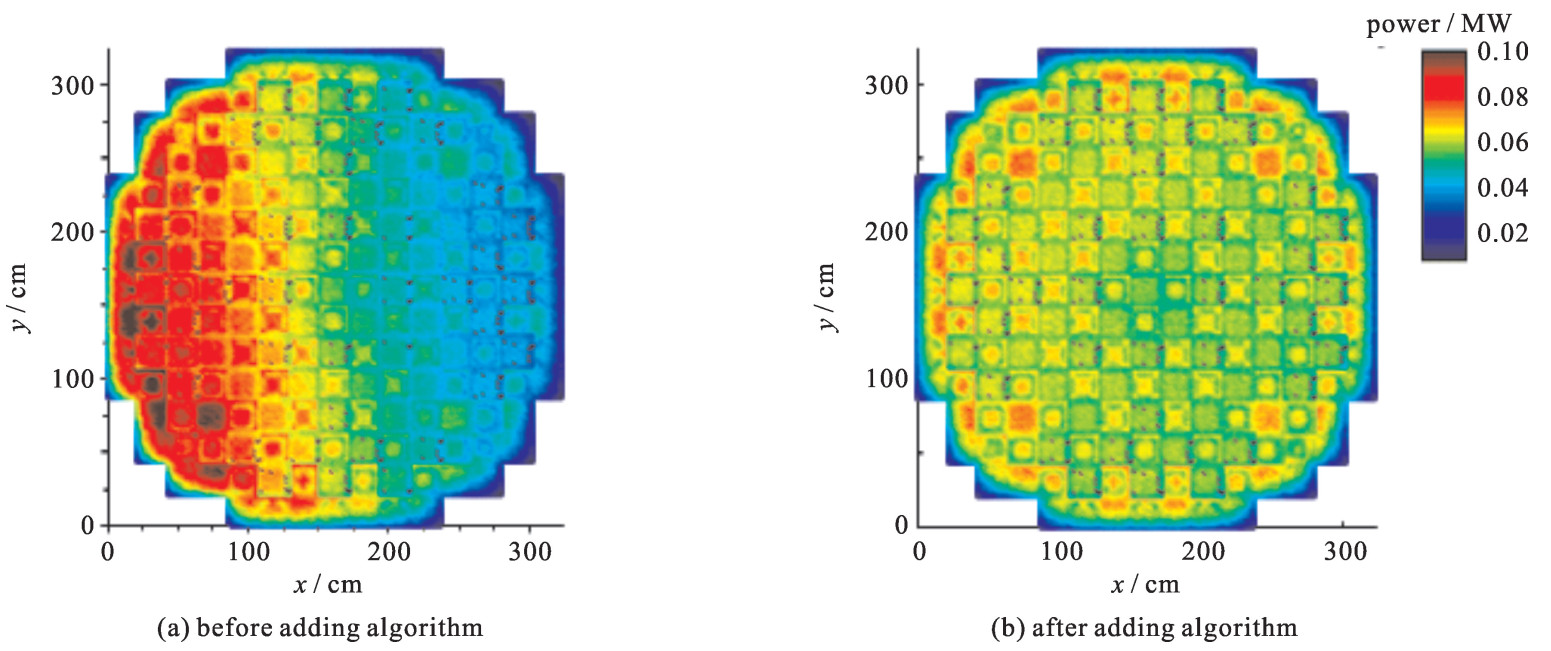
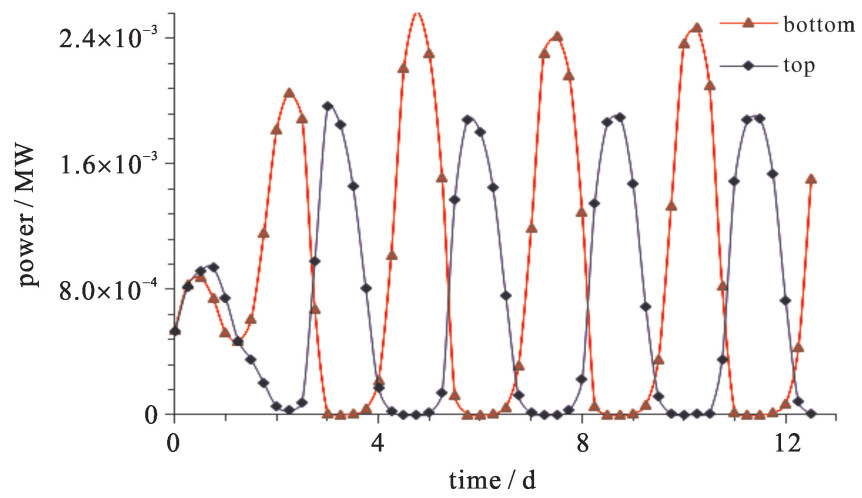
摘要: 根据氙振荡产生的机理，按照燃耗步长来划分：在短步长条件下会产生物理氙振荡，在长步长条件下会产生数值氙振荡。研究发现，当燃耗步长增大到19 h，物理氙振荡的振幅最小，随着燃耗步长继续增大，表现为数值氙振荡，当燃耗步长27 d，数值氙振荡振幅最小。采用平衡氙方法强制使中子通量与氙浓度平衡，在堆芯物理计算程序(RMC)的输运模块中运用平衡氙方法对碘氙的浓度进行不断的迭代更新，在保证收敛的情况下抑制了氙振荡现象的发生。Abstract: Burnup calculation is one of the core contents in physical analysis of nuclear reactors. In Monte Carlo burnup calculation, power oscillation might occur periodically in certain geometrical dimension and certain step length, namely xenon oscillation. Xenon oscillations might result in a serious deviation from the actual situation. The fundamental reason for the xenon oscillation is that the neutron flux and xenon concentration in the regions of burn-up do not match. According to the mechanism of xenon oscillation, we classify xenon oscillations as physical xenon oscillations produced in short step conditions and numerical xenon oscillations produced in long time step conditions. Balanced xenon oscillation suppression method is forced to balance neutron flux with xenon concentration. In the RMC transport the article uses module a balanced xenon method for the calculation of iodine and xenon. In the RMC transport module, the equilibrium xenon method was used to continuously update the concentration of iodine and xenon. In the case of convergence, the occurrence of xenon oscillation is suppressed.
-
图 7 第0燃耗步平衡氙算法添加前后功率分布对比
Figure 7. Burnup step 0, comparison of power distribution before and after add equilibrium xenon algorithm
图 8 第9燃耗步平衡氙算法添加前后功率分布对比
Figure 8. Burnup step 9, comparison of power distribution before and after add equilibrium xenon algorithm
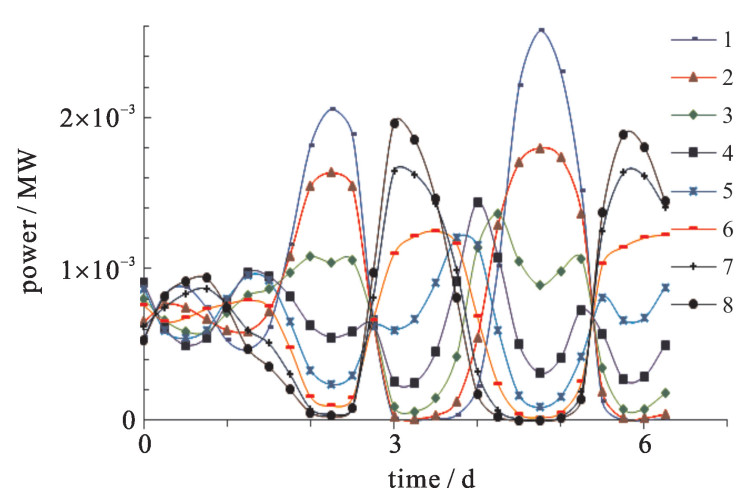
表 1 单棒4 m，8个区，不同时间步长下最大振幅(短步长)
Table 1. 4 m long single rod separated into 8 regions, maximum amplitude of oscillation under different time steps(short time steps)
time step/h maximum amplitude/MW end point of physical xenon oscillations/h 13 0.015 848 14 0.014 940 15 0.010 416 16 0.008 625 17 0.004 345 18~19 18 0.004 380 19 0.003 672 20 0.005 061 21 0.003 367 
下载: 导出CSV
表 2 单棒, 4 m, 8个区，不同时间步长下最大振幅差(长步长)
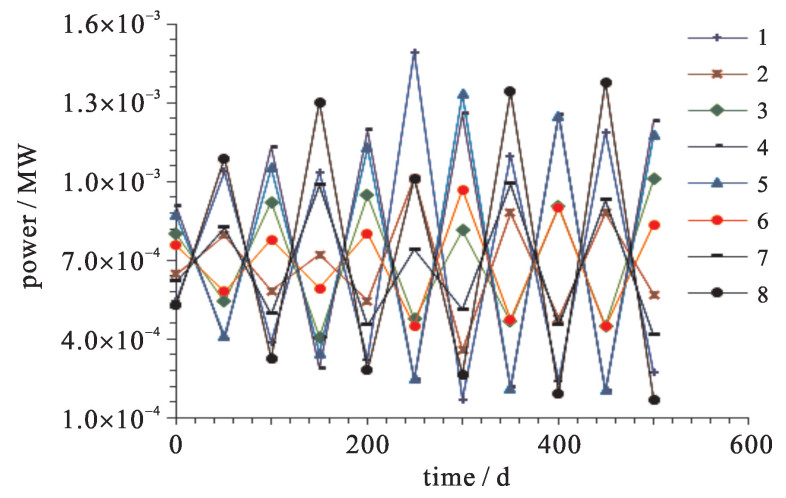
Table 2. 4 m long single rod separated into 8 regions, maximum amplitude of oscillation under different time steps(long time steps)
time step/d maximum amplitude/MW end point of numerical xenon oscillations/d 26 0.002 389 27 0.001 821 28 0.006 336 27~28 29 0.007 038 30 0.007 125 31 0.006 285
下载: 导出CSV
-
[1] 佘顶. 基于自主堆用蒙卡程序RMC的燃耗与源收敛问题研究[D]. 北京: 清华大学, 2013.She Ding. Study on the burn-up and source convergence of RMC based on Monarch program. Beijing: Tsinghua University, 2013 [2] 余纲林, 王侃, 王煜宏. MCBurn—MCNP和ORIGEN耦合程序系统[J]. 原子能科学技术, 2003, 37 (3): 250-254. https://www.cnki.com.cn/Article/CJFDTOTAL-YZJS200303013.htmYu Ganglin, Wang Kan, Wang Yuhong. MCBurn—MCNP and ORIGEN coupling program system. Atomic Energy Science and Technology, 2003, 37 (3): 250-254 https://www.cnki.com.cn/Article/CJFDTOTAL-YZJS200303013.htm [3] Isotalo A, Leppänen J, Dufek J. Preventing xenon oscillations in Monte Carlo burnup calculations by enforcing equilibrium xenon distribution[J]. Annals of Nuclear Energy, 2013, 60 : 78-85. doi: 10.1016/j.anucene.2013.04.031 [4] 张法邦, 吴清泉. 核反应堆运行物理[M]. 北京: 原子能出版社, 2000.Zhang Fabang, Wu Qingquan. Nuclear physics in reactor operation. Beijing: Atomic Energy Press, 2000 [5] Dufek J, Kotlyar D, Shwageraus E, et al. Numerical stability of the predictor-corrector method in Monte Carlo burnup calculations of critical reactors[J]. Annals of Nuclear Energy, 2013, 56 : 34-38. doi: 10.1016/j.anucene.2013.01.018 [6] Dufek J, Kotlyar D, Shwageraus E. The stochastic implicit Euler method—A stable coupling scheme for Monte Carlo burnup calculations[J]. Annals of Nuclear Energy, 2013, 60 : 295-300. doi: 10.1016/j.anucene.2013.05.015 [7] Griesheimer D P. In-line xenon convergence algorithm for Monte Carlo reactor calculations[C]//PHYSOR2010. 2010: 660-679. -
点击查看大图
计量
- 文章访问数: 1313
- HTML全文浏览量: 326
- PDF下载量: 243
- 被引次数: 0
