Stability analysis and improvement of conformal leapfrog alternating direction implicit finite-difference time-domain method
-
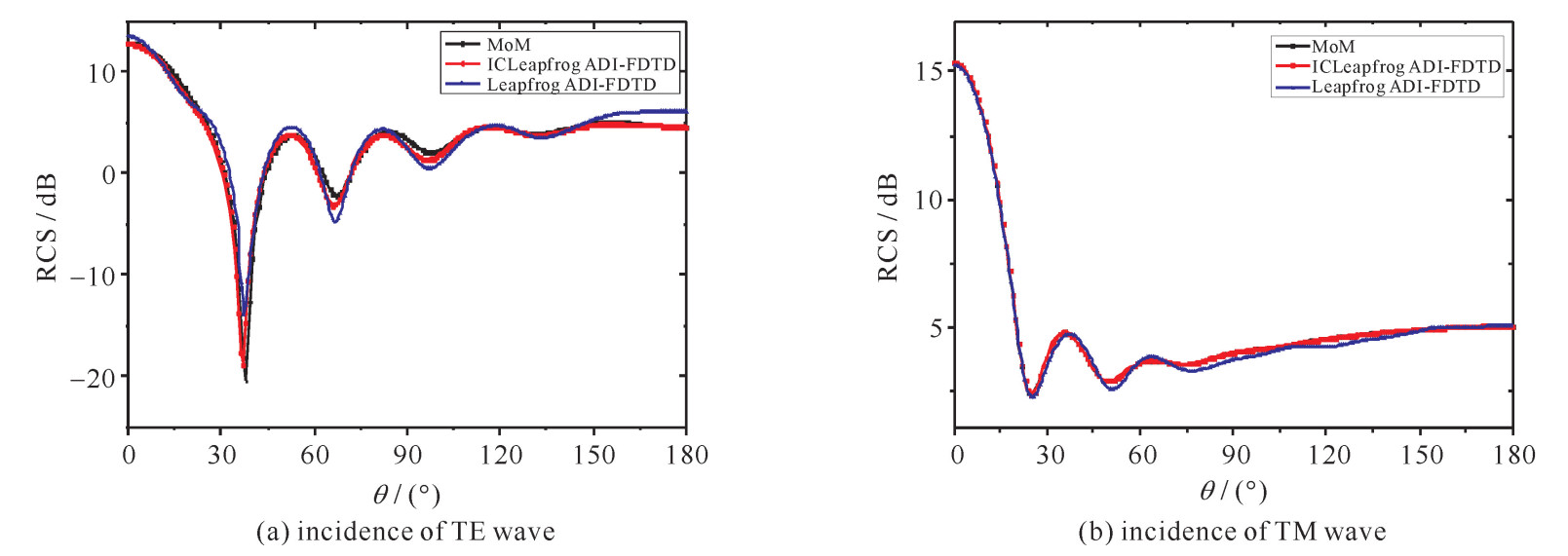
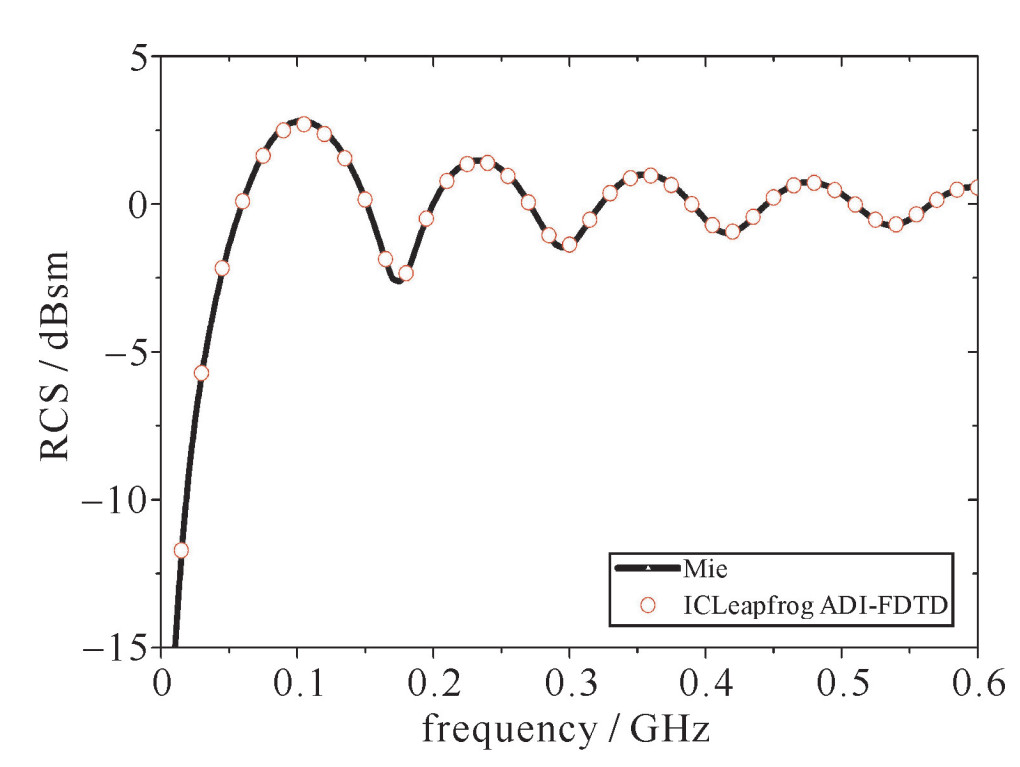
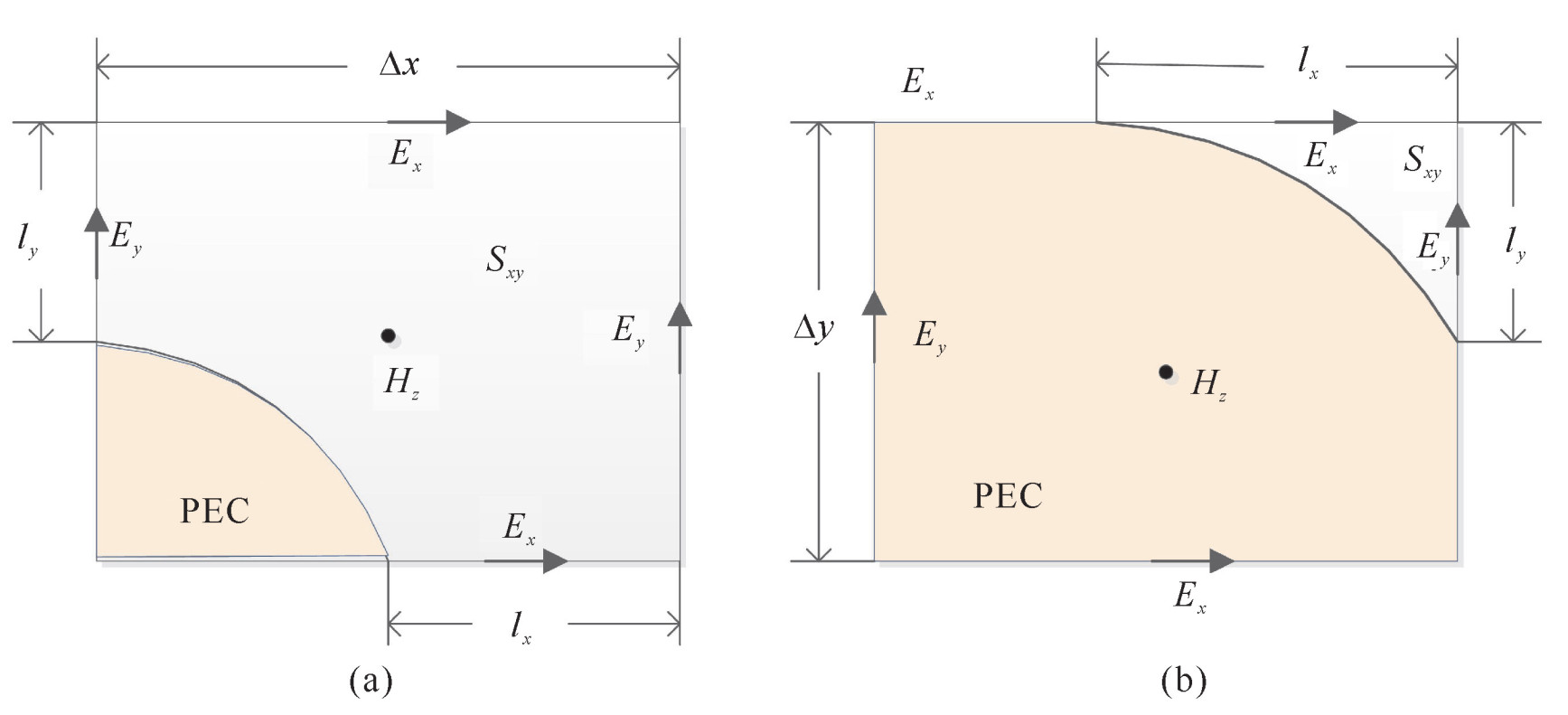
摘要: 提出了一种基于共形网格技术的共形单步交替方向隐式时域有限差分(CLeapfrog ADI-FDTD)方法。与常规FDTD方法相比,此方法能够减小由于目标边界不契合网格划分而引入的阶梯近似误差,提高算法计算不规则目标时的精度;同时算法稳定性更强,计算效率更高。由于引入共形技术后显著降低了原差分法的无条件稳定性,本文利用增长矩阵本征值方法理论分析了算法的稳定性,然后采用了一种改进的共形面积计算方法,在此基础上提出了一种稳定性更高的改进的共形单步交替方向隐式时域有限差分(ICLeapfrog ADI-FDTD)方法。数值算例验证了ICLeapfrog ADI-FDTD是一种具有高稳定性和高精度的高效算法。
-
关键词:
- 共形网格 /
- 时域有限差分 /
- Leapfrog ADI-FDTD /
- 无条件稳定 /
- 稳定性改进
Abstract: A conformal leapfrog alternating direction implicit finite-difference time-domain (CLeapfrog ADI-FDTD) method based on conformal technique was proposed in the article. Compared with the conventional FDTD method, the proposed method decreased the step approximation error, it was used to simulate the irregular object whose boundary couldn't match the orthogonal grid; at the same time, this method could have a high efficiency because leapfrog alternating direction implicit finite-difference time-domain (Leapfrog ADI-FDTD) is a method with unconditional stability. However, CLeapfrog ADI-FDTD method may lose the stability expected with the Leapfrog ADI-FDTD schemes, and instability factor in CLeapfrog ADI-FDTD was analyzed through eigenvalue of the growth matrix, then a new method named improved conformal leapfrog alternating direction implicit finite-difference time-domain (ICLeapfrog ADI-FDTD) with a modified conformal technique was proposed, which could improve the stability without losing the calculation accuracy. The accuracy and efficiency of the proposed ICLeapfrog ADI-FDTD method were verified by numerical results. -
表 1 不同时间步长下共形方法的增长矩阵模的最大值
Table 1. Maximum eigenvalue in the growth matrix at different time step
C CLeapfrog ADI-FDTD ICLeapfrog ADI-FDTD 0.1 1.025 1.065 0.5 1.129 1.371 1.0 1.276 1.909 1.5 1.444 2.717 2.0 1.637 3.903 3.0 2.114 7.551 4.0 2.740 12.700 -
[1] 葛德彪, 闫玉波. 电磁波时域有限差分法[M]. 3版. 西安: 西安电子科技大学出版社, 2011: 1-27.Ge Debiao, Yan Yubo. Finite-difference time-domain method for electromagnetic waves. 3rd ed. Xi'an: Xidian University Press, 2011: 1-27 [2] 白剑. FDTD在若干电磁问题中应用的研究[D]. 西安: 西安电子科技大学, 2007: 1-45.Bai Jian. Research on the applications of FDTD for the analysis of the electromagnetic problems. Xi'an: Xidian University, 2007: 1-45 [3] 刘宗信, 陈亦望, 徐鑫, 等. 三维周期结构弱无条件稳定时域有限差分算法[J]. 强激光与粒子束, 2012, 24(11): 2687-2692. doi: 10.3788/HPLPB20122411.2687Liu Zong-xin, Chen Yiwang, Xu Xin, et al. Conformal FDTD mesh-generating technique for objects with triangle-patch model. High Power Laser and Particle Beams, 2012, 24(11): 2687-2692 doi: 10.3788/HPLPB20122411.2687 [4] Cooke S J, Botton M, Antonsen Jr T M, et al. A leapfrog formulation of the 3-D ADI-FDTD algorithm[J]. International Journal of Numerical Modeling, 2009, 22(2): 187-200. doi: 10.1002/jnm.707 [5] Wang Yigang, Chen Bin, Chen Hailin, et al. One step leapfrog ADI-FDTD method in 3-D cylindrical grids with a CPML implementation[J]. IEEE Antennas and Wireless Propagation Letters, 2014, 13: 714-717. doi: 10.1109/LAWP.2014.2315435 [6] Liang Zhuoxian, Xie Hao, Guo Yang, et al. Improved hybrid leapfrog ADI-FDTD method for simulating near-field coupling effects among multiple thin wire monopole antennas on a complex platform[J]. IEEE Trans Electromagnetic Compatibility, 2017, 59(2): 618-626. doi: 10.1109/TEMC.2016.2632129 [7] 杨利霞, 胡晓娟, 葛德彪. 基于目标三角面元模型生成FDTD共形网格的方法[J]. 强激光与粒子束, 2007, 19(8): 1333-1337. https://www.cnki.com.cn/Article/CJFDTOTAL-QJGY200708024.htmYang Li-xia, Hu Xiaojuan, Ge Debiao. Conformal FDTD mesh-generating technique for objects with triangle-patch model. High Power Laser and Particle Beams, 2007, 19(8): 1333-1337 https://www.cnki.com.cn/Article/CJFDTOTAL-QJGY200708024.htm [8] Dey S, Mittra R, Chebolu S. A locally conformal finite-difference time-domain algorithm for modeling three-dimensional perfectly conduction objects[J]. IEEE Microwave Opt Tech Lett, 1997, 7(9): 273-275. [9] 何页. 共形时域有限差分方法的理论研究及其相关应用[D]. 南京: 南京航空航天大学, 2010: 21-55.He Ye. Theoretical study and related applications of the conformal finite difference time domain. Nanjing: Nanjing University of Aeronautics and Astronautics, 2010: 21-55 [10] Kong Yongdan, Chu Qingxin. Efficient unconditionally stable one step leapfrog ADI-FDTD method with low numerical dispersion[J]. IET microwave, antennas & propagation, 2014, 8(5): 337-345. [11] Dai J, Chen Z, Su D, et al. Stability analysis and improvement of the conformal ADI-FDTD methods[J]. IEEE Trans Antennas and Propagation, 2011, 59(6): 2248-2258. doi: 10.1109/TAP.2011.2143686 -





 下载:
下载: