基于小波变换的拼接重叠区域融合方法

刘丁枭; 盛伟繁

doi:10.11884/HPLPB201830.180020

基于小波变换的拼接重叠区域融合方法

doi: 10.11884/HPLPB201830.180020

刘丁枭^{1, 2,},
盛伟繁^{1, 2, ,}

1.
中国科学院高能物理研究所, 北京 100049
2.
中国科学院大学, 北京 100049

基金项目:

国家自然科学青年基金项目 11005123

详细信息

作者简介:
刘丁枭(1990－), 男，博士研究生，从事光学测量等方面研究；liudx@ihep.ac.cn

通讯作者:
盛伟繁(1962－), 男，研究员，博士生导师，从事同步辐射光学及光束线技术等方面研究；shengwf@ihep.ac.cn

中图分类号: O439
计量
- 文章访问数: 1032
- HTML全文浏览量: 267
- PDF下载量: 117
- 被引次数: 0
出版历程
- 收稿日期: 2018-01-17
- 修回日期: 2018-04-18
- 刊出日期: 2018-08-15

Fusion of the stitching interferometer overlapping areas

Liu Dingxiao^{1, 2
,},
Sheng Weifan^{1, 2
, ,}

1.
Institute of High Energy Physics, Chinese Academy of Sciences, Beijing 100049, China
2.
University of Chinese Academy of Sciences, Beijing 100049, China

摘要

摘要: 为了解决大口径光学元件面形高精度测量问题，建立了拼接测量系统，通过测量得到整体表面面形。在拼接测量过程中，需要将待测面形进行划分，按着一定的顺序进行测量，再根据各个子口径之间的相对位置进行拼接。各个子口径存在重叠部分，采用均化的处理方法会导致高频面形数据的丢失。采用小波变换的拼接重叠区域融合方法可以减少高频数据的丢失。首先，对各个子口径的重叠区域分别进行小波变换得到低频和高频系数矩阵；然后，根据不同的方法对低频和高频系数矩阵进行融合得到新的系数矩阵；最后，通过小波逆变换得到整体面形。对尺寸为120 mm×40 mm的长方形反射镜面形进行拼接干涉测量，并用功率谱密度对本文方法和平均融合结果进行客观比较。实验结果表明，该方法可以保留更多的高频面形数据。
- 拼接干涉仪 /
- 大口径光学元件 /
- 小波融合 /
- 功率谱密度曲线
Abstract: In order to achieve the measurement of large-aperture mirrors with high precision, the stitching interferometer system is established, the whole surface is obtained. The basic theory of stitching measurement technology is to divide the whole test mirror into several parts, and then measure the surface of each part respectively, and finally stitch these surfaces of different parts together. In the process of stitching measurement, overlapping areas between different local surface shapes use mean method of fusing overlapping areas would reduce the high-frequency components. First of all, the original overlapping areas surface shape goes through the wavelet transformation, resulting in low frequency coefficients and high frequency coefficients; Then, low frequency coefficients and high frequency coefficients is determined by different fusion rules; in the end, Finally, overlapping area surface shape is obtained by wavelet inverse transformation. This paper discusses the stitching interferometer test on a 120 mm×40 mm rectangular reflection mirror, in which fusion of overlapping areas has average method as well as the proposed algorithm, and also uses power spectral density conduct an objective comparison on the fusion results. The result of this experiment shows that the proposed algorithm fusion has better effects to improves the retention of high-frequency than the conventional methods.
- stitching interferometer /
- large optical elements /
- wavelet fusion /
- power spectral density curve

HTML全文

图 1 两个相邻局部面形机械定位相对准确

Figure 1. Two adjacent partial surfaces register accurate

下载: 全尺寸图片幻灯片

图 2 两个相邻局部械定位精度不高

Figure 2. Two adjacent partial surfaces register inaccurate

下载: 全尺寸图片幻灯片

图 3 基于小波变换拼接融合过程图

Figure 3. Fusion of stitching interferometer overlapping areas based on wavelet transformation

下载: 全尺寸图片幻灯片

图 4 拼接实验

Figure 4. Stitching measurement

下载: 全尺寸图片幻灯片

图 5 拼接测量路径

Figure 5. Stitching routine of measurement

下载: 全尺寸图片幻灯片

图 6 小波变换方法融合面形结果

Figure 6. Results obtained by using wavelet transformation algorithm

下载: 全尺寸图片幻灯片

图 7 均化方法融合面形结果

Figure 7. Results obtained by using mean algorithm

下载: 全尺寸图片幻灯片

图 8 融合面形结果PSD分析

Figure 8. PSD curve of the surface

下载: 全尺寸图片幻灯片

参考文献(17)

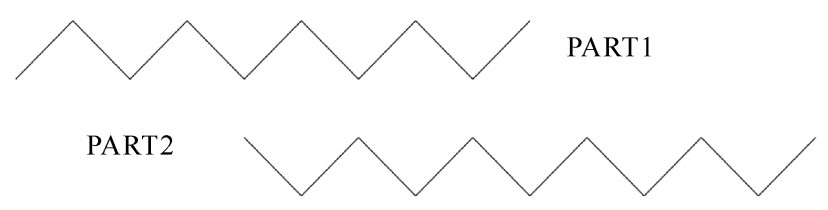
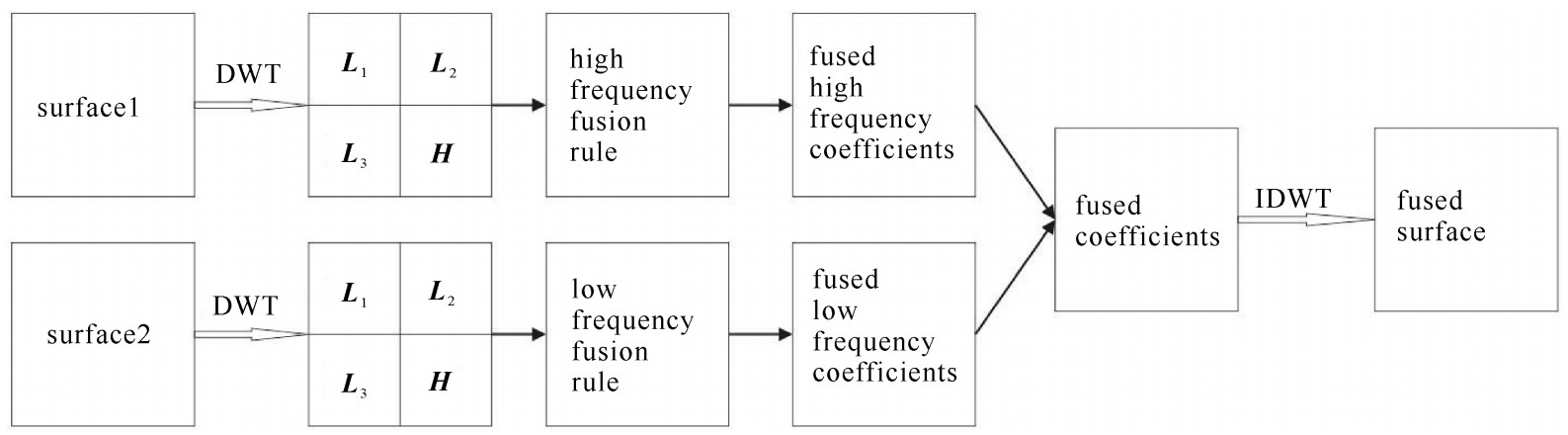
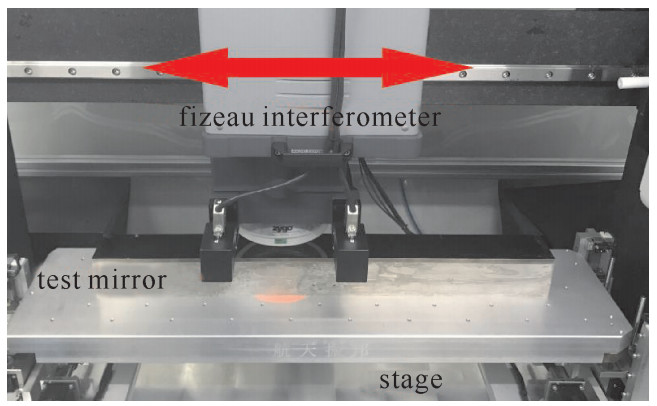
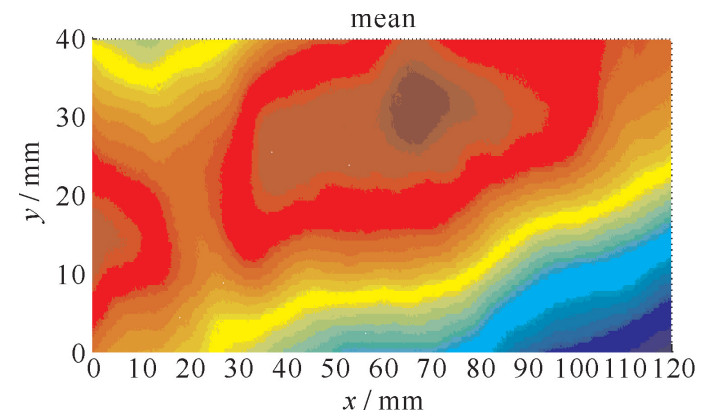
[1]	Catanzaro B, Thomas J A, Cohen E J. Comparison of full-aperture interferometry to sub-aperture stitched interferometry for a large diameter fast mirror[C]//Proc of SPIE. 2001, 4444: 224-237.
[2]	Fleig J, Dumas P, Murphy P E, et al. An automated subaperture stitching interferometer workstation for spherical and aspherical surfaces[C]//Proc of SPIE. 2003, 5188: 296-307.
[3]	Vivo A, Lantelme B, Baker R, et al. Stitching methods at the European Synchrotron Radiation Facility(ESRF)[J]. Review of Scientific Instruments, 2016, 87: 051908. doi: 10.1063/1.4950745
[4]	Fritz B S. Absolute calibration of an optical flat[J]. Optical Engineering, 1984, 23(4): 379-383.
[5]	Kim C J. Polynomial fit of interferograms[J]. Applied Optics, 1982, 21(24): 4521-4525. doi: 10.1364/AO.21.004521
[6]	Otsubo M, Okada K, Tsujiuchi J. Measurement of large plane surface shapes by connecting small-aperture interferograms[J]. Optical Engineering, 1994, 33(2): 608-613. doi: 10.1117/12.152248
[7]	Bray M. Stitching interferometer for large piano optics using a standard interferometer[C]//Proc of SPIE. 1997, 3134: 39-50.
[8]	Bray M. Stitching interferometer for large optics using a standard interferometer. Description of an automated system[C]//Proc of SPIE. 1997, 3047: 911-918.
[9]	Zhao Chunyu, James H B. Stitching of off-axis sub-aperture null measurements of an aspheric surface[C]//Proc of SPIE. 2008: 706316.
[10]	陈一巍. 子孔径拼接检测方法的研究[D]. 长春: 中国科学院长春光学精密机械与物理研究所, 2015: 35-49. Chen Yiwei. Research on subaperture stitching testing method. Changchun: Changchun Institute of Optics, Fine Mechnics and Physics, Chinese Academy of Sciences, 2015: 35-49
[11]	田金文, 苏康, 柳健, 等. 航空影响的自动无缝镶嵌[J]. 华中理工大学学报, 1998, 26(11): 11-13. Tian Jinwen, Su Kang, Liu Jian, et al. Automatic seamales mosaic of aerial image. Journal of Huazhong University of Science & Technology, 1998, 26(11): 11-13
[12]	Pajares G, Cruz J M D L. A wavelet-based image fusion tutorial[J]. Pattern Recognition, 2004, 37(9): 1855-1872. doi: 10.1016/j.patcog.2004.03.010
[13]	Amolins K, Zhang Y, Dare P. Wavelet based image fusion techniques-an introduction, review and comparison[J]. ISPRS Journal of Photogrammetry and Remote Sensing, 2007, 62(4): 249-293. doi: 10.1016/j.isprsjprs.2007.05.009
[14]	曹喆. 一种区域特性的小波图像融合新算法[J]. 计算机工程与应用, 2011, 47(26): 213-215. doi: 10.3778/j.issn.1002-8331.2011.26.060 Cao Zhe. Novel image fusion algorithm based on regional feature with wavelet. Computer Engineering and Applications, 2011, 47(26): 213-215 doi: 10.3778/j.issn.1002-8331.2011.26.060
[15]	Novak E, Ai C, Wyant J C. Transfer function characterization of laser Fizeau interferometer for high spatial frequencv phase measurements[C]//Proc of SPIE. 1997, 3134: 114-121.
[16]	张蓉竹, 许乔, 顾元元, 等. 大口径光学元件检测中的主要误差及其影响[J]. 强激光与粒子束, 2001, 13(2): 133-136. http://www.hplpb.com.cn/article/id/1579 Zhang Rongzhu, Xu Qiao, Gu Yuanyuan, et al. Testing errors and its influence of the large aperture optical elements. High Power Laser and Particle Beams, 2001, 13(2): 133-136 http://www.hplpb.com.cn/article/id/1579
[17]	于瀛洁, 李国培. 关于光学元件波面测量中的功率谱密度[J]. 计量学报, 2003, 24(2): 103-107. https://www.cnki.com.cn/Article/CJFDTOTAL-JLXB200302006.htm Yu Yingjie, Li Guopei. Power spectral density in wavefront measurement of optical components. Acta Metrologica Sinica, 2003, 24(2): 103-107 https://www.cnki.com.cn/Article/CJFDTOTAL-JLXB200302006.htm