Evaluation of electromagnetic shielding effectiveness for metallic enclosure with three-dimensional complex thin slots
-
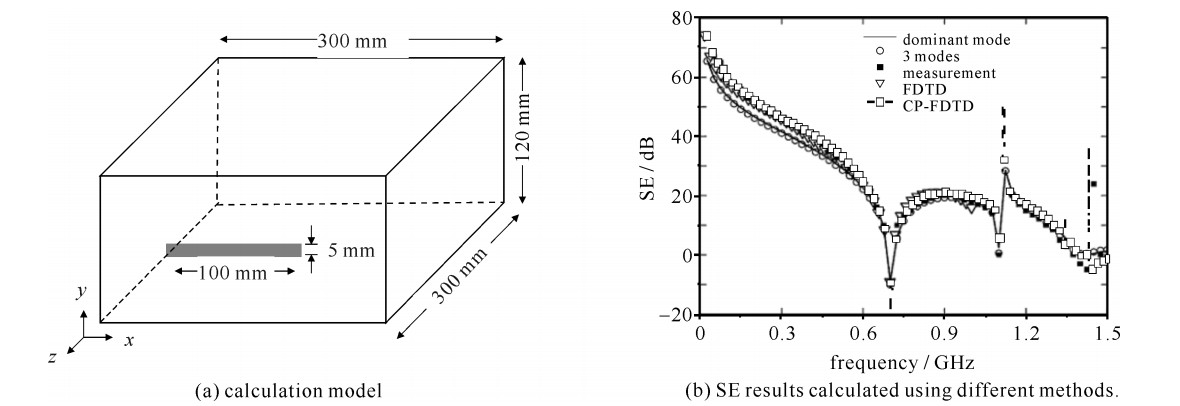
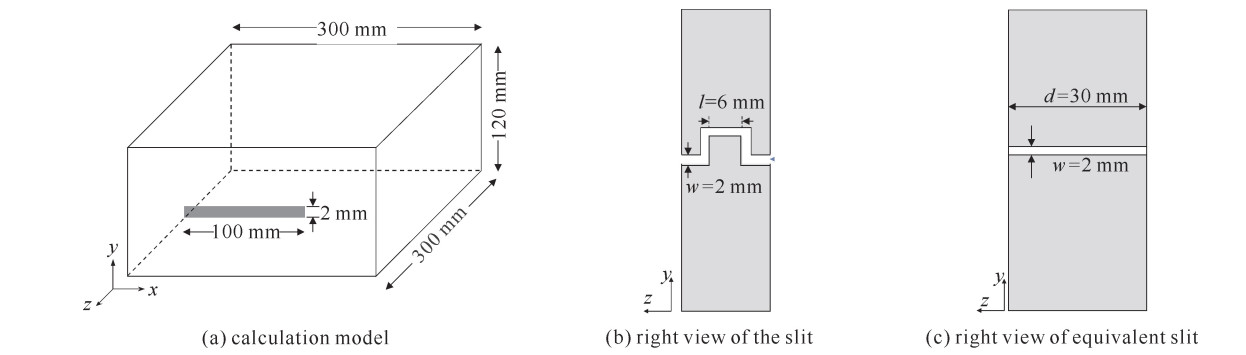
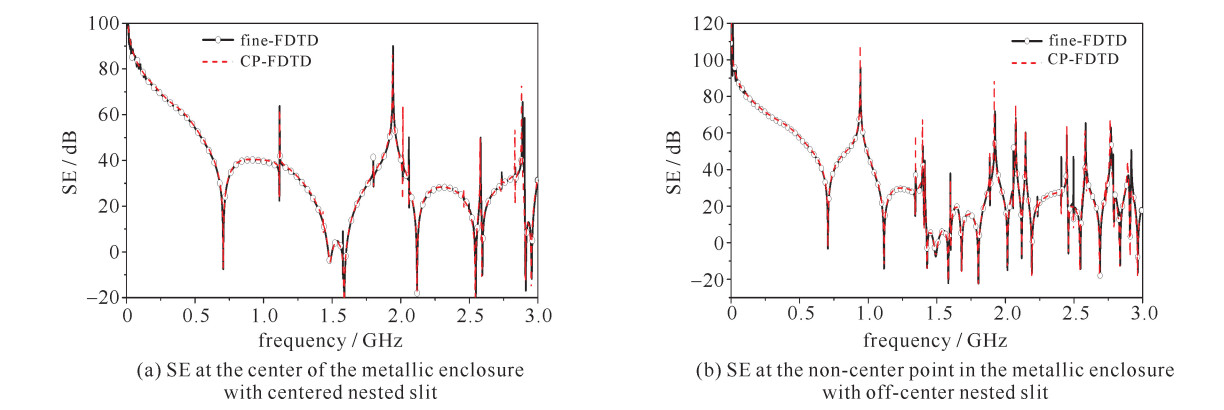
摘要: 复杂工程细缝为金属腔电磁屏蔽效能的评估带来了挑战。采用时域有限差分(FDTD)法结合环路(CP)法,在不改变原有粗网格剖分的基础上,实现了对三维复杂细缝结构金属腔电磁屏蔽效能的快速计算。提出复杂工程细缝的等效模型,提高了复杂细缝结构的CP编程和计算效率,计算结果与采用精细网格剖分的传统FDTD(fine-FDTD)计算结果吻合很好,验证了等效模型的有效性。在此基础上,分析了两种开有典型工程细缝金属腔在0~3 GHz内的电磁屏蔽效能,计算结果与fine-FDTD算法结果吻合很好,且计算效率大大提高。Abstract: Thin slots with complex configurations used in practical engineering make the evaluation of electromagnetic shielding effectiveness(SE) for metallic enclosure increasingly challenging. The contour path (CP) method is adopted to deal with the complex thin slots in order to keep the coarse gridding in FDTD programming. An equivalent model with simple configuration is presented to replace the complex nested slits, and as a result, the programming and calculation efficiency of the CP-FDTD method is improved when dealing with thin slots with complex configurations. The equivalent model and CP-FDTD are used to calculate the SE of metallic enclosure with two typical kinds of thin slot configurations at the wide frequency range respectively. Results are in good agreements with those obtained by using fine-gridded FDTD, and high calculation efficiency is achieved at the same time.
-
表 1 不同算例CP-FDTD与fine-FDTD计算效率对比
Table 1. Comparison of computation efficiency between CP-FDTD and fine-FDTD
model method enclosure cells total cells memory/MB computation time/min nested slit CP-FDTD 9.50×104 5.88×105 40.32 7.00 fine-FDTD 1.14×106 2.92×106 174.41 263.67 covered slot CP-FDTD 8.64×104 5.53×105 38.32 6.91 fine-FDTD 2.17×106 5.06×106 291.60 409.51 -
[1] 刘备, 刘强, 阚勇, 等. 基于TLM的双腔体屏蔽系数快速算法[J]. 强激光与粒子束, 2015, 27: 053202. doi: 10.11884/HPLPB201527.053202Liu Bei, Liu Qiang, Kan Yong, et al. Fast prediction algorithm for shielding effectiveness of double enclosures based on transmission line equivalent circuit method. High Power Laser and Particle Beams, 2015, 27: 053202 doi: 10.11884/HPLPB201527.053202 [2] Konefal T, Dawson J F, Marvin A C, et al. A fast multiple mode intermediate level circuit model for the prediction of shielding effectiveness of a rectangular box containing a rectangular aperture[J]. IEEE Trans Electromagn Compat, 2005, 47(4): 678-691. doi: 10.1109/TEMC.2005.853715 [3] 张亚普, 达新宇, 谢铁城. 孔缝箱体屏蔽效能电磁拓扑分析模型[J]. 强激光与粒子束, 2014, 26: 023204. doi: 10.3788/HPLPB201426.023204Zhang Yapu, Da Xinyu, Xie Tiecheng. Electromagnetic topology model for shielding effectiveness estimation of metallic enclosure with apertures. High Power Laser and Particle Beams, 2014, 26: 023204 doi: 10.3788/HPLPB201426.023204 [4] 阚勇, 闫丽萍, 赵翔, 等. 基于电磁拓扑的多腔体屏蔽效能快速算法[J]. 物理学报, 2016, 65: 030702. doi: 10.7498/aps.65.030702Kan Yong, Yan Liping, Zhao Xiang, et al. Electromagnetic topology based fast algorithm for shielding effectiveness estimation of multiple enclosures with apertures. Acta Physica Sinica, 2016, 65: 030702 doi: 10.7498/aps.65.030702 [5] Dehkhoda P, Tavakoli A, Azadifar M. Shielding effectiveness of an enclosure with finite wall thickness and perforated opposing walls at oblique incidence and arbitrary polarization by GMMoM[J]. IEEE Trans Electromagn Compat, 2012, 54(4): 792-805. doi: 10.1109/TEMC.2012.2188855 [6] Gilbert J, Holland R. Implementation of the thin-slot formalism in the finite-difference EMP Code THREDⅡ[J]. IEEE Trans Nucl Sci, 1981, 28(6): 4269-4274. doi: 10.1109/TNS.1981.4335711 [7] Taflove A, Umashankar K R, Beker B, et al. Detailed FD-TD analysis of electromagnetic fields penetrating narrow slots and lapped joints in thick conducting screens[J]. IEEE Trans Antennas Propagat, 1988, 36(2): 247-257. doi: 10.1109/8.1102 [8] 孙大伟, 俞集辉. 基于环路法的三维细孔缝电磁分析算法[J]. 重庆大学学报, 2006, 29(5): 32-35. https://www.cnki.com.cn/Article/CJFDTOTAL-FIVE200605008.htmSun Dawei, Yu Jihui. A 3D thin slot model for shielding analysis. Journal of Chongqing University, 2006, 29(5): 32-35 https://www.cnki.com.cn/Article/CJFDTOTAL-FIVE200605008.htm [9] Riley D J, Turner C D. Hybrid thin-slot algorithm for the analysis of narrow apertures in finite-difference time-domain calculations[J]. IEEE Trans Antennas Propagat, 1990, 38(12): 1943-1950. doi: 10.1109/8.60983 [10] Brenger J P. A Huygens subgridding for the FDTD method[J]. IEEE Trans Antennas Propagat, 2006, 54(12): 3797-3804. doi: 10.1109/TAP.2006.886519 [11] Xue M F, Yin W Y, Liu Q F, et al. Wideband pulse responses of metallic rectangular multistage cascaded enclosures illuminated by an EMP[J]. IEEE Trans Electromagn Compat, 2008, 50(4): 928-939. doi: 10.1109/TEMC.2008.927943 -






 下载:
下载: