Charged particles acceleration by linearly polarized electromagnetic wave in medium with tapered refractive index
-
摘要: 讨论了线极化波对带电粒子三种加速机制：(1)介质折射率递减但外加磁场保持不变；(2)介质折射率不变但外加磁场递增；(3)介质折射率递减且外加磁场递增。结果显示，在一定的加速距离内，按照机制(3)利用LPEMW加速电子的效率最高。另外，机制(3)可以避免机制(2)中电子在加速过程中回飞的问题，这一点在利用线极化波(LPEMW)加速电子束或带电粒子束时非常重要。Abstract: Acceleration of electrons or charged particles by a linearly polarized electromagnetic wave (LPEMW) propagating in a medium along an external magnetic field is theoretically studied. We have investigated three acceleration cases here: (Ⅰ) the medium's refractive index changes with location but the external magnetic field is constant, (Ⅱ) the medium's refractive index is constant but the external magnetic field increases with the distance in the wave propagation direction, and (Ⅲ) both the medium's refractive index and the external magnetic field change with the distance. The results show that the most efficient (i.e., at a fixed acceleration distance, obtaining the highest relativistic factor) electron acceleration by the LPEMW can be fulfilled in case (Ⅲ). Besides, in case (Ⅲ), the drawback (such as charged particles' going backward for a while) in the acceleration of case (Ⅱ) can be avoided or greatly reduced, which is very important for acceleration of a bunch of electrons or charged particles by LPEMW. It is believed that the acceleration mechanism found in case (Ⅲ) can be helpful in the design of a compact and efficient chargedparticle accelerator.
-
图 1 在固定外磁场中，对于不同的α值，相对论因子γ关于z的变化曲线
Figure 1. Relativistic factor γ as a function of z for different α values in the case of a fixed external magnetic field
图 2 在均匀介质中，不同的β值时相对论因子γ与距离z的变化曲线
Figure 2. Relativistic factor γ as a function of z for different β values in the homogeneous refractive medium
图 3 在均匀介质中，不同的β值时电子运动距离z与时间t的函数关系
Figure 3. Electron travel distance in z direction as a function of time t for different β values in case of a constant refractive index
图 4 三种加速机制下的线极化波对电子加速的相对论因子γ与距离z的函数关系
Figure 4. Relativistic factor γ as a function of z for electron acceleration by the linearly polarized EMW for three cases: VRIS, VEMFS and their combination (i.e., VRIEMFS)
图 5 在变化的介质中，沿增大的外加磁场方向传播的线极化波对带电粒子加速的粒子在z=0.1 m处相对论因子γ与B0的关系
Figure 5. Values of γ reached at z=0.1 m as a function of B0 for charged particles acceleration by the linearly polarized EMW along an increasing external magnetic field in a medium with a tapered refractive index
图 6 三种机制下粒子回旋半径R与距离z的关系
Figure 6. Cyclotron radius R=(x2+y2)1/2 as a function of z for the three acceleration mechanisms
-
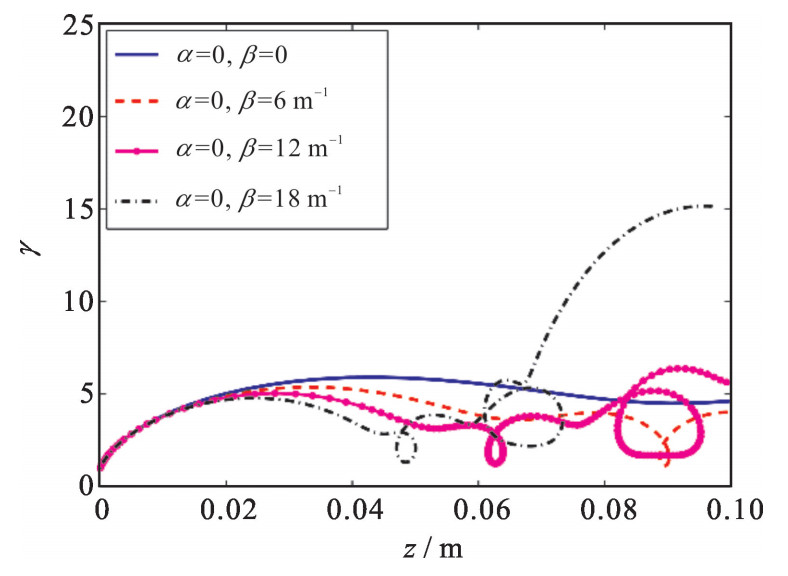
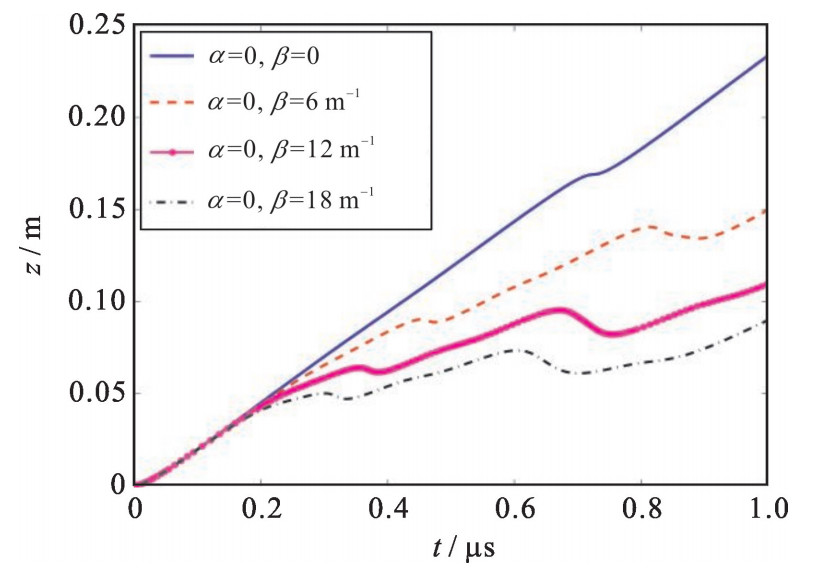
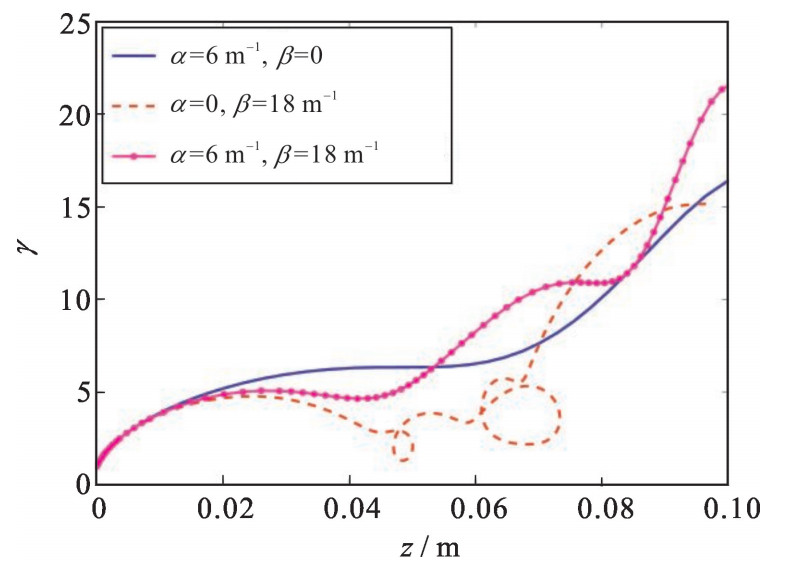
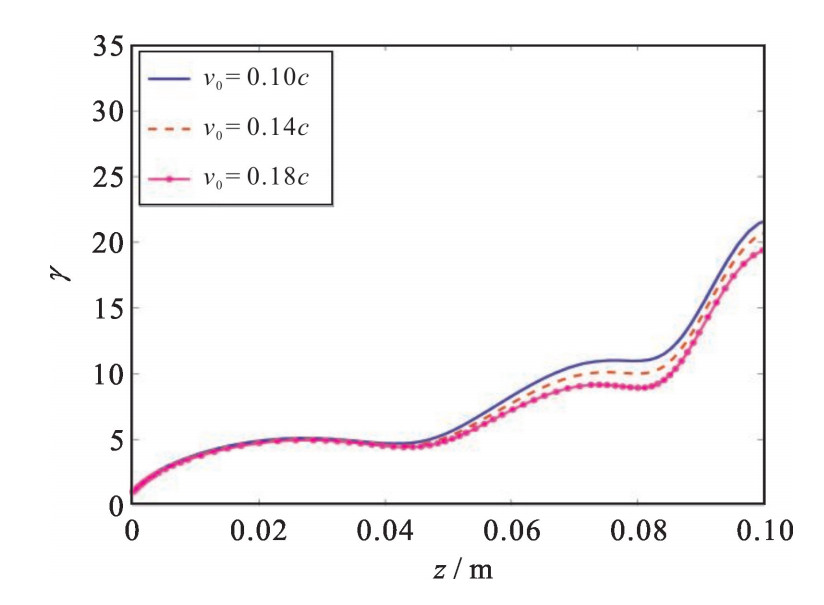
[1] White M G, Isaila M, Prelec K, et al. Acceleration of nitrogen ions to 7.4 GeV in the Princeton Particle Accelerator[J]. Science, 1971, 174: 1121-1123. doi: 10.1126/science.174.4014.1121 [2] Hoffmann D H H, Blazevic A, Rosmej O N, et al. Particle accelerator physics and technology for high energy density physics research[J]. European Physical Journal D, 2007, 44(2): 293-300. doi: 10.1140/epjd/e2006-00125-0 [3] Murphy C D, Trines R, Vieira J, et al. Evidence of photon acceleration by laser wake fields[J]. Physics of Plasmas, 2006, 13: 033108. doi: 10.1063/1.2178650 [4] Suk H. Electron acceleration based on selftrapping by plasma wake fields[J]. Journal of Applied Physics, 2002, 91(1): 487-491. doi: 10.1063/1.1423392 [5] Sullivan D J. Simulation of preaccelerated particles in the plasma beatwave accelerator[J]. IEEE Trans Nuclear Science, 1983, 30(4): 3159-3161. doi: 10.1109/TNS.1983.4336600 [6] Banna S, Mizrahi A, Schächter L. PASER-particle acceleration by stimulated emission of radiation: theory, experiment, and future applications[J]. Laser & Photonics Reviews, 2010, 3(1/2): 97-122. [7] Shimoda K. Proposal for an electron accelerator using an optical maser[J]. Applied Optics, 1962, 1(1): 33-35. doi: 10.1364/AO.1.000033 [8] Flanz J. Accelerators for charged particle therapy[J]. Modern Physics Letters A, 2015, 30: 1540020. doi: 10.1142/S0217732315400209 [9] Hatori S, Kurita T, Hayashi Y, et al. Developments and applications of accelerator system at the Wakasa Wan Energy Research Center[J]. Nuclear Instruments & Methods in Physics Research, 2005, 241(1): 862-869. [10] Tenneti S, Garg R, Hrenya C M, et al. Direct numerical simulation of gas-solid suspensions at moderate Reynolds number: Quantifying the coupling between hydrodynamic forces and particle velocity fluctuations[J]. Powder Technology, 2010, 203(1): 57-69. doi: 10.1016/j.powtec.2010.03.042 [11] Kong L B, Wang H Y, Hou Z L, et al. The self-consistent nonlinear theory of charged particle beam acceleration by slowed circularly polarized electromagnetic waves[J]. Plasma Science and Technology, 2013, 15(12): 1174-1177. doi: 10.1088/1009-0630/15/12/02 [12] Kong L B, Hou Z L, Xie C R. The self-consistent nonlinear theory of electron cyclotron maser based on anomalous Doppler effect[J]. Applied Physics Letters, 2011, 98: 261502. doi: 10.1063/1.3604016 -
下载:
点击查看大图
计量
- 文章访问数: 974
- HTML全文浏览量: 279
- PDF下载量: 88
- 被引次数: 0
