Compressed strong magnetic field confinement effect on alpha particle energy in field-reversed configuration plasma target
-
摘要: 基于一维弹塑性磁流体力学程序（SSS-MHD），研究了反场构型（FRC）等离子体靶在磁驱动固体套筒压缩过程中强磁场对α粒子能量约束效应，分析了α粒子的非局域和局域自加热对FRC等离子靶压缩峰值温度的影响，以及α粒子能量在整个压缩过程中端部损失效应。等离子体部分采用多温单流体的模型，能量的计算中引入了DT离子、电子及α粒子多成分温度的能量方程，同时考虑了等离子体压缩过程热平衡下的核反应和非局域自加热问题。研究结果表明，磁化靶聚变等离子体在压缩过程中具有较好的稳定性，能够保持刚性转子的靶结构，压缩过程形成的强磁场能够将α粒子的能量约束在O点附近的区域，有利于等离子体靶的点火及燃烧；α粒子对等离子体的自加热效应主要集中在等离子体电流中心区，而非等离子体中心轴处；α粒子对DT等离子体局域和非局域自加热过程存在差异，局域自加热过程的功率大于非局域自加热过程的功率，FRC等离子靶压缩峰值状态温度相差0.5倍。在反场构型的刮离层区，α粒子的能量端部损失在FRC等离子体靶的压缩和膨胀过程中逐渐增大。Abstract: Based on an one dimensional elastic-plastic reactive hydro-dynamic code SSS-MHD, confinement effect, by strong magnetic field during compression of field-reversed configuration (FRC) plasma target by solid liner, on the alpha particle energy transport is studied numerically. Also, investigations on the alpha particles self-heating (including local and non-local) and end loss effects are carried out. In the physical model, plasma energy is divided into three parts as that of DT ions, electrons, and alpha particles. In addition, fusion reaction in thermal equilibrium is taken into account. Numerical results imply that FRC target behaves like rigid rotor during solid liner compression. The compressed strong magnetic field can well define alpha particle energy in O-point area in target center rather than in the axis area, which is helpful for an FRC plasma burning. The non-local self-heating power peak value locates at O-point, but the local self-heating power maximum is beyond O-point. The plasma temperature peak value of local self-heating is about 0.5 times greater than that of non-local self-heating. In the script-off layer (SOL), end loss effect of alpha particle energy increases with the solid liner convergence. Especially in the SOL boundary, peak alpha particle energy loss rate appears.
-
Key words:
- imploding of solid liner /
- compressed magnetic field /
- alpha particles /
- end effect
-
图 1 FRC等离子体初始状态下密度、温度及磁场的分布
Figure 1. The initial profile of plasma density, temperature and magnetic field
图 2 脉冲电流波形及固体套筒内外半径随时间的变化
Figure 2. Pulse current and inner and outer radius of the solid liner as a function of time
图 3 等离子体内部（R1＝0.8 mm，R2＝8 mm，R3＝16 mm）及边界磁场压缩历程
Figure 3. Compressed magnetic field both at boundary and internal plasma(R1＝0.8 mm, R2＝8 mm, R3＝16 mm) as a function of time.
图 6 聚变能沉积功率密度、辐射损失功率密度及热传导损失功率密度分布
Figure 6. Distributions of fusion energy deposited to plasma, thermal conduction and radiation
图 7 α粒子非局域自加热电子和离子功率密度曲线
Figure 7. Non-local heating power of alpha particles to ions and electrons
图 8 α粒子局域自加热等离子体功率密度曲线
Figure 8. Local heating power of alpha particles to ions and electrons
图 9 刮离层不同位置处的能量损失率
Figure 9. Loss rate in SOL (initial position：R1=2.51 cm， R2=2.54 cm，R3=2.59 cm)
图 10 刮离层平行于磁场方向的热传导系数及分界面长度的变化
Figure 10. Parallel conductivity coefficient in SOL and separatrix length (initial position：R3=2.59 cm)
-
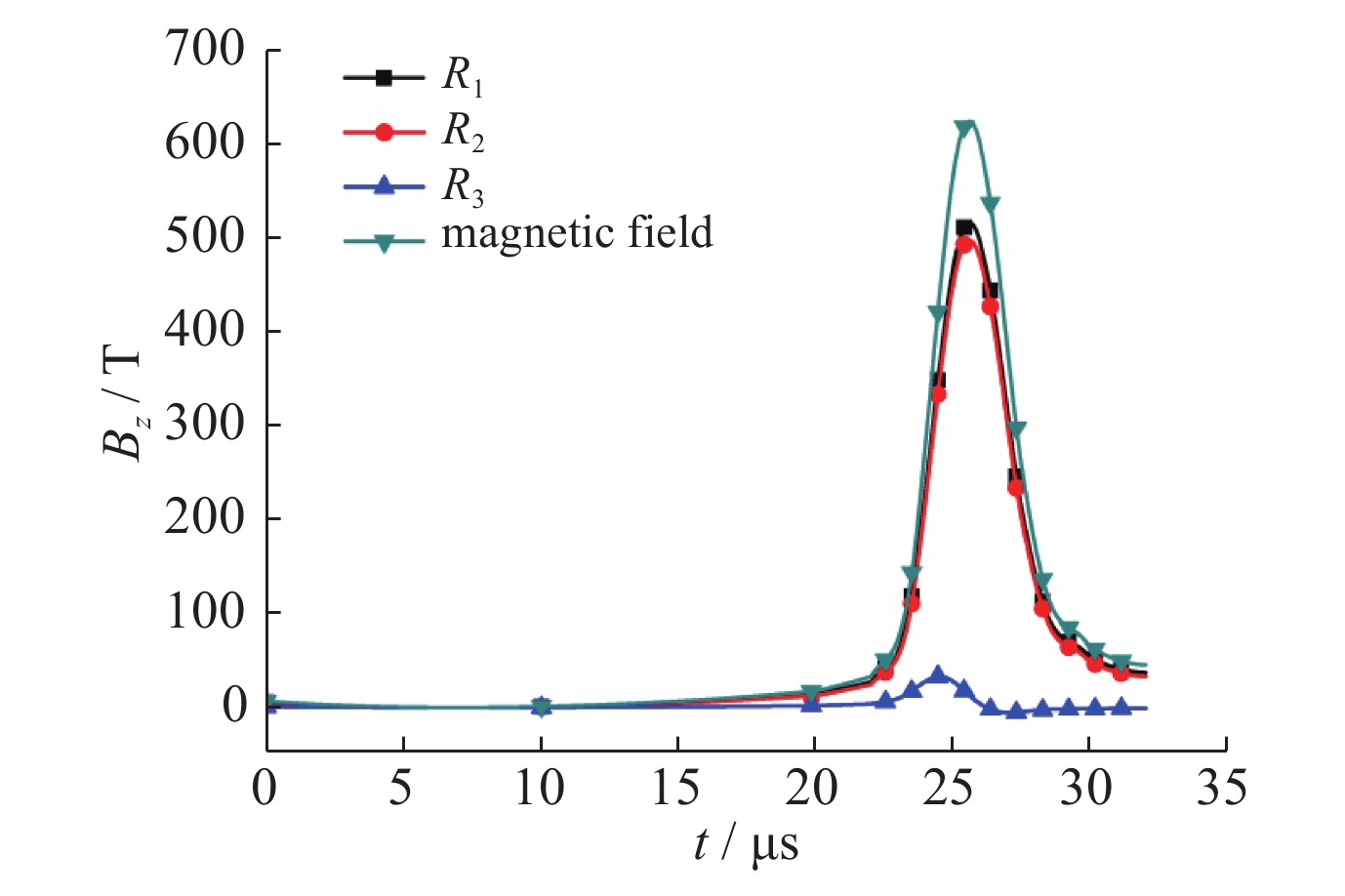
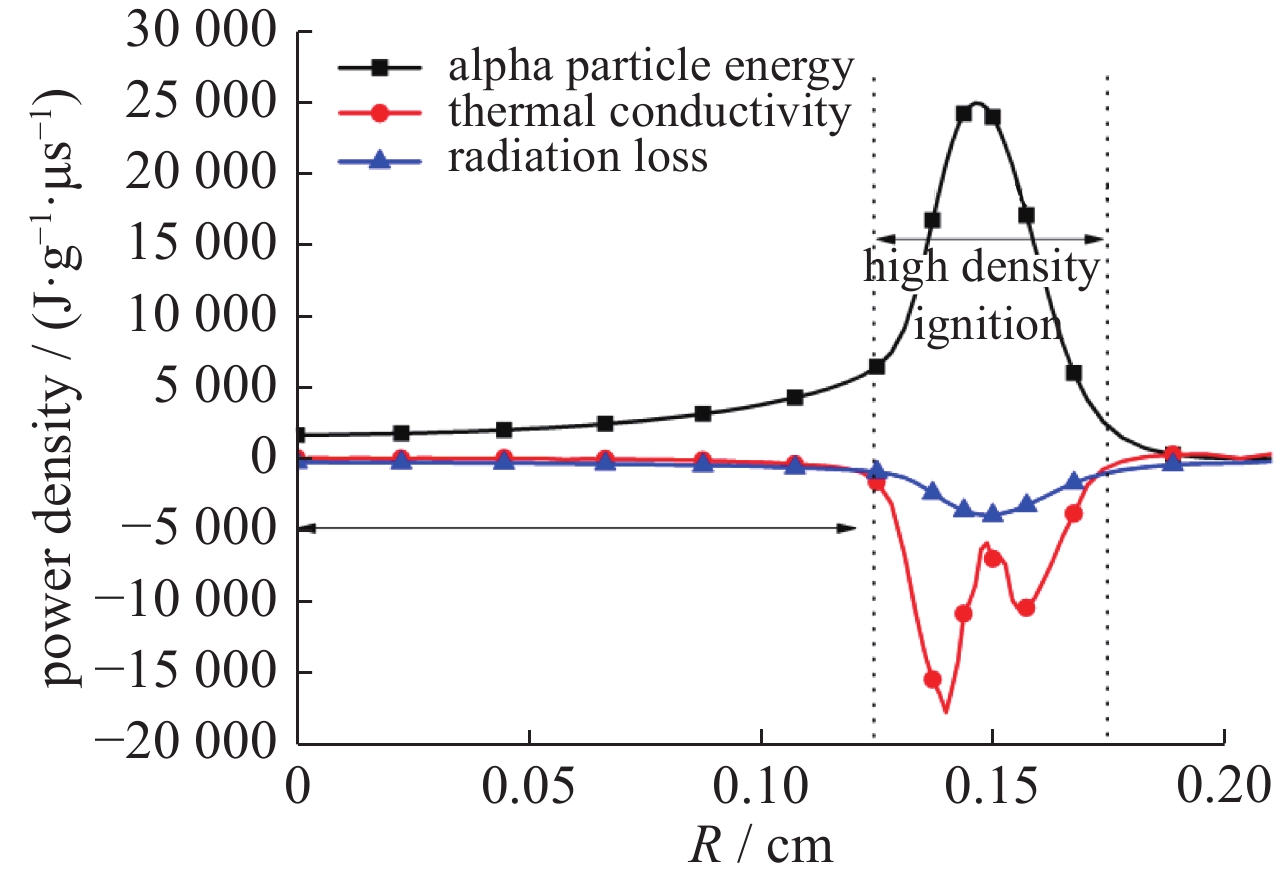
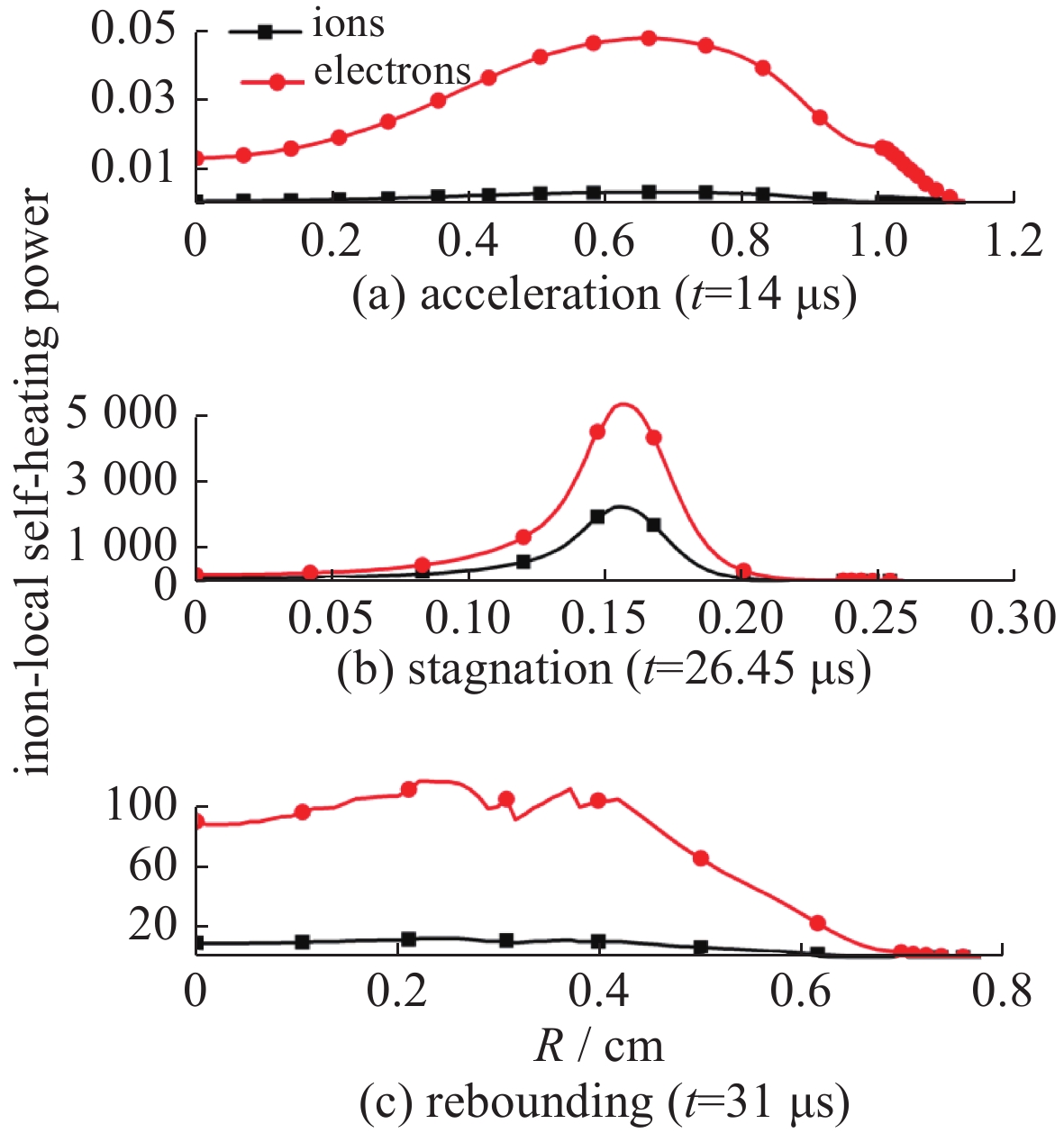
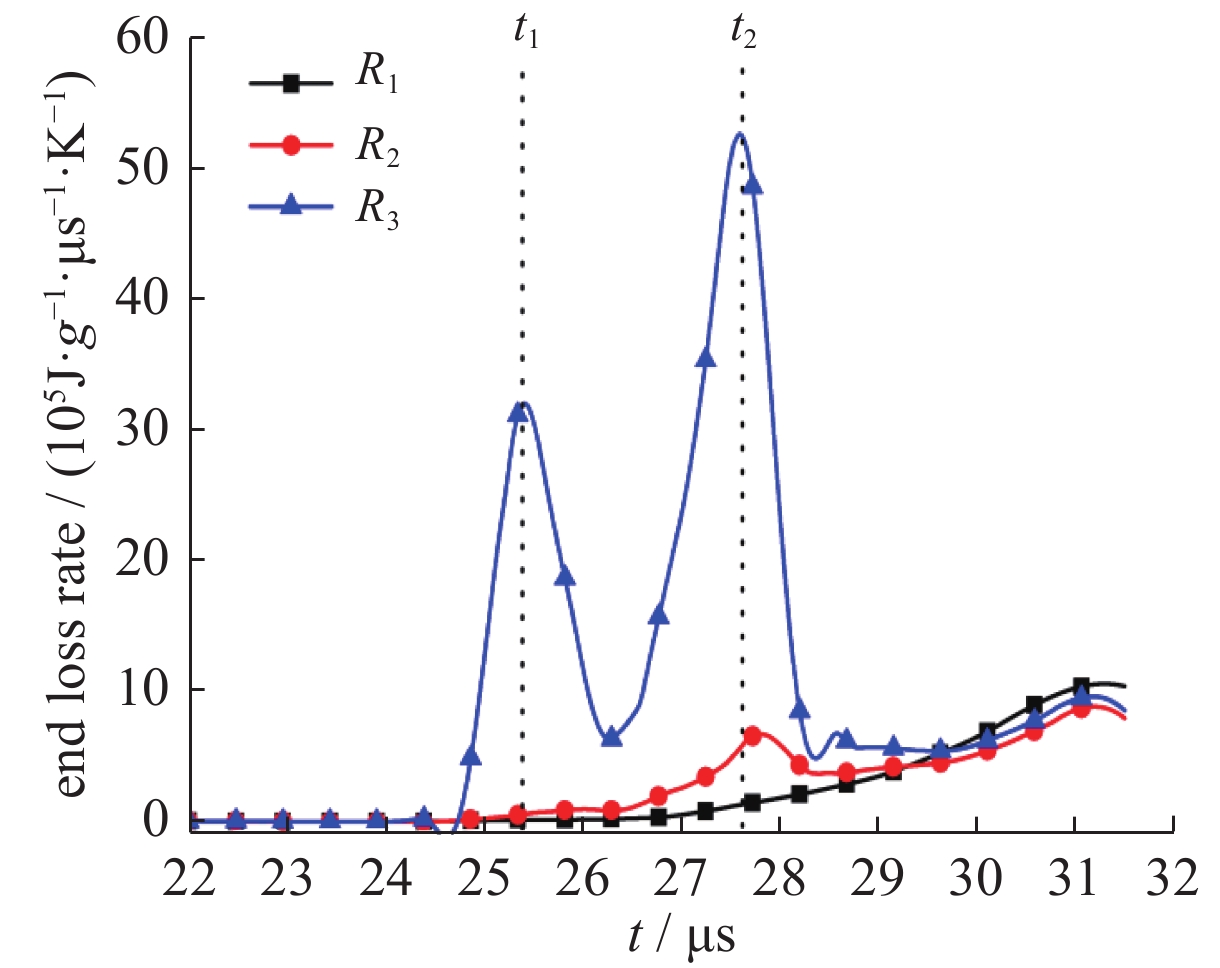
[1] Tuszewski M. Field reversed configurations[J]. Nucl Fusion, 1988, 28(11): 2033-2092. doi: 10.1088/0029-5515/28/11/008 [2] Steinhauer L C. Review of field-reversed configurations[J]. Phys Plasmas, 2011, 18: 070501. doi: 10.1063/1.3613680 [3] Degnan J H, Amdahl D J, Domonkos M, et al. Recent magneto-inertial fusion experiments on the field reversed configuration heating experiment[J]. Nucl Fusion, 2013, 53: 093003. doi: 10.1088/0029-5515/53/9/093003 [4] Degnan J H, Amdahl D J, Brown A, et al. Experimental and computational progress on liner implosions for compression of FRCs[J]. IEEE Trans Plasma Sci, 2008, 36(1): 80-91. doi: 10.1109/TPS.2007.913814 [5] Intrator T, Zhang S Y, Degnan J H, et al. A high density field reversed configuration (FRC) target for magnetized target fusion: First internal profile measurements of a high density FRC[J]. Phys Plasmas, 2004, 11(5): 2580-2585. doi: 10.1063/1.1689666 [6] Sun Q Z, Jia Y S, Yang X J, et al. Formation of field-reversed-configuration (FRC) on the Yingguang-I device[J]. Matter and Radiation at Extremes, 2017, 2(5): 263-274. doi: 10.1016/j.mre.2017.07.003 [7] 孙奇志, 方东凡, 刘伟, 等. " 荧光-1”实验装置物理设计[J]. 物理学报, 2013, 62:078407. (Sun Qizhi, Fang Dongfan, Liu Wei, et al. Physical design of " Yingguang-1” device. Acta Physica Sinica, 2013, 62: 078407 [8] Wang X G, Wang G Q, Liu B, et al. Modeling for compression of field-reversed configurations by an imploding liner[J]. Phys Plasmas, 2016, 23: 112706. doi: 10.1063/1.4968238 [9] Spencer R L, Tuszewski M, Linford R K. Adiabatic compression of elongated field-reversed configurations[J]. Phys Fluids, 1983, 26(6): 1564-1573. doi: 10.1063/1.864334 [10] Yoshimura S, Sugimoto S, Ohi S, et al. Electron cyclotron current drive in a lower hybrid current drive plasma[J]. Nucl Fusion, 1999, 39(4): 2009-2014. [11] Zhang S Y. MHD instability of field-reversed configuration[J]. IEEE Trans Plasma Sci, 2006, 34(13): 223-229. [12] Lindemuth I R, Kirkpatrick R C. Parameter space for magnetized fuel targets in inertial confinement fusion[J]. Nucl Fusion, 1983, 23(3): 263-284. doi: 10.1088/0029-5515/23/3/001 [13] Lindemuth I R. The ignition design space of magnetized target fusion[J]. Phys Plasmas, 2015, 22: 122712. doi: 10.1063/1.4937371 [14] 赵小明, 孙奇志, 贾月松. 球形及柱形磁化等离子体靶中α粒子能量沉积率分析[J]. 强激光与粒子束, 2014, 26:035002. (Zhao Xiaoming, Sun Qizhi, Jia Yuesong. Energy deposition of alpha particles in cylindrical and spherical magnetized plasma target. High Power Laser and Particle Beams, 2014, 26: 035002 [15] Basko M M, Kemp A J, Meyer-ter-Vehn J. Ignition conditions for magnetized target fusion in cylindrical geometry[J]. Fusion, 2000, 40(1): 59-68. doi: 10.1088/0029-5515/40/1/305 [16] 赵继波, 孙承纬, 谷卓伟, 等. 爆轰驱动固体套筒压缩磁场计算及准等熵过程分析[J]. 物理学报, 2015, 64:080701. (Zhao Jibo, Sun Chengwei, Gu Zhuowei, et al. Magneto-hydrodynamic calculation of magnetic flux compression with explosion driven solid liners and analysis of quasi-isentropic process. Acta Physica Sinica, 2015, 64: 080701 doi: 10.7498/aps.64.080701 [17] Zhao J B, Sun C W, Luo B Q, et al. Loading circuit coupled magnetohydrodynamic simulation of sample configurations in isentropic compression experiments[J]. IEEE Tans Plasma Sci, 2015, 43(1): 1068-1077. [18] Zhao X M, Sun Q Z, Sun C W, et al. Simulation on the compressed field reversed configuration with alpha particle self-heating[J]. Plasma Phys Control Fusion, 2019, 61: 075015. doi: 10.1088/1361-6587/ab1e84 [19] Linerman M A, Velikovich A L. Distribution function and diffusion of alpha particles in DT fusion plasma[J]. J Plasma Phys, 1984, 31(3): 369-380. doi: 10.1017/S0022377800001719 [20] Braginskii S I. Transport processes in a plasma; in Reviews of PlasmaPhysics[M]. New York: Springer, 1965: 205-316. [21] Nozachi K, Nishihara K. Thermonuclear reaction wave in high density plasma[J]. J Phys Soc Japan, 1977, 43(4): 1393-1399. doi: 10.1143/JPSJ.43.1393 [22] 赵小明, 孙奇志, 方东凡, 等. 反场构型等离子体中Grad-Shafranov方程数值解[J]. 物理学报, 2016, 65:185201. (Zhao Xiaoming, Sun Qizhi, Fang Dongfan, et al. Numerical solution of Grad-Shafranov equation in FRC plasma. Acta Physica Sinica, 2016, 65: 185201 doi: 10.7498/aps.65.185201 [23] Li C G, Yang X J. Modeling and numerical analysis of a magneto-inertial fusion concept with the target created through FRC merging[J]. Phys Plasmas, 2016, 23: 102702. doi: 10.1063/1.4964367 -
下载:
点击查看大图
计量
- 文章访问数: 1446
- HTML全文浏览量: 404
- PDF下载量: 43
- 被引次数: 0
