Research on influence of cutting parameters on frequency characteristics of KDP surface topography
-
摘要: 针对采用单点金刚石超精加工的KDP晶体光学表面，研究了切削参数对微观形貌频率特征的影响。通过功率谱密度获得表面轮廓频率分布，并用连续小波重构加工过程中随切削用量变化的微观轮廓频率特征。结果表明：切削参数对微观形貌的影响具体表现在实际频率特征上,中频特征波长及幅值反映了切深及转速变化，随切深及转速增加，幅值变大；低频特征反映了进给量变化，随着进给量变小，频率及幅值变小；高频特征是加工过程中振动及材料各向异性的具体表现。
-
关键词:
- 频率特征 /
- 表面形貌 /
- 切削参数 /
- 功率谱密度（PSD） /
- 连续小波变换（CWT）
Abstract: The influence of cutting parameters on the frequency characteristics of optical crystal surface has been studied. The experiment of single point diamond turning was adopted to process KDP crystal. The frequency distribution of surface profile was obtained by power spectral density. The continuous wavelet method was used to evaluate the influence of cutting depth, feed rate and spindle speed on machined surface. The results show that the frequency characteristics reflect the effects of cutting parameters on surface topography. The wavelength and amplitude of mid frequency embody the change of cutting depth and spindle speed, and the amplitude increases with the increase of cutting depth and speed. Low frequency reflects the change of feed rate, and the amplitude becomes small as feed rate decreases. High frequency is the manifestation of vibration and material anisotropy in processing. Therefore the analysis of frequency characteristics on surface topography provides a reference for selecting the optimal process parameters. -
光学晶体加工中工艺参数的选择会直接影响工件表面质量和使用性能。如惯性约束聚变过程中对光学元件可靠性要求极为严格[1]。晶体的高硬脆性及强各向异性等一系列不利因素使加工高质量表面变得困难[2-4]。目前，对晶体材料精密加工而言，单点金刚石超精加工[5-6]相比于磁流变光整加工[7-9]和磨料切割工艺[10]两种常用加工方式，能有效缩短加工周期，表面一致性较好。其过程参数如切削用量、主轴振动、导轨误差、刀具形状、刀具磨损以及切削力的波动将直接影响表面质量。已加工表面微观形貌可看作是加工过程中各参数综合作用的集合，切削用量对微观形貌影响最为明显[11]。本文根据切削用量影响晶体表面微观形貌的频率特征进行分析，获得了切削参数（进给量、切削深度和主轴转速）与实际频率特征关系。
表面形貌极大程度影响着材料使用功能，而切削用量是影响表面形貌的主要因素。国内外学者已开展机加工过程中切削用量对晶体表面质量及其功能基础性研究。Chen N用自行研制的微型PCD球头铣刀对KDP材料进行加工，获得铣削过程中最优参数并验证了微型PCD球头铣刀对表面微缺陷具有修复能力[12-13]。Zheng W研究SiCp/AI复合材料磨削过程中工艺参数对表面形貌影响，综合分析了进给量、主轴转速、主轴振动及切削深度对表面粗糙度不同影响程度[14]。Wang S对KDP晶体材料进行切削实验，获得进给量对KDP单晶表面质量和切削力的关系并分析了材料韧脆性变化[15]。Tian F发现金刚石刀具车削过程中工件振动对表面形貌有很大影响，建立振动对加工表面影响的理论模型并分析工件和刀具在切削方向上的振动关系[16]。Wang X考虑到加工参数及振动对研磨材料的影响并建立研磨运动模型，发现了磨削表面形貌主要受研磨过程中研磨速度影响[17]。Krolczyk G M对表面形貌参数进行差异化描述，包括表面振幅和粗糙度等参数进行评估[18]。Zhang Q用AISI H13钢球头刀具进行铣削实验，分析了进给量和切削深度对已加工表面形貌的影响关系[19]。Wei W研究了加工参数在高速铣削过程中对铣削力的影响规律[20-21]。但上述研究均未考虑表面形貌是实际频率特征无序叠加的组合体，对微观形貌分析仍停留在表面轮廓纹理分布上。为获得加工过程中切削用量对微观形貌影响规律，本文进行单点金刚石车削KDP单晶实验。PSD结合连续小波方法，进行已加工表面轮廓频率特征的提取，研究切削参数对频率特征的影响。
1. 方法与实验
1.1 PSD计算方法
功率谱密度（PSD）通常用来分析输入信号的空间频率信息。PSD不仅包含表面垂直方向的高度信息，同时包含重要的横向空间频率分布[22]。表面微观轮廓频率信息由计算一维PSD得到。PSD定义式为
PSD1(f)=limL→∞|z(f,L)|2L (1) 式中：L是采样长度，f是空间频率，z（f，L）是表面微观轮廓傅里叶变换的结果。z（f，L）的计算公式为
z(f,L)=∫L/2−L/2z(x)exp(−j2πfx)dx (2) 式中：z（x）是微观轮廓高度信息。
1.2 连续小波提取方法
空间域中，小波变换（WT）定义为分析原始信号f（x）的一系列函数，即小波基函数ψ（x）。ψa，b（x）是小波基ψ（x）根据尺度因子a和平移因子b构成的一组函数。连续小波变换（CWT）是对空间频率进行多尺度变换[23]，能有效地从微观形貌中提取实际频率特征。CWT定义式为
ψa,b(x)=|a|−1/2ψ(x−ba) (3) Wψ(f)(a,b)=1√a∫∞−∞f(t)ψ(t−ba)dt (4) 式中：Wψ是连续小波系数，由ψ（x）（本文取Mexican小波基）、尺度因子a、平移因子b（本文中b＝0）共同决定。
尺度因子a为轮廓频率特征信息与小波基中心频率的变换关系。频率特征与a对应关系为
a=fcfsΔ (5) 式中：fc是Mexican小波基中心频率（fc＝0.25）；Δ是白光干涉仪采样周期；fs是实际频率特征的中心频率。其中fs可表示为
fs=fLN/2 (6) 式中：f是PSD提取表面轮廓的实际频率，N是采样长度上采样点数目。
式（4）的小波系数Wψ表示原信号与小波基相似程度，与轮廓频率特征无关。需对小波系数逆变换，定义式为
f(t)=1Cψ∫a∫b[Wψ(f)(a,b)]ψa,b(t)dbdaa2 (7) 式中：f（t）是小波系数重构的实际频率特征；Cψ是小波容许常数。
1.3 表面轮廓均方根表征
微观形貌处于nm量级时，仍能用描述表面微观轮廓大小的传统统计参数，如轮廓均方根Rq表征轮廓垂直方向的高度信息，可表示为
Rq=√∫L0[z(x)]2dxL (8) 式中：z（x）为轮廓高度。
1.4 实验
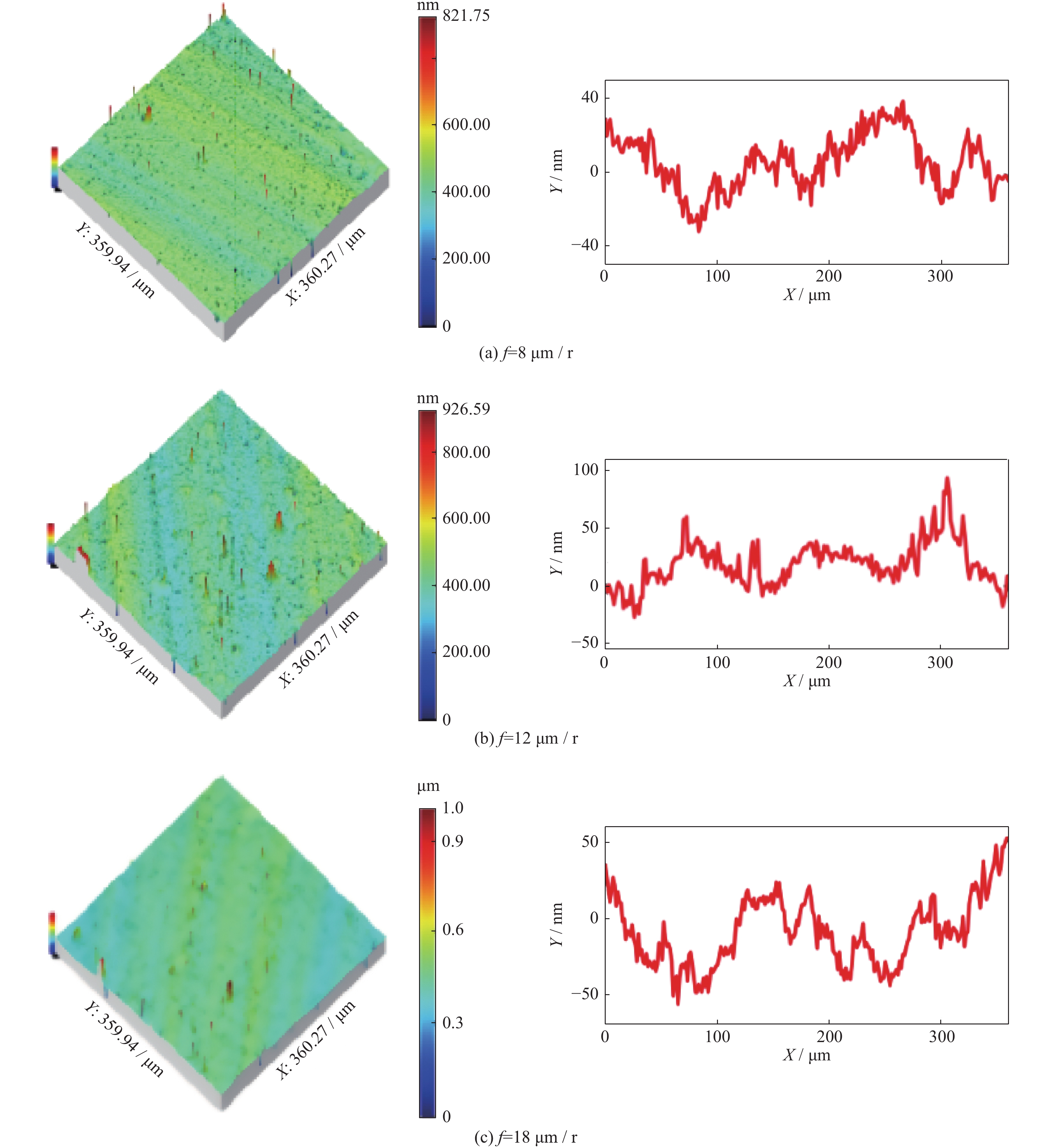
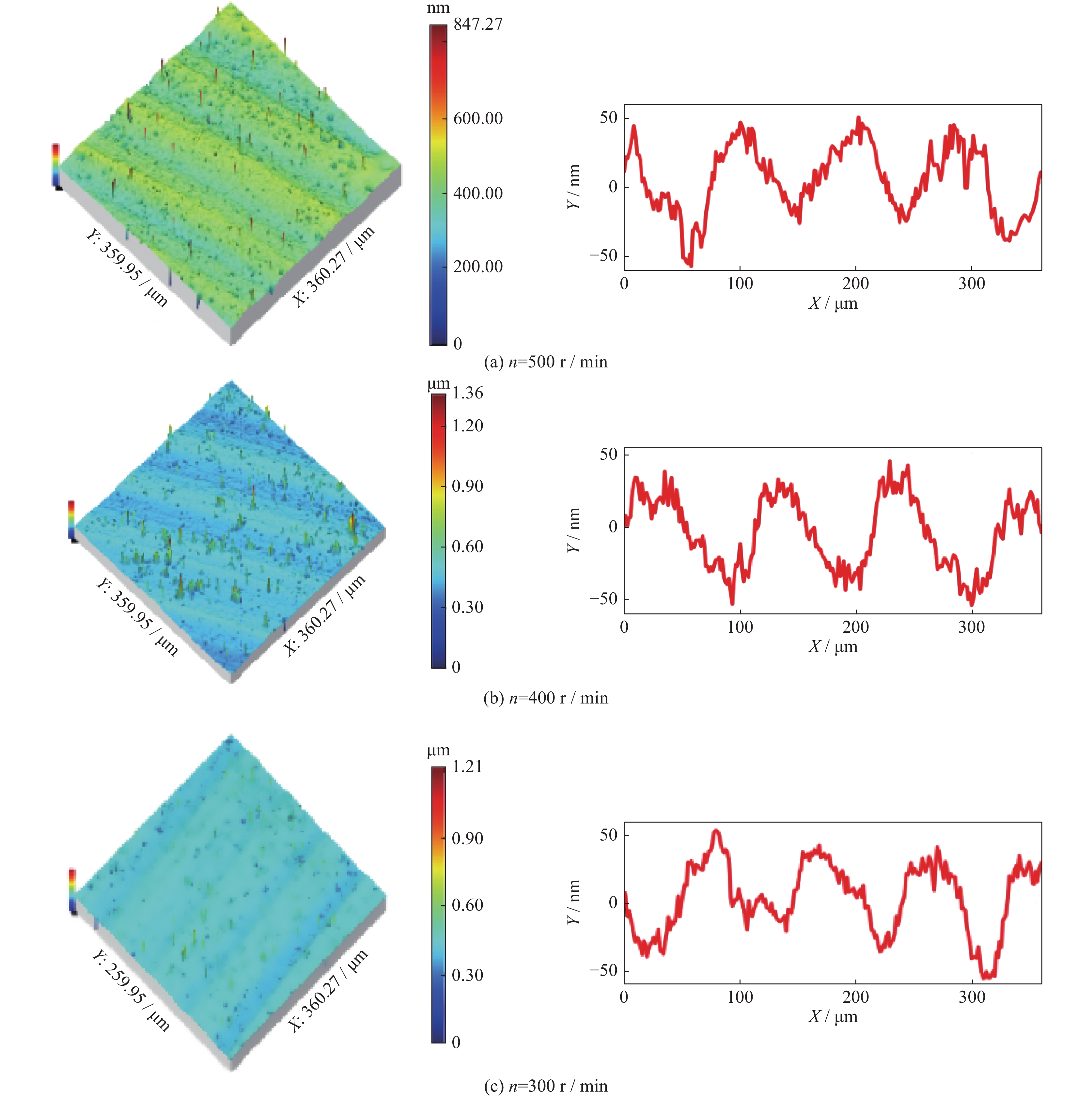
本文中KDP单晶表面（001）由单点金刚石车削获得。刀具参数见表1，切削方式为端面车削，选取切削参数见表2。
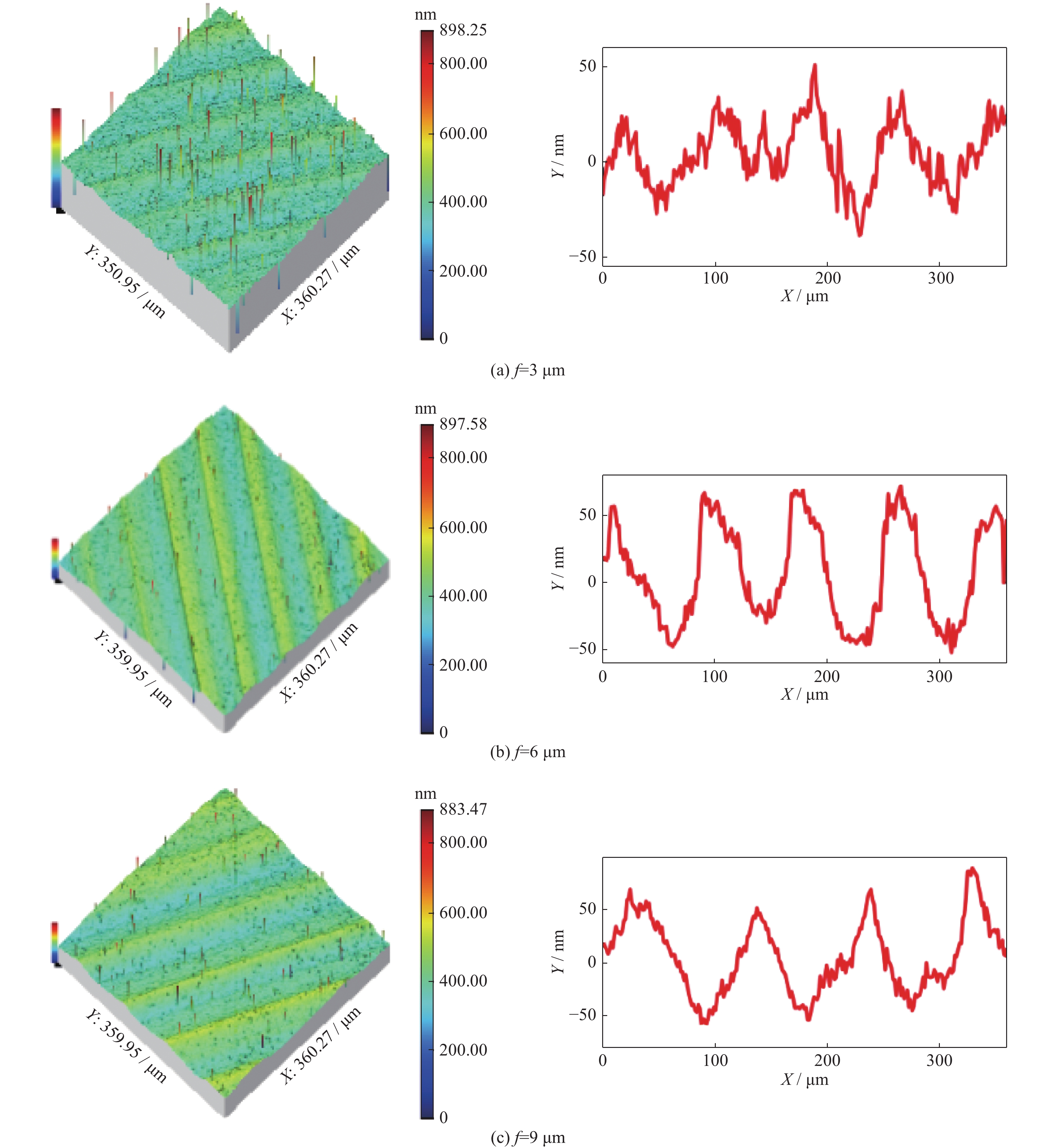
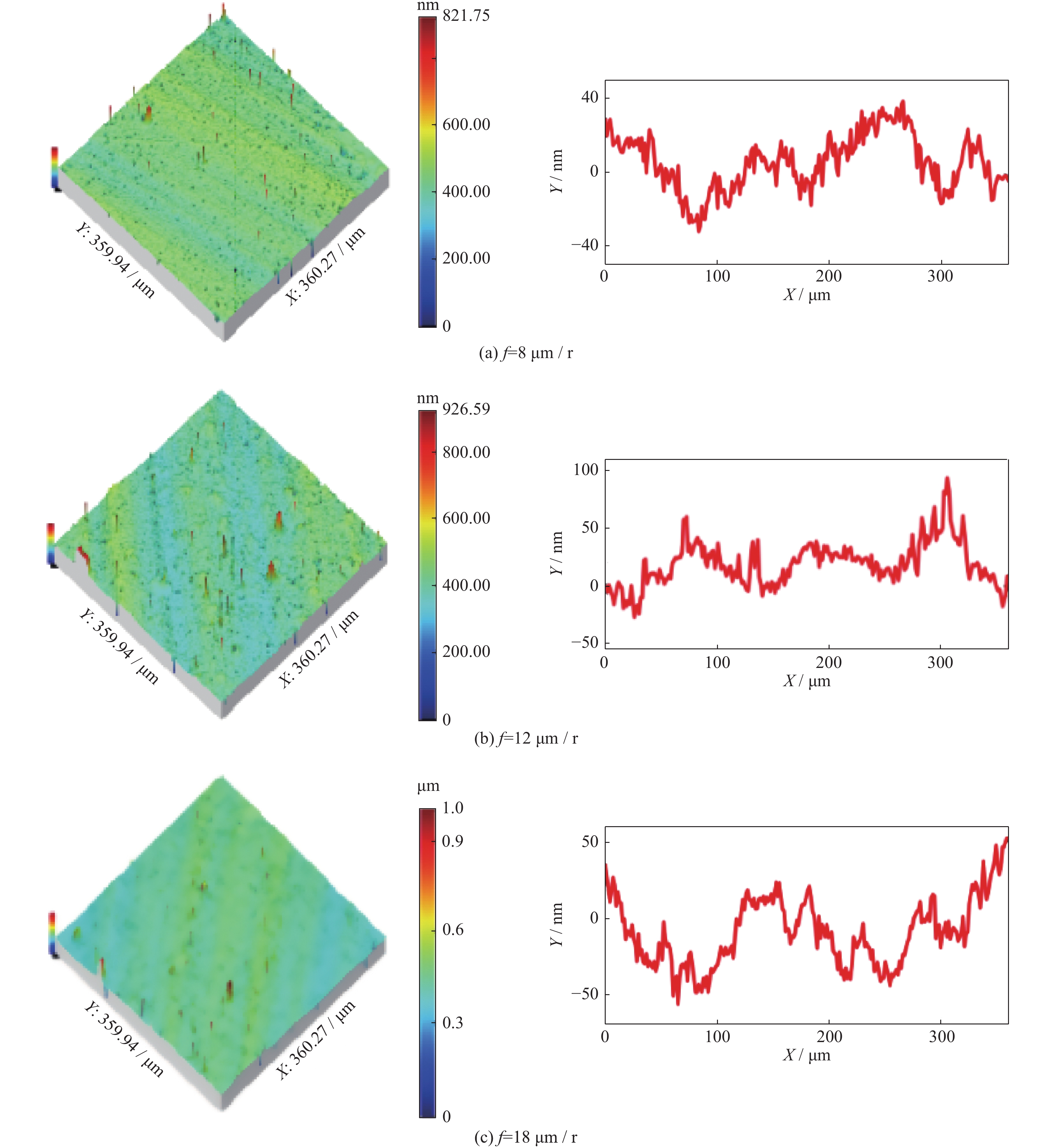
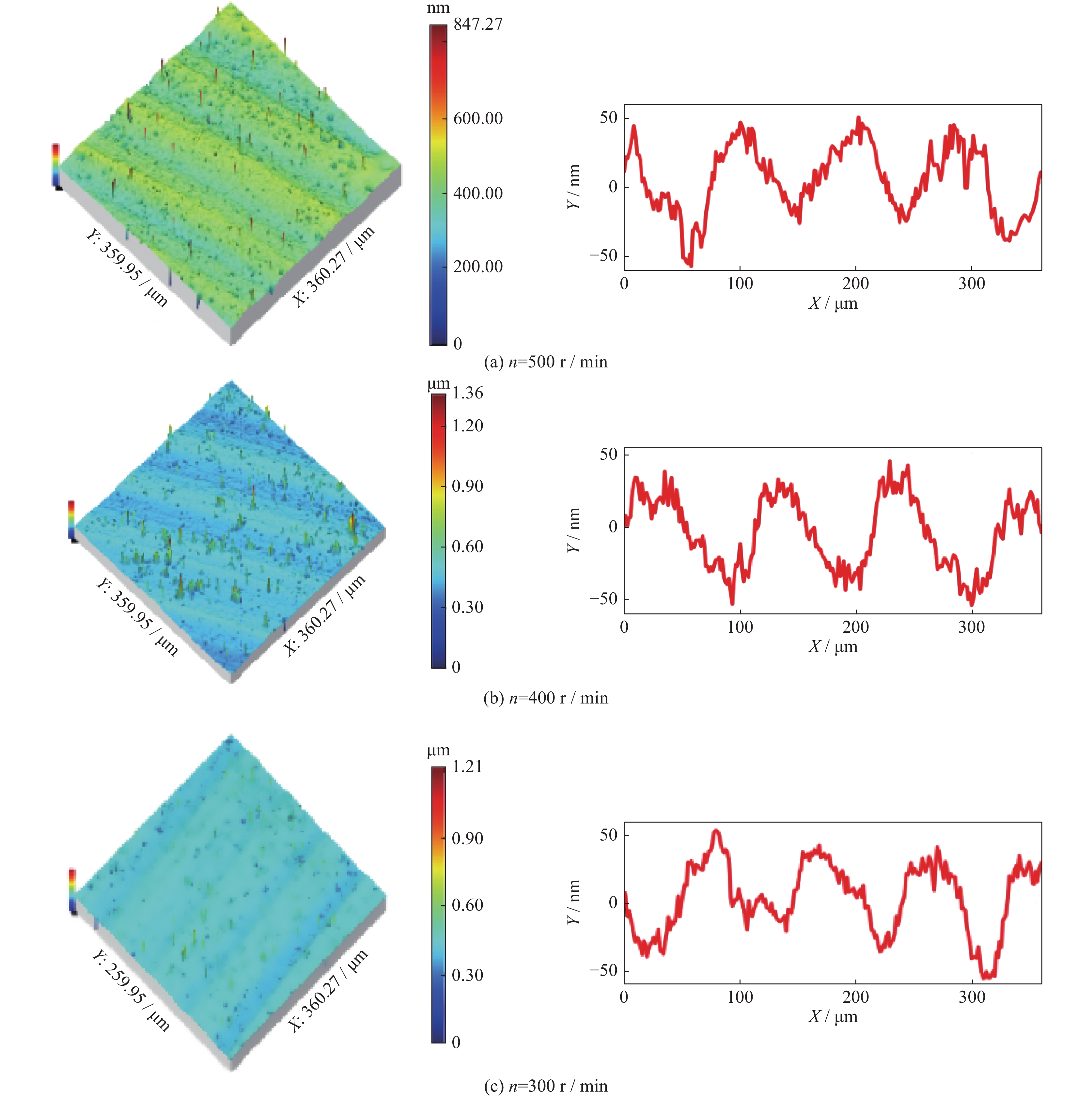
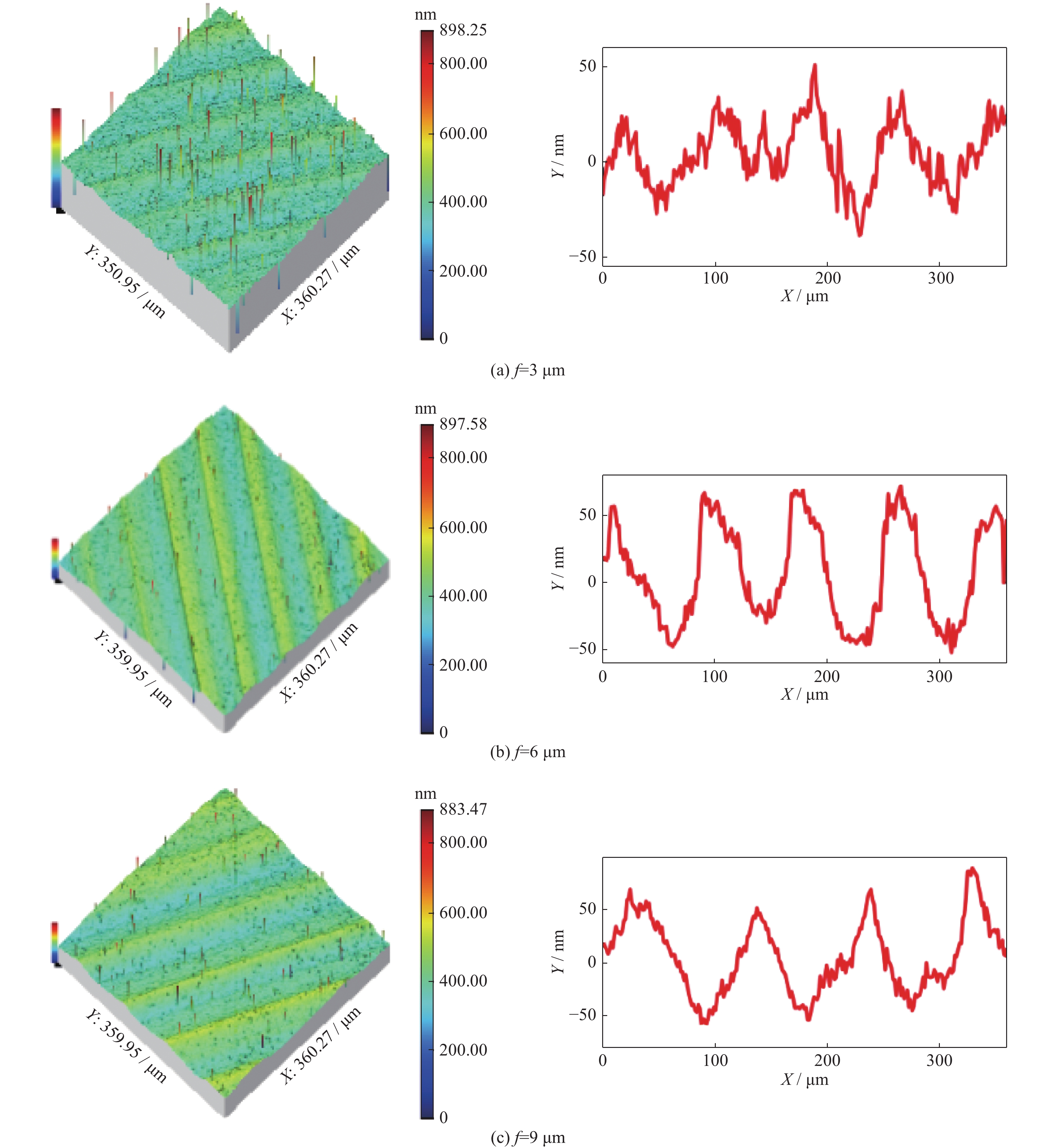
表 1 圆弧刃天然单晶金刚石车刀参数Table 1. Parameters of diamond turning toolnose radius r/mm edge radius rn/nm rake angle γ0/(°) clearance angle α0/(°) 3.2 150 0 9 表 2 切削参数及其轮廓均方根比较Table 2. Cutting parameters and RMS of contourNo.（group） spindle speed n/(r/min) feed rate f /(μm/r) depth of cut ap/μm RMS of contour Rq/nm 1 400 10 3 17 6 35 9 37 2 300 8 3 15 12 24 18 27 3 500 10 5 25 400 24 300 26 采样样本通过白光干涉仪测量获得，采样面积360 μm×360 μm;采样点数256×256；最小采样周期1.412 μm/pixel（360 μm/255 pixels），如图1～3所示。根据采样大小确定公式（5），（6）中参数取值Δ＝1.412，L＝360 μm，N＝256。图1（a）左为例，能看到相对运动的走刀痕迹。轮廓纹理需提取垂直走刀方向上高度信息，如图1（a）右所示，轮廓均方根Rq见表2。
图 1 白光干涉仪测量的变切削深度时晶体表面和轮廓Figure 1. Crystal surface and contour at different cutting depth measured by a white light interferometer
图 2 白光干涉仪测量的变进给量时晶体表面和轮廓Figure 2. Crystal surface and contour at different feed rate measured by a white light interferometer
图 3 白光干涉仪测量的主轴转速改变时晶体表面和轮廓Figure 3. Crystal surface and contour at different spindle speed measured by a white light interferometer2. 分析
2.1 PSD分析
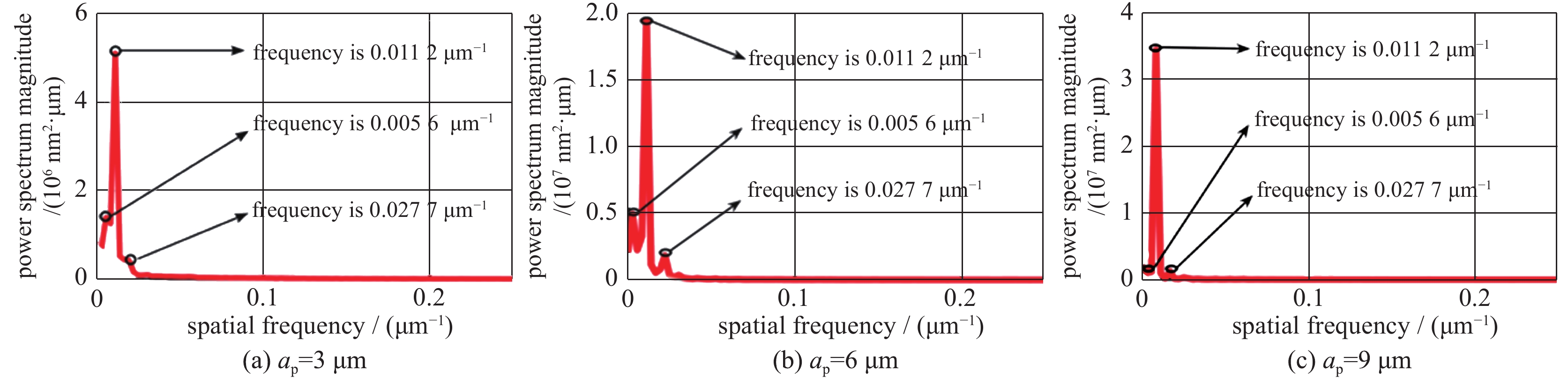
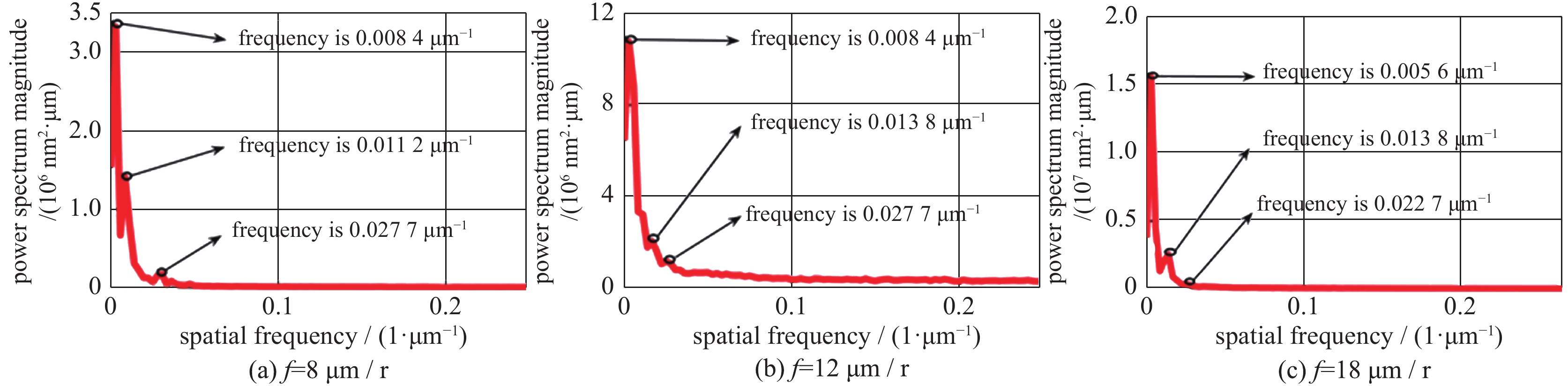
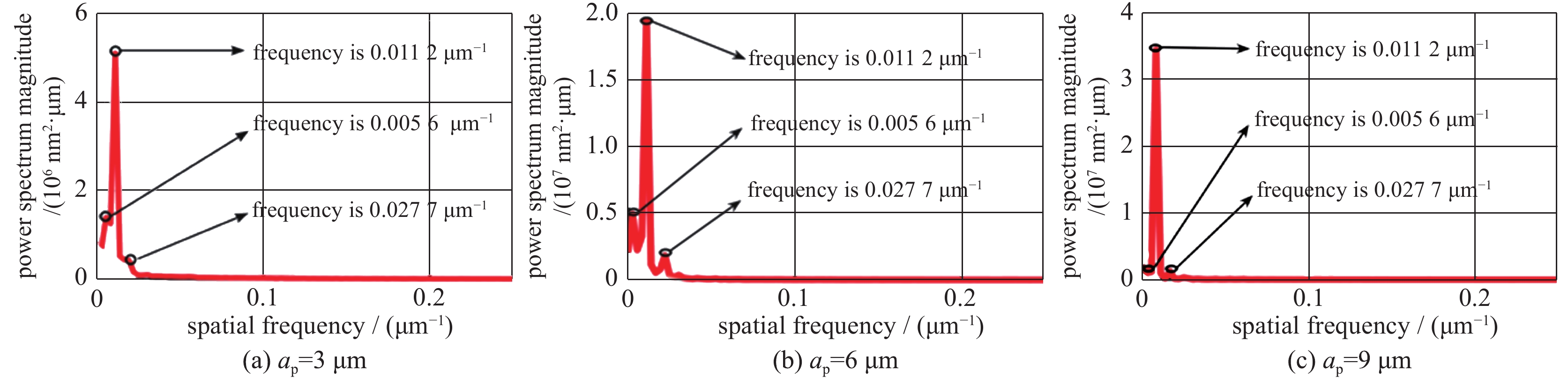
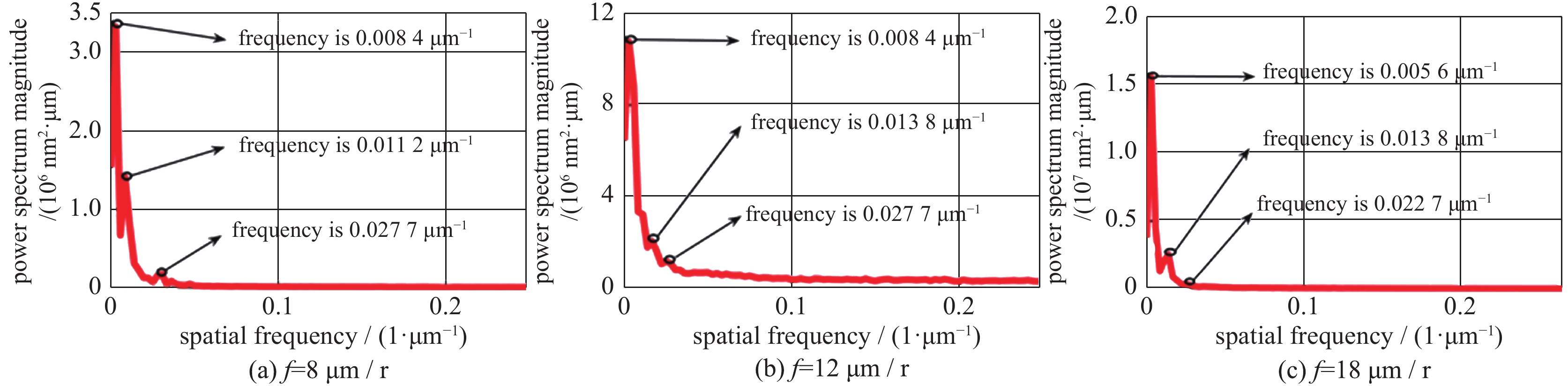
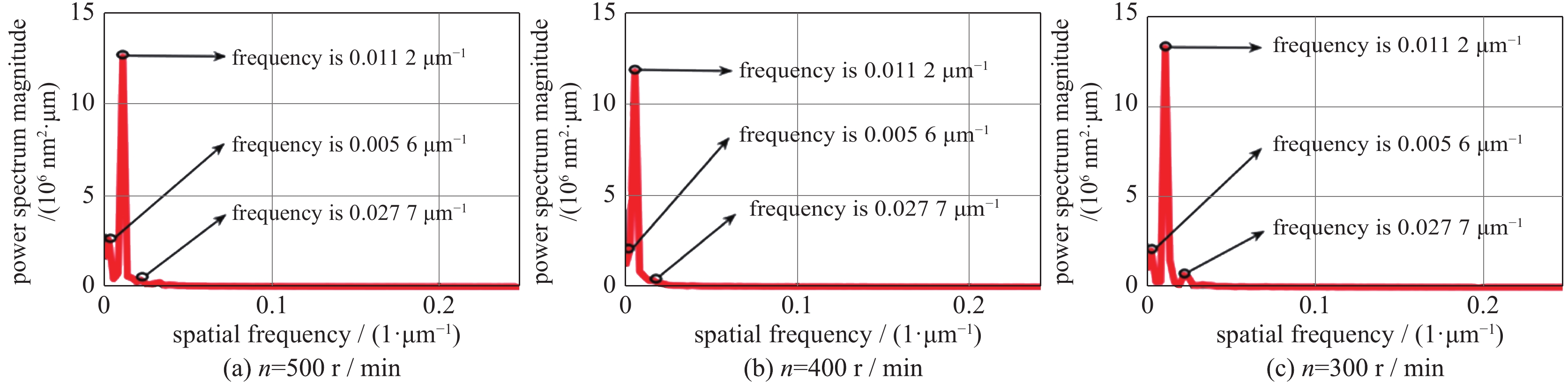
通过PSD计算获得取样域内频率值及其功率量。根据白光干涉仪测量参数，确定取样周期范围2.824～360 μm，频率取值范围0.002 8～0.354 μm−1，最小频率间隔为0.002 8 μm−1。因频率取值为最小间隔的整数倍，只得到取值范围内的固定频率及其功率量。为弥补轮廓上部分频率信息遗漏，连续小波变换时需对频率段进行提取及重构。式（1），（2）计算表面微观轮廓的功率谱密度大小，如图4～6所示。
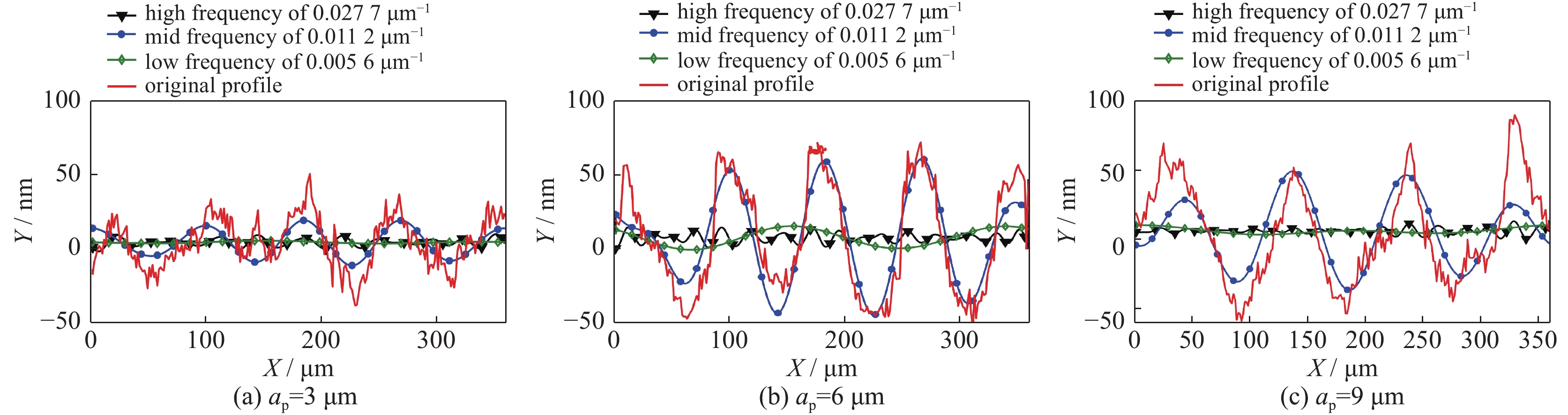
图4在转速n＝400 r/min，进给量f＝10 μm/r，切削深度ap分别为3、6、9 μm时PSD计算结果。可以看出，切削深度变化时，PSD值在频率0.011 2 μm−1处最大，说明该值是影响表面微观轮廓的主要频率，且随切深变大，功率量也相应增加。频率为0.005 6、0.027 7 μm−1存有少量功率量，是影响表面轮廓的次要频率。
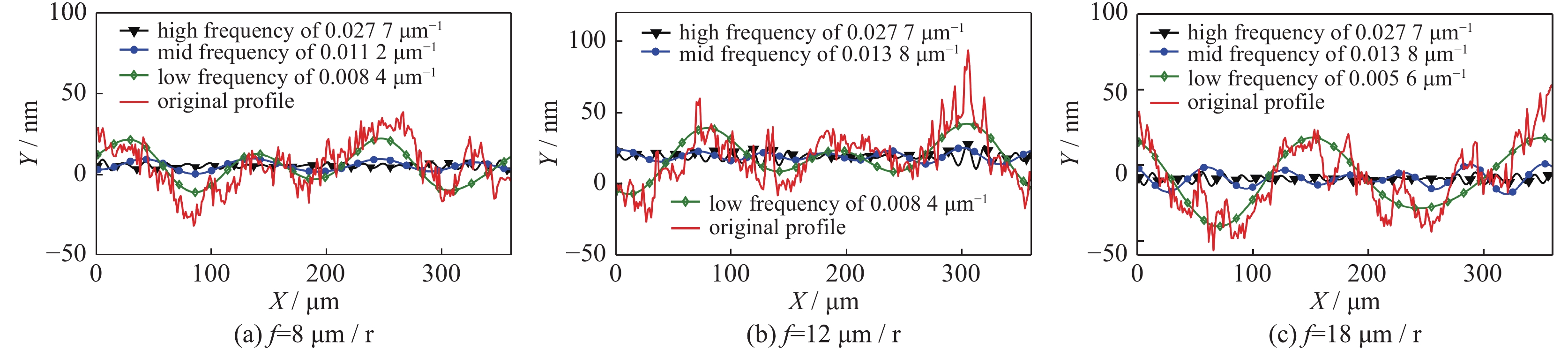
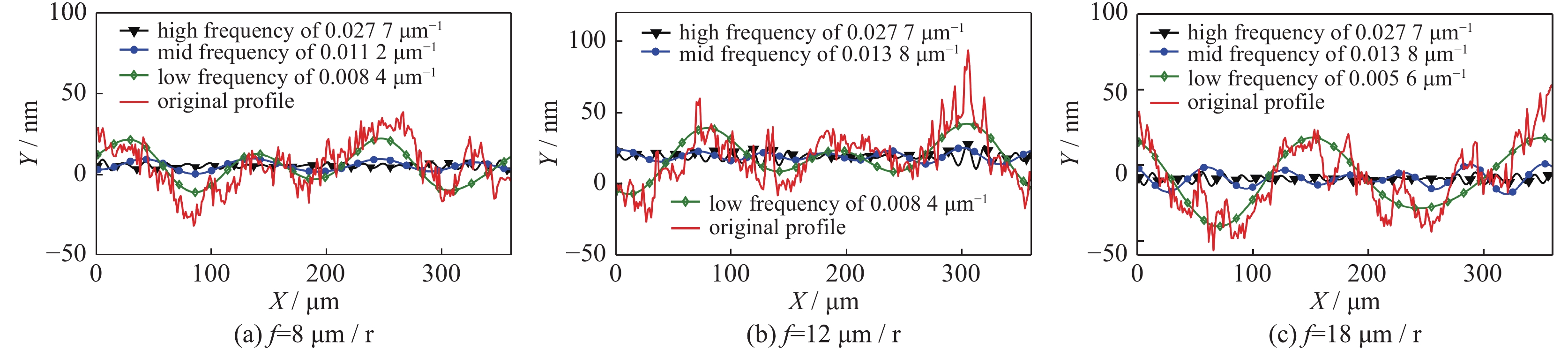
图5在转速n＝300 r/min，切深ap＝3 μm，进给量f分别为8、12、18 μm/r时PSD计算结果。可以看到，变进给量时主频值发生变化，进给量f为8、12 μm/r时主频率为0.008 4 μm−1，f为18 μm/r时主频率为0.005 6 μm−1。这是因为轮廓细密程度随进给量减少变得细密，采样范围内纹理增多，反应在PSD上为主频值右移。图5（a），（b）也可看出，进给量减小时中频特征（0.011 2、0.013 8 μm−1）有向低频过渡的趋势，低频成为进给量变化时主要频率，其重构波形对表面轮廓影响程度慢慢变小。
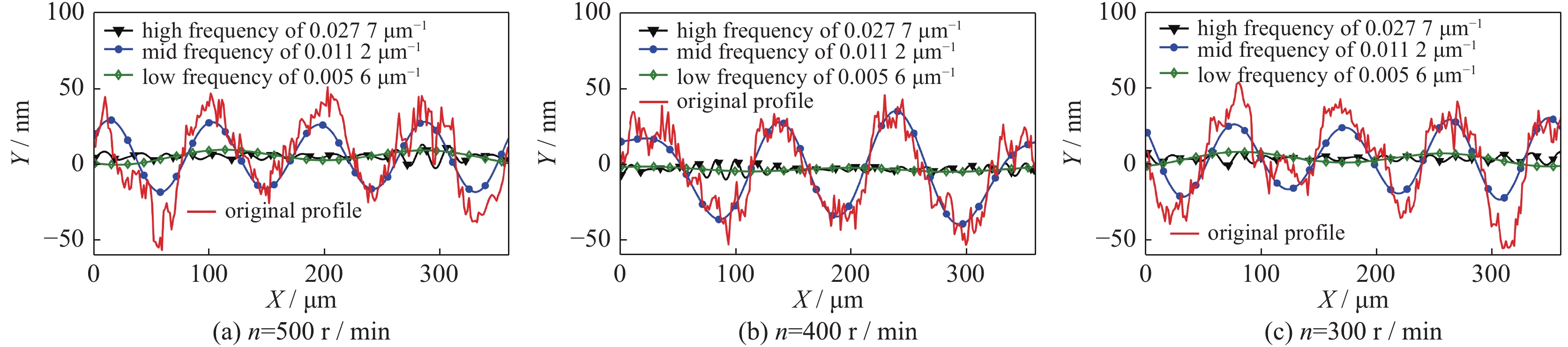
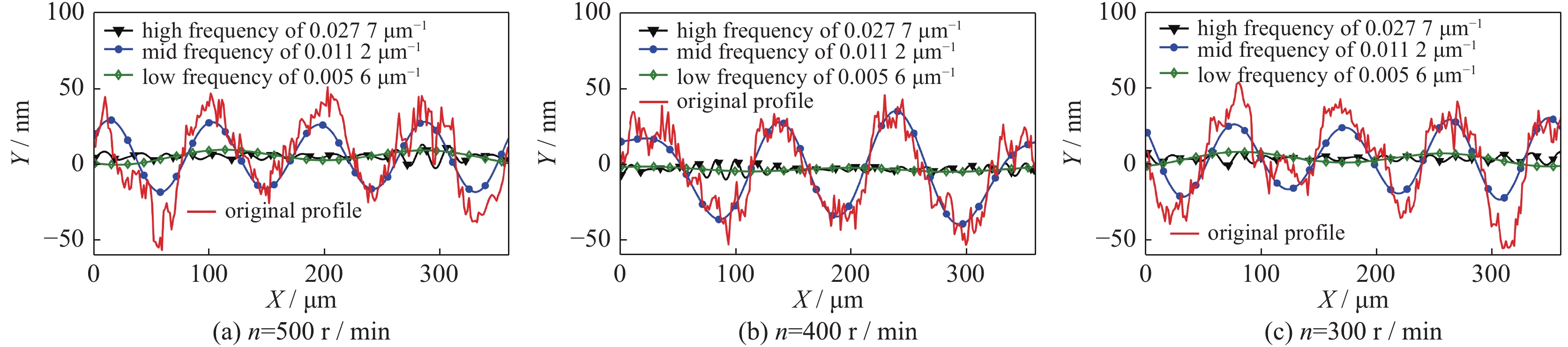
图6在切削深度ap＝5 μm，进给量f ＝10 μm/r，主轴转速n分别为500、400、300 r/min时PSD计算结果。频率0.011 2 μm−1是转速变化时影响微观轮廓的主要频率。图6（a），（b），（c）具有相同的频率分布，表明转速不会影响频率值变化，只影响功率量大小。说明这三个轮廓拥有近似频率特征，其重构波形有相似性。
2.2 频率特征提取及重构
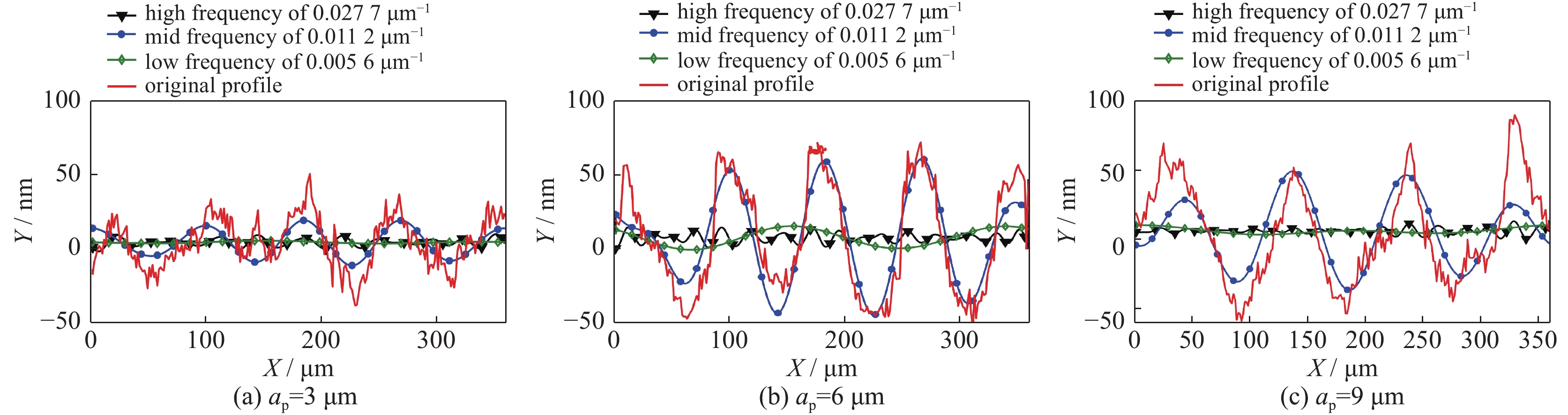
超精加工表面形貌可看作是多尺度波纹无序叠加组成。图4～6选取较为明显的频率值进行频率特征提取及重构。为保证分析过程及结果准确一致，变切削深度和变转速条件下选择低、中、高频率值分别为0.005 6，0.011 2，0.027 7 μm−1；变进给量情况下提取及重构频率值分别为0.005 6，0.008 4，0.011 2，0.013 8，0.027 7 μm−1。式（5），（6）中小波参数及尺度计算结果见表3。
表 3 尺度因子及其计算过程参数Table 3. Scale a and its parameters of calculationsfrequency f/μm−1 size L/μm number N waviness central frequency fs mexihat central frequency fc sampling period Δ scale a low 0.005 6 360 256 0.015 8 0.25 1.412 11.209 3 0.008 4 0.023 7 7.472 9 mid 0.011 2 0.031 2 5.674 8 0.013 8 0.038 8 4.563 2 high 0.027 7 0.077 9 2.272 8 式（3），（4）得到的小波系数与表面轮廓并无实质联系。为获得轮廓频率特征信息需对小波系数逆变换，由式（7）重构微观轮廓频率特征。
2.3 切削参数对频率特征的影响
切削参数对微观轮廓影响主要体现在频率特征的波长及幅值上。图7为变切削深度时轮廓与实际频率特征进行比较。频率为0.011 2 μm−1重构的波形与原始轮廓最为接近，频率值为0.005 6、0.027 7 μm−1含有少量轮廓信息，与PSD分析结果一致。由图7的原始轮廓比较发现：其周期及振幅随切削深度改变而变化，具体表现为切削越深，轮廓周期明显，幅值变大。这与重构中频0.011 2 μm−1的波形特征保持一致。切削深度对高、低频轮廓影响较小，在幅值和波长上无明显变化。均方根Rq分别为17，35，37 nm，得到ap＝ 3 μm是本文中较为合适的切削深度。
图 7 变切削深度时原始轮廓与实际频率特征比较Figure 7. Comparison of surface profile and actual frequency characteristics at different depth of cut图8为变进给量时轮廓与实际频率特征进行比较。低频特征（0.008 4、0.005 6 μm−1）的大小及走势与原始轮廓最为接近，视为主频率。对比低频特征发现，在进给量增加时，频率值变小，其特征表现为波长变长，幅值增加，即进给量变化会影响主频率值变化，随进给量增大趋向低频，振幅变大。进给量变化对中、高频无规律变化。均方根Rq分别为15、24、7 nm，得到f＝8 μm/r是本文较为适宜的进给量。
图 8 变进给量时原始轮廓与实际频率特征比较Figure 8. Comparison of surface profile and actual frequency characteristics at different feed rate图9为变转速时原始轮廓与实际频率特征进行比较。在变转速情况下，中频特征（0.011 2 μm−1）与原始轮廓走势一致。高频特征（0.027 7 μm−1）反映了轮廓的细节变化，与加工过程中的振动和材料自身性质有关。转速变化对高、低频无明显影响。均方根Rq分别为25，24，26 nm，得到n＝400 r/min是本文的理想转速。
图 9 变主轴转速时原始轮廓与实际频率特征比较Figure 9. Comparison of surface profile and actual frequency characteristics at different spindle speed3. 结 论
通过分析切削参数变化对KDP晶体已加工表面实际频率特征的影响，可得到以下结论：（1）功率谱密度结合连续小波的方法能够获得采样域内的频率特征，将表面微观轮廓信息按实际频率进行提取及重构。获得影响微观形貌的主频率，建立加工参数与实际频率特征的关系；（2）切削参数对表面微观形貌的影响归结于实际频率特征上。中频特征反映了切深与主轴转速信息；进给量变化会影响低频值发生改变，频率特征随进给量增加时表现为波长变大、幅值增加。通过实际频率特征分析对评价加工工艺和光学晶体表面质量有着重要指导意义；（3）PSD仅能获得取样长度范围内取样步长整数倍的频率，但表面形貌上空间频率是连续分布的。通过连续小波重构可以在某种程度上弥补PSD方法在分析频率特征连续性的不足，提取出微观轮廓上的实际频率特征。
-
图 1 白光干涉仪测量的变切削深度时晶体表面和轮廓
Figure 1. Crystal surface and contour at different cutting depth measured by a white light interferometer
图 2 白光干涉仪测量的变进给量时晶体表面和轮廓
Figure 2. Crystal surface and contour at different feed rate measured by a white light interferometer
图 3 白光干涉仪测量的主轴转速改变时晶体表面和轮廓
Figure 3. Crystal surface and contour at different spindle speed measured by a white light interferometer
图 7 变切削深度时原始轮廓与实际频率特征比较
Figure 7. Comparison of surface profile and actual frequency characteristics at different depth of cut
图 8 变进给量时原始轮廓与实际频率特征比较
Figure 8. Comparison of surface profile and actual frequency characteristics at different feed rate
图 9 变主轴转速时原始轮廓与实际频率特征比较
Figure 9. Comparison of surface profile and actual frequency characteristics at different spindle speed
表 1 圆弧刃天然单晶金刚石车刀参数
Table 1. Parameters of diamond turning tool
nose radius r/mm edge radius rn/nm rake angle γ0/(°) clearance angle α0/(°) 3.2 150 0 9 
下载: 导出CSV
表 2 切削参数及其轮廓均方根比较
Table 2. Cutting parameters and RMS of contour
No.（group） spindle speed n/(r/min) feed rate f /(μm/r) depth of cut ap/μm RMS of contour Rq/nm 1 400 10 3 17 6 35 9 37 2 300 8 3 15 12 24 18 27 3 500 10 5 25 400 24 300 26
下载: 导出CSV
表 3 尺度因子及其计算过程参数
Table 3. Scale a and its parameters of calculations
frequency f/μm−1 size L/μm number N waviness central frequency fs mexihat central frequency fc sampling period Δ scale a low 0.005 6 360 256 0.015 8 0.25 1.412 11.209 3 0.008 4 0.023 7 7.472 9 mid 0.011 2 0.031 2 5.674 8 0.013 8 0.038 8 4.563 2 high 0.027 7 0.077 9 2.272 8
下载: 导出CSV
-
[1] Wang Hui, Zhang Zheng, Liu Tianye, et al. Surface error modeling, evaluation and optimization of large optics in inertial confinement fusion laser system[J]. Fusion Eng Des, 2018, 137: 61-70. doi: 10.1016/j.fusengdes.2018.08.005 [2] NayarB K. Nonlinear optical properties of organic molecules and crystals[M]. Elsevier, 2012. [3] Tie Guipeng, Dai Yifan, Guan Chaoliang, et al. Research on full-aperture ductile cutting of KDP crystals using spiral turning technique[J]. J Mater Process Tech, 2013, 213(12): 2137-2144. doi: 10.1016/j.jmatprotec.2013.06.006 [4] Chen Ni, Chen Mingjun, Wu Chunya, et al. The design and optimization of micro polycrystalline diamond ball end mill for repairing micro-defects on the surface of KDP crystal[J]. Precis Eng, 2016, 43: 345-355. doi: 10.1016/j.precisioneng.2015.08.015 [5] Li Zhanjie, Jin Gang, Fang Fengzhou, et al. Ultrasonically assisted single point diamond turning of optical mold of tungsten carbide[J]. Micromachines, 2018, 9(2): 77-87. doi: 10.3390/mi9020077 [6] Cheng Jian, Xiao Yong, Liu Qi, et al. Effect of surface scallop tool marks generated in micro-milling repairing process on the optical performance of potassium dihydrogen phosphate crystal[J]. Mater Design, 2018, 157: 447-456. doi: 10.1016/j.matdes.2018.07.057 [7] Wang Yongqiang, Yin Shaohui, Huang Han, et al. Magnetorheological polishing using a permanent magnetic yoke with straight air gap for ultra-smooth surface planarization[J]. Precis Eng, 2015, 40: 309-317. doi: 10.1016/j.precisioneng.2014.11.001 [8] Ji Fang, Xu Min, Wang Baorui, et al. Preparation of methoxyl poly (ethylene glycol) (MPEG)-coated carbonyl iron particles (CIPs) and their application in potassium dihydrogen phosphate (KDP) magnetorheological finishing (MRF)[J]. Appl Surf Sci, 2015, 353: 723-727. doi: 10.1016/j.apsusc.2015.06.063 [9] Peng Wenqiang, Li Shenyi, Guan Chaoliang, et al. Ultra-precision optical surface fabricated by hydrodynamic effect polishing combined with magnetorheological finishing[J]. Optik-International Journal for Light and Electron Optics, 2018, 156: 374-383. doi: 10.1016/j.ijleo.2017.11.055 [10] Clark W I, Shih A J, Hardin C W, et al. Fixed abrasive diamond wire machining—part I: Process monitoring and wire tension force[J]. Int J Mach Tool Manu, 2003, 43(5): 523-532. doi: 10.1016/S0890-6955(02)00215-8 [11] Pang Qilong, Kuang Liangjie, Xu Youlin, et al. Study on the extraction and reconstruction of arbitrary frequency topography from precision machined surfaces[J]. P I Mech Eng B-J Eng, 2019, 233(7): 1772-1780. [12] Chen Ni, Chen Mingjun, Wu Chunya, et al. Cutting surface quality analysis in micro ball end-milling of KDP crystal considering size effect and minimum undeformed chip thickness[J]. Precis Eng, 2017, 50: 410-420. doi: 10.1016/j.precisioneng.2017.06.015 [13] Chen Ni, Chen Mingjun, Guo Yanqiu, et al. Effect of cutting parameters on surface quality in ductile cutting of KDP crystal using self-developed micro PCD ball end mill[J]. Int J Adv Manuf Tech, 2015, 78(1/4): 221-229. [14] Zheng Wei, Zhou Ming, Zhou Li. Influence of process parameters on surface topography in ultrasonic vibration-assisted end grinding of SiCp/Al composites[J]. Int J Adv Manuf Tech, 2017, 91(5/8): 2347-2358. [15] Wang Shengfei, An Chenhui, Zhang Feihu, et al. An experimental and theoretical investigation on the brittle ductile transition and cutting force anisotropy in cutting KDP crystal[J]. Int J Mach Tool Manu, 2016, 106: 98-108. doi: 10.1016/j.ijmachtools.2016.04.009 [16] Tian Fujing, Yin Ziqiang, Li Shengyi. Theoretical and experimental investigation on modeling of surface topography influenced by the tool-workpiece vibration in the cutting direction and feeding direction in single-point diamond turning[J]. Int J Adv Manuf Tech, 2016, 86(9/12): 2433-2439. [17] Wang Xuezhi, Yu Tianbiao, Dai Yuanxing, et al. Kinematics modeling and simulating of grinding surface topography considering machining parameters and vibration characteristics[J]. Int J Adv Manuf Tech, 2016, 87: 2459-2470. doi: 10.1007/s00170-016-8660-y [18] Krolczyk G M, Maruda R W, Krolczyk J B, et al. Parametric and nonparametric description of the surface topography in the dry and MQCL cutting conditions[J]. Measurement, 2018, 121: 225-239. doi: 10.1016/j.measurement.2018.02.052 [19] Zhang Qing, Zhang Song, Shi Wenhao. Modeling of surface topography based on relationship between feed per tooth and radial depth of cut in ball-end milling of AISI H13 steel[J]. Int J Adv Manuf Tech, 2018, 95(9/12): 4199-4209. [20] Wei Weihua, Li Yuantong, Xue Tongming, et al. Research on milling forces during high-speed milling of wood-plastic composites[J]. BioResources, 2018, 14(1): 769-779. [21] Wei Weihua, Li Yuantong, Mei Changtong, et al. The research progress of machining mechanisms in milling wood-based materials[J]. BioResources, 2018, 13(1): 2139-2149. [22] Itoh T, Yamauchi N. Surface morphology characterization of pentacene thin film and its substrate with under-layers by power spectral density using fast Fourier transform algorithms[J]. Appl Surf Sci, 2007, 253(14): 6196-6202. doi: 10.1016/j.apsusc.2007.01.056 [23] Nieslony P, Krolczyk G M, Wojciechowski S, et al. Surface quality and topographic inspection of variable compliance part after precise turning[J]. Appl Surf Sci, 2018, 434: 91-101. doi: 10.1016/j.apsusc.2017.10.158 期刊类型引用(0)
其他类型引用(6)
-
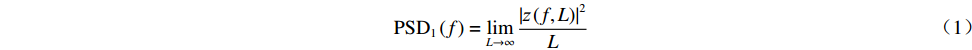
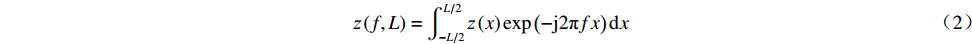
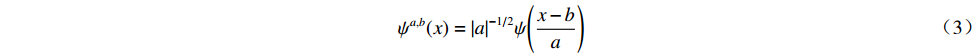
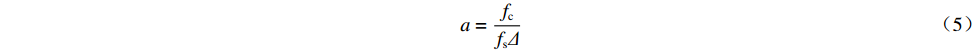
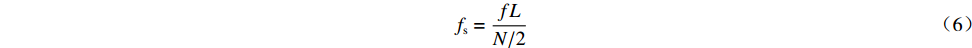
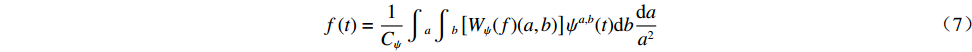
下载:
点击查看大图
计量
- 文章访问数: 1399
- HTML全文浏览量: 718
- PDF下载量: 60
- 被引次数: 6
