Calculation method and modes of radiation damage for single element target
-
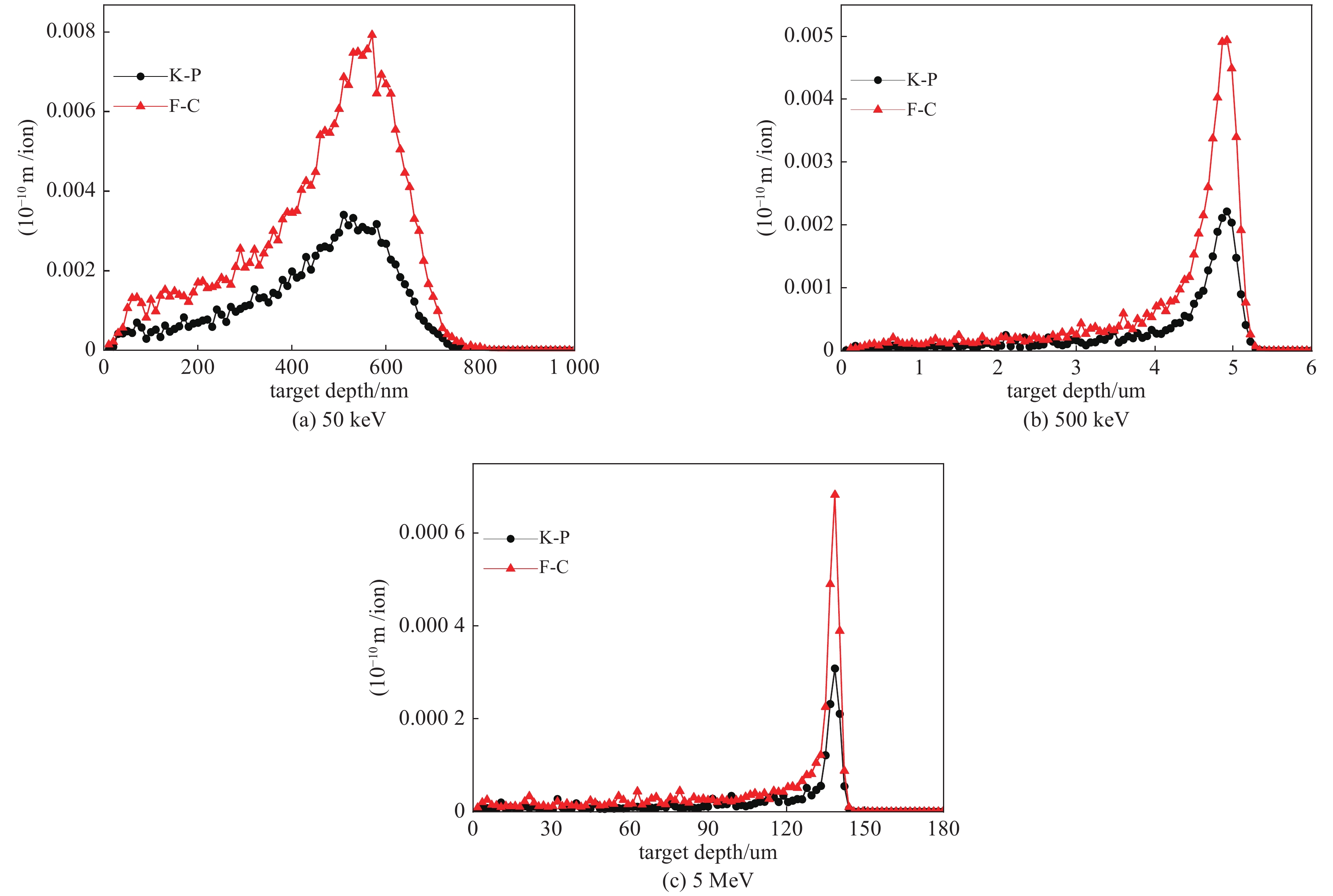
摘要: 通过SRIM程序的快速损伤计算与全级联计算两种常用模式，对单元素靶材料进行粒子辐照模拟计算，分别利用基于损伤能量间接计算移位数的NRT位移模型方法和直接通过输出文件读取的方法获得移位数，并对数据进行相应的处理及分析对比，结果表明：对于单元素靶来说，在SRIM快速损伤和全级联两种计算模式下，利用NRT位移模型数值计算得到的移位数基本一致，都可以用于进一步计算得到可靠的位移损伤剂量（dpa）；而通过SRIM两种模式下的输出文件数据直接获得的移位数则有两倍左右的差异，要想得到相对可靠的dpa相关参数，需要根据不同辐照情况选取合适的计算模式。Abstract: The ion irradiation will cause different degrees of radiation damage to the target material. One of the important physical parameters to evaluate the radiation damage is dpa, that is, the displacements per atom. The Monte Carlo method based SRIM simulation program, which describes the collision and energy loss between ions and the target, is widely used to calculate many parameters related to particle beam irradiation. The number of atomic shifts per unit depth per incident particle can also be calculated as an important parameter in dpa. In this paper, the single element target material is simulated by two common models of SRIM program. The NRT mathematical model based on damage energy is used to calculate the number of displacements indirectly and the output file is used to get the displacements number directly. The results show that for the single element target, the displacements calculated by the mathematical model are basically the same under the two modes of SRIM Quick damage and SRIM Full cascade, which can be used to calculate the dpa reliably. The displacements obtained directly from the output file of the two modes have about two-fold difference. To get the reliable dpa parameters, it is necessary to select the appropriate calculation mode according to different irradiations.
-
Key words:
- radiation damage /
- displacement /
- damage energy /
- NRT model /
- quick damage /
- full cascade
-
自福岛核电站事故发生以来，反应堆的安全性越来越受到公众的关注，成为影响核能发展的关键因素。采用非能动系统来提高反应堆的固有安全性已经在新的反应堆设计中得到了广泛的应用。其中，利用依靠回路内冷热段内冷却剂的密度差来产生驱动压头带走堆芯余热的自然循环成为主要的研究方向。目前，国际上对自然循环的研究以试验和数值模拟为主。试验研究方面，杨祖毛等[1]对单相自然循环进行了大量的试验，研究了稳态自然循环流量与热源功率、进出口温差的关系；黄彦平[2]通过试验研究了自然循环能力的影响因素等。但试验研究大多针对具体的回路及系统，不具有良好的移植性，且需要大量的资金投入。数值模拟方面，国内外通常使用一些系统分析程序，如RELAP5，RETRAN-02等大型商用软件，对于某些反应堆，也会采用独立开发的专用程序，如田文喜等[3]开发了CARR堆的自然循环能力计算程序，黄洪文等[4]开发了池式研究堆的自然循环能力分析程序，Kaminaga等[5]开发了JRR-3M自然循环能力程序。针对JRR-3M类型的研究堆，稳定自然循环形成前存在冷却剂流动方向反转的过程，有必要开展瞬态安全计算分析。本文利用RELAP5/Mod 3.4对JRR-3M进行了细致的堆芯建模，研究了不同事故条件下自然循环建立前的流量反转过程瞬态热工特性，并得到了瞬态时自然循环的载热极限，同时验证了计算模型和计算方法的可行性。
1. 计算模型
1.1 系统概述
RELAP程序是美国爱达荷国家工程实验室开发、美国核管理委员会批准的用于工程审评的大型瞬态热工水力计算程序，是一维瞬态、两流体六方程流体力学及点堆动力学模型[6]，现已广泛用于压水堆的事故模拟分析。JRR-3M是一座重水慢化、轻水冷却的池式研究堆，在正常运行工况下，冷却剂在两台主泵的驱动下从堆芯上部水池进入堆芯，向下流经堆芯带走裂变热后流入下部的下联箱，与换热器内二次侧的冷却水进行热交换冷却后重新流入主泵。在自然循环阶段，下联箱与水池连接的自然循环阀开启，池水在自然循环压头的驱动下进入下联箱，向上流过堆芯带走衰变热后返回水池，形成自然循环回路。应急冷却系统由两台由不间断电源供电的辅助泵组成，它们的额定流量为75.00 kg·s-1，最多能持续工作3 h。在发生场外电源丧失事故时，一台辅助泵投入运行，带走堆芯热量。
在一回路失去强迫流动后，存在一个由于冷却剂流动方向反转所导致的零流量时刻，此时由于没有冷却剂的流动带走衰变热，堆芯温度迅速上升，该时刻堆芯最有可能被烧毁。基于RELAP5/Mod 3.4程序，对JRR-3M进行建模，反应堆节点图如图 1所示。
其中，考虑到堆水池中存在明显的热分层现象以及内部循环，用两个管道(100，102)来模拟堆芯上部水池，两根管间用横向接管连接，最上方的控制体为空气层，时间控制体130表示空气的温度压力边界，取35 ℃和1.013×105 Pa。104和106是两种不同燃料组件的平均通道，108表示热通道，110和112表示旁路流道，堆芯流道均划分为15个控制体。114表示下联箱，116是环绕堆芯的水池，它们之间用自然循环阀连接。正常运行时从堆芯流出的冷却剂在热交换器118中与202中的冷却水进行冷却热交换。冷却剂由主泵流出后在分支部件126汇合后流回堆芯上部水池。
对于堆芯内功率的分布取该池式反应堆的物理设计结果，其功率衰变曲线采用中国行业标准EJ/T 745-2001[7]推荐公式计算给出, 即
P(τ0)=G(t)4∑i=1∫τ00Pi(t)Qif(t)dt (1) Q(t)=1.0+τ0.40φ(3.24×10−6+5.23×10−10t) (2) 式中：P(τ0)是τ0时刻的裂变总功率；G(t)是考虑裂变产物中子俘获的校正因子；Pi(t)是核素i在t时刻的裂变功率；Qi为核素i一次裂变可回收能量(MeV)；f(t)为可裂变核素的衰变热功率函数，它的值由标准附表给出(略)；φ为一个初始裂变原子的裂变数；i取1，2，3，4分别代表235U热中子裂变、239Pu热中子裂变、238U快中子裂变和241Pu热中子裂变对衰变热功率的贡献；t为停堆后时间(s)。
1.2 稳态参数
将系统调节至稳态工况是进行瞬态计算的前提条件。在自然循环的瞬态计算中，系统各个参数应在某个预计工况下稳态运行，随后关闭主泵打开自然循环阀进行瞬态计算，而这个稳态的工况就决定了事故的初始状态。通过调节回路内的物理参数，使得稳态工况下的热工参数与设计的热工参数一致且达到稳定。表 1为该池式研究堆的热工设计参数。
表 1 池式研究堆主要设计参数Table 1. Main design parameters of pool-type research reactortotal power/MW total mass flow rate/(kg·s-1) inlet temperature/℃ outlet temperature/℃ core inlet pressure/MPa 20.00 661.91 35.00 42.19 1.69 1.3 安全准则
按照该堆设计确定的安全标准为：
(1) 使用Sudo临界热流密度经验公式[8]，计算的最小偏离泡核沸腾比(MDNBR)应大于限值1.5。
(2) 燃料元件芯体最高温度低于250 ℃。
2. 计算结果与分析
2.1 无应急冷却的场外电源丧失事故分析
反应堆在满功率正常运行了100 s后发生外电源丧失事故，主泵由于失去电力供应立即失电惰转，二次侧热阱立即丧失，自然循环阀打开，1 s后控制棒落下反应堆进行停堆，此时堆芯所产生的热量主要依靠主泵惰转和自然循环带走。整个过程的事件序列列于表 2。
表 2 无应急冷却系统事件序列Table 2. Accident process without emergency cooling systemevent start time event start time main pump loss of off-site power supply 100 s control rods drop 101 s secondary side loss of hot trap 100 s finish main pump coasting 220 s open the natural circulation valve 100 s reverse coolant flow 221 s 图 2为事故分析的结果曲线。
从图 2(a)和图 2(b)中可以看出，在0~100 s内，反应堆满功率稳态运行，堆芯流量保持不变。在100 s时刻，由于主泵失电惰转流量迅速减少，在t=220 s时主泵惰转停止，堆芯流量减少至0。此时自然循环阀已经打开，在自然循环驱动压头的作用下，由于热通道中热流密度最大，故最先产生流量反转，随后堆芯流量反转，冷却剂由下而上地通过堆芯，自然循环迅速建立。
从图 2(c)看出，在稳态运行时，燃料芯块最热温度为108.27 ℃，包壳最高温度为99.37 ℃, 在101 s时控制棒开始下落后，由于堆芯功率的呈指数式降低，燃料板和冷却剂的温度也随之急剧下降。随后，由于冷却剂流量不足以完全带走燃料所产生的热量，热构件和冷却剂的温度逐渐上升。在220 s时冷却剂流量为0，燃料外表面与冷却剂的对流换热性能最差，燃料板的温度达到峰值，燃料芯块的峰值温度为114.30 ℃, 包壳峰值温度为114.07 ℃。此后，由于自然循环流量的增大，对流换热作用增强，燃料板和冷却剂的温度开始下降。在稳定的自然循环建立后，燃料板和冷却剂的温度由于堆芯功率的缓慢衰减而缓慢降低。
图 2(d)是整个过程中的MDNBR变化曲线。从图中可以看出，热管处的MDNBR在稳态运行时为2.66，在反应堆停堆后由于燃料板热流密度的急剧减少而迅速增加，随后随着流量的降低而降低，在流量反转时刻为最小值，最小值为0.31，远低于安全限值1.50。
2.2 有应急冷却的场外电源丧失事故分析
当该池式研究堆在满功率正常运行了100 s后发生外电源丧失事故，主泵失电惰转，当冷却剂流量低于75.00 kg·s-1时，一台辅助泵投入运行。当t=3 188 s时，衰变功率已降至200 kW，辅助泵停止运行，在辅助泵停止运行前5 s打开自然循环阀。事故过程中的事件时间序列如表 3所示。
表 3 有应急冷却事件序列Table 3. Accident process with emergency cooling systemevent start time event start time main pump loss of off-site power supply 100 s close auxiliary pump 3 188 s control rods drop 101 s auxiliary pump finish coast 3 198 s input auxiliary pump 110 s coolant flow reverse 3 199 s open the natural circulation valve 3 183 s 图 3为事故分析的结果曲线。
从图 3(a)和图 3(b)中可以看出，在100 s以前，反应堆满功率稳态运行，堆芯流量不变。100 s后主泵失电惰转，流量降低。当流量降低至75.00 kg·s-1时，辅助泵投入运行，堆芯流量保持不变。当t=3 183 s时，自然循环阀打开，从图中可以发现堆芯流量平台有一个下降。这时由于此时堆水池与反应堆一回路连接，具有了一定的流量，导致流过堆芯的流量减少。在t=3 188 s时关闭辅助泵，堆芯流量逐渐降低至0，随后由于自然循环的建立，流量发生反转，最后趋向稳定。
通过比较图 2(c)和图 3(c)可以发现，在投入应急冷却系统的事故工况下，由于堆芯拥有一定程度的强迫流量，这一部分的流量带走了部分衰变热，有效地降低了燃料板及冷却剂的温度，相较于未投入应急冷却系统的条件下，燃料芯块峰值温度降低了46.10 ℃，堆芯材料不会发生熔毁。同时可以发现冷却剂的温度和燃料板温度的变化趋势相似，但有一定的滞后。
从图 3(d)可以看出，有应急冷却时事故过程中的MDNBR始终大于安全限值，不会发生偏离核态沸腾，池式研究堆始终处于安全状态。
2.3 最大载热能力计算
为进一步探究池式研究堆自然循环瞬态过程中的最大载热能力，在堆芯功率衰减至不同功率水平时关闭辅助泵并打开自然循环阀，不同功率下自然循环过程中的最高燃料温度和MDNBR如图 4所示。
从图 4可以明显看出，此时反应堆的安全性主要受到来自MDNBR的限制，因此，池式研究堆最大瞬态自然循环能力约为590 kW。
3. 结论
本文利用系统性分析程序RELAP5/Mod 3.4对JRR-3M池式研究堆进行了建模，计算了在无应急冷却和有应急冷却情况下的丧失场外电源事故。RELAP5很好地模拟了堆芯流量的反转过程以及自然循环的建立过程。针对这两种事故工况，计算得到了堆芯各个流道的温度、压力、冷却剂流量等热工水力参数。计算结果表明：若未投入应急冷却系统，反应堆在失去场外电源后热管内MDNBR为0.31，小于1.5的安全限值，会发生偏离核态沸腾，存在烧毁堆芯的风险。应急冷却系统能有效地降低事故后燃料板及冷却剂的温度，提高了系统的安全性。在瞬态条件下，池式研究堆的最大自然循环能力为590 kW，大于设计值200 kW，表明该堆具有良好的固有安全性。
-
图 1 50 keV，500 keV和 5 MeV He辐照Si产生的空位分布
Figure 1. Distributions of vacancies for 50 keV，500 keV and 5 MeV He in Si
表 1 50 keV粒子辐照时，由NRT模型计算得到的移位数
ν Table 1. Number of vacancies calculated by the NRT model at 50 keV ion irradiation
incident ion target Tdam /eV νNRT ratio νNRT(F−C)/νNRT(K−P) K-P F-C K-P F-C proton Si 625 550 7.1 6.3 0.88 proton Fe 955 955 9.6 9.6 1.00 Fe Si 32 660 29 135 373.3 333.0 0.89 Fe Fe 35 165 37 130 351.7 371.3 1.06 Au Si 33 490 30 180 382.7 344.9 0.90 Au Fe 35 805 37 545 358.1 375.5 1.05 
下载: 导出CSV
表 2 500 keV粒子辐照时，由NRT模型计算得到的移位数
ν Table 2. Number of vacancies calculated by the NRT model at 500 keV ion irradiation
incident ion target Tdam/eV νNRT ratio νNRT(F−C)/νNRT(K−P) K-P F-C K-P F-C proton Si 1 150 1 000 13.1 11.4 0.87 proton Fe 1 550 1 550 15.5 15.5 1.00 Fe Si 212 850 181 000 2 432.6 2 068.6 0.85 Fe Fe 258 050 281 400 2 580.5 2 814.0 1.09 Au Si 258 400 218 250 2 953.1 2 494.3 0.84 Au Fe 298 850 326 350 2 988.5 3 263.5 1.09
下载: 导出CSV
表 3 5 MeV粒子辐照时，由NRT模型计算得到的移位数
ν Table 3. Number of vacancies calculated by the NRT model at 5 MeV ion irradiation
incident ion target Tdam/eV νNRT ratio νNRT(F−C)/νNRT(K−P) K-P F-C K-P F-C proton Si 3 500 3 000 40 34.3 0.86 proton Fe 4 000 4 000 40 40 1.00 Fe Si 578 000 485 500 6 605.7 5 548.6 0.84 Fe Fe 799 000 890 000 7 990 8 900 1.11 Au Si 1 388 000 115 000 15 862.9 12 743 0.81 Au Fe 1 836 000 2 065 000 18 360 20 650 1.12
下载: 导出CSV
表 4 50 keV粒子辐照时，由SRIM计算得到的移位数
ν Table 4. Number of vacancies from output of SRIM for 50 keV ion irradiation
incident ion target number of vacancies from “vacancy.txt” ratio νK−P νF−C νF−C/νK−P νK−P/νNRT νF−C/νNRT proton Si 3.6 5.3 1.47 0.50 0.84 proton Fe 4.1 7.7 1.88 0.43 0.81 Fe Si 368 516.4 1.40 0.99 1.55 Fe Fe 347.8 711.7 2.05 0.99 1.92 Au Si 377.9 546.4 1.45 0.99 1.58 Au Fe 355.1 747.4 2.10 0.99 1.99
下载: 导出CSV
表 5 500 keV粒子辐照时，由SRIM计算得到的移位数
ν Table 5. Number of vacancies from output of SRIM for 500 keV ion irradiation
incident ion target number of vacancies from “vacancy.txt” ratio νK−P νF−C νF−C/νK−P νK−P/νNRT νF−C/νNRT proton Si 7.5 11.1 1.48 0.57 0.97 proton Fe 7.7 14.9 1.94 0.50 0.96 Fe Si 2 403.9 3 152.9 1.31 0.99 1.52 Fe Fe 2 559.5 5 230.2 2.04 0.99 1.86 Au Si 2 936.4 3 816.8 1.30 0.99 1.53 Au Fe 2 978.7 6 101.8 2.05 1.00 1.87
下载: 导出CSV
表 6 5 MeV粒子辐照时，由SRIM计算得到的移位数
ν Table 6. Number of vacancies from output of SRIM for 5 MeV ion irradiation
incident ion target number of vacancies from “vacancy.txt” ratio νK−P νF−C νF−C/νK−P νK−P/νNRT νF−C/νNRT proton Si 21.9 32.1 1.47 0.55 0.94 proton Fe 21.8 43.2 1.98 0.55 1.08 Fe Si 6 464.6 5 548.6 1.29 0.98 1.50 Fe Fe 7 863.3 16 315.4 2.07 0.98 1.83 Au Si 15 771.3 19 429.1 1.23 0.99 1.52 Au Fe 18 300.2 38 272 2.09 1.00 1.85
下载: 导出CSV
表 7 He、Li粒子辐照Si，不同方法得到的移位数对比
Table 7. Number of vacancies from different tests for He、Li in Si
incident ion and energy（keV） νNRTfrom Eq.（2） number of displaced atoms from "vacancy.txt" ratio K-P F-C νK−P νF−C νF−C/νK−P νK−P/νNRT νF−C/νNRT He，50 58.9 55.1 45.6 69.4 1.52 0.77 1.26 He，500 85.7 79.4 66 98.7 1.50 0.77 1.24 He，5 000 120.0 114.3 91.7 137.3 1.50 0.76 1.20 Li，50 109.0 101.8 94.7 141 1.49 0.87 1.38 Li，500 189.1 174.9 161.3 236.7 1.47 0.85 1.35 Li，5 000 257.1 234.3 212.2 305.7 1.44 0.83 1.30
下载: 导出CSV
-
[1] Sang P Y. Evaluation of radiation damage induced by a proton beam at the KOMAC facility[J]. J Korean Phys Soc, 2015, 66(3): 439-442. doi: 10.3938/jkps.66.439 [2] Ziegler J F, Biersack J P, Ziegler M D. SRIM—The stopping and range of ions in matter(2010)[J]. Nucl Instrum Methods Phys Res Sect B, 2010, 268(11/12): 1818-1823. [3] Norgett M J, Robinson M T, Torrens I M. A proposed method of calculating displacement dose rates[J]. Nucl Eng Des, 1975, 33(1): 50-54. doi: 10.1016/0029-5493(75)90035-7 [4] Ziegler J F, Biersack J P, Littmark U. The stopping and range of ions in matter[M]. New York: Pergamon, 1985. [5] Stoller R E, Toloczko M B, Was G S, et al. On the use of SRIM for computing radiation damage exposure[J]. Nucl Instrum Methods Phys Res Sect B, 2013, 310: 75-80. doi: 10.1016/j.nimb.2013.05.008 [6] Saha U, Devan K, Ganesan S. A study to compute integrated dpa for neutron and ion irradiation environments using SRIM-2013[J]. J Nucl Mater, 2018, 503: 30-41. doi: 10.1016/j.jnucmat.2018.02.039 [7] Weber W J, Zhang Yanwen. Predicting damage production in monoatomic and multi-elemental targets using stopping and range of ions in matter code: Challenges and recommendations[J]. Curr Opin Solid State Mater Sci, 2019, 23(4): 1-11. [8] Seitz F. On the disordering of solids by action of fast massive particles[J]. Discuss Faraday Soc, 1949, 5: 271-282. doi: 10.1039/df9490500271 [9] Kinchin G H, Pease R S. The displacement of atoms in solids by radiation[J]. Rep Prog Phys, 1955, 18(1): 1-51. doi: 10.1088/0034-4885/18/1/301 [10] Lindhard J, Scharff M, Schiøtt H E. Range concepts and heavy ion ranges (notes on atomic collisions, II) [M].Denmark: I kommission hos Ejnar Munksgaard, 1963. [11] Etherington E W, Bramman J I, Nelson R S, et al. A UKAEA evaluation of displacement damage models for iron[J]. Nucl Eng Des, 1975, 33(1): 82-90. doi: 10.1016/0029-5493(75)90039-4 [12] Robinson M T. Basic physics of radiation damage production[J]. J Nucl Mater, 1994, 216: 1-28. doi: 10.1016/0022-3115(94)90003-5 期刊类型引用(3)
1. 何进，赵士龙，郭凌伟，华有杰，叶仁广，徐时清. 反射式体布拉格光栅设计及其在激光器中的应用研究. 光电子·激光. 2024(02): 122-127 . 
百度学术2. 李奎奎，张伟桥，董风鑫，张建心，周旭彦. 高功率半导体激光叠阵光纤耦合系统与光束整形设计. 中国激光. 2024(17): 98-103 . 百度学术3. 任慧娟. 激光惯性约束下多帧影视图像视觉传达模型构建. 激光杂志. 2023(09): 103-108 . 百度学术其他类型引用(1)
-
下载:
点击查看大图
计量
- 文章访问数: 2820
- HTML全文浏览量: 581
- PDF下载量: 194
- 被引次数: 4
