Simulation of the solenoid scan method used in overlapping field for thermal emittance measurement
-
摘要: 在一种猝发高重频的X射线自由电子激光（XFEL）装置中，由于受到光阴极注入器内补偿螺线管与电子枪之间特殊结构的限制，阴极附近电场与磁场为叠加状态。实验中需要对阴极热发射度进行测量，而测量热发射度常用的螺线管扫描法基于几何发射度不变的前提，无法直接应用于电磁场叠加的结构。针对这一问题，考虑到归一化过程可以剔除电场对发射度的影响，基于此，研究归一化相空间中应用的螺线管扫描法，并通过仿真计算与分析，最终证明该方法适用于电磁叠加场中阴极热发射度的测量。Abstract: In one high repetition frequency X-ray free electron laser (XFEL) equipment, the electron gun and compensate solenoid have special structure in the photoinjector, thus the electric field and the magnetic field overlaps near the cathode. The thermal emittance of the cathode should be measured in the experiment. The solenoid scan method used to measure the thermal emittance is not suitable for the overlapping field, because it works effectively only when the rms emittance keeps constant. As the normalized phase space can avoid the influence of the electric field, we tried to use the solenoid scan method in normalized phase space. Using simulation code to do simulations and analysis, we finally demonstrate that this method is feasible for measurement of thermal emittance of the photoinjector which has overlapping field.
-
Key words:
- thermal emittance /
- solenoid scan method /
- phase space /
- electron gun
-
图 1 阴极附近的电磁场分布与归一化RMS发射度在束团电荷量0.5 pC时的演化
Figure 1. Electric field and magnetic field near the photocathode and normalized RMS emittance evolution for 0.5 pC bunch charge
图 2 在z=1.3 m 与z=2.0 m 处的仿真结果与拟合结果
Figure 2. Simulation data and the curve fitting data at 1.3 m and 2.0 m
表 1 模拟的初始参数
Table 1. Parameters used in the simulation
single bunch charge/pC electric field on cathode/(MV/m) laser pulse length (FWHM)/ps laser RMS spot size/mm transverse distribution (uniform) longitudinal distribution
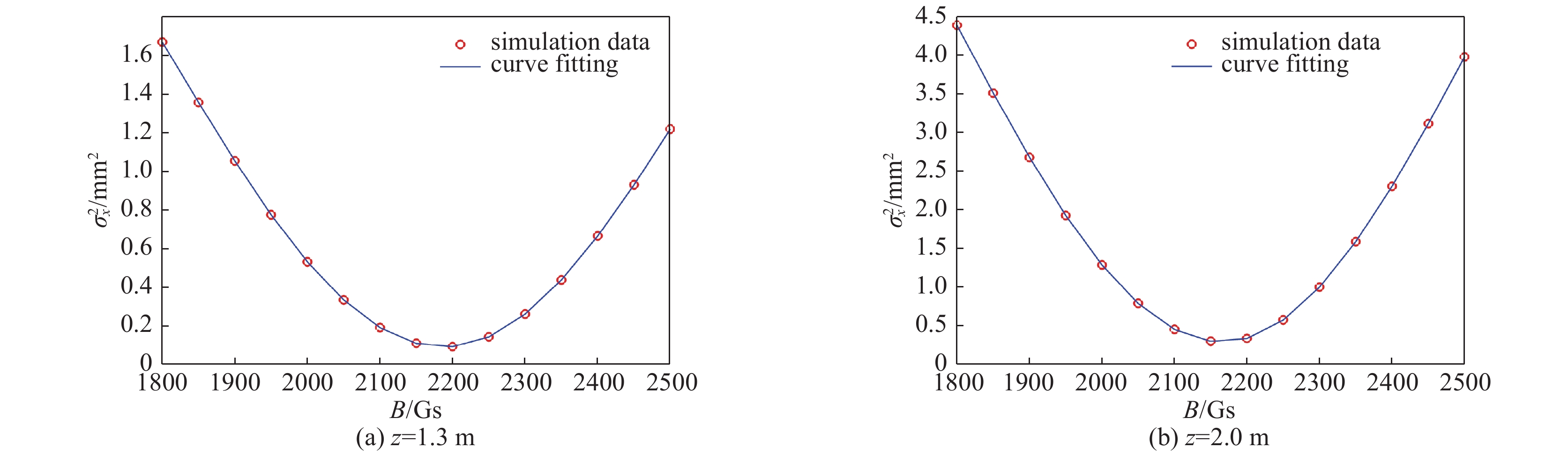
(flat top)0.5 60 20 0.2 − − 
下载: 导出CSV
表 2 ASTRA仿真结果与拟合结果
Table 2. ASTRA simulation results and curve fitting results
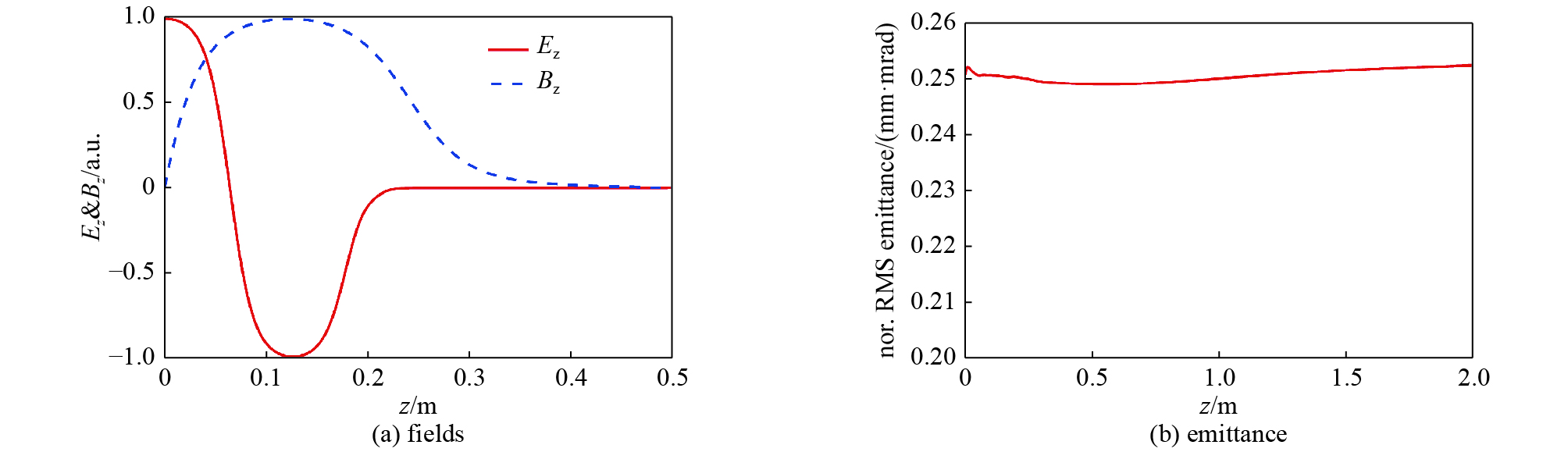
parameter result from ASTRA curve fitting result at 1.3 m curve fitting result at 2.0 m normalized RMS emittance/mm·mrad 0.2503 0.2506 0.2506 normalized $\;{\beta _0}$ 0.1598 0.1597 0.1597
下载: 导出CSV
-
[1] Altarelli M, Brinkmann R, Chergui M, et al. Technical design report of the European X-ray free-electron laser[R]. DESY Report No. 2006-097, 2006. [2] Arthur J, Anfinrud P, Audebert P, et al. LCLS conceptual design[R]. Report No. SLAC-R-593, 2002. [3] Russell S J, Carlsten B E, Duffy L D, et al. MaRIE XFEL pre-conceptual reference design injector[R]. LA-UR-15-21963, 2015. [4] Kwang-Je K I M. RF and space-charge effects in laser-driven RF electron guns[J]. Nuclear Instruments and Methods in Physics Research A, 1989, 275: 201-218. doi: 10.1016/0168-9002(89)90688-8 [5] Carlsten B E. New photoelectic injector design for the Los Alamos National Laboratory XUV FEL accelerator[J]. Nuclear Instruments and Methods in Physics Research A, 1989, 28: 313-319. [6] Luiten O J, van der Geer S B, de Loos M J, et al. How to realize uniform three-dimensional ellipsoidal electron bunches[J]. Phys Rev Lett, 2004, 93: 094802. doi: 10.1103/PhysRevLett.93.094802 [7] Yusof Z M, Conde M E, Wei Gai. Schottky-enabled photoemission in a RF accelerator photoinjector: possible generation of ultralow transverse thermal-emittance electron beam[J]. Phys Rev Lett, 2004, 93: 114801. doi: 10.1103/PhysRevLett.93.114801 [8] Miltchev V. Modelling the transverse phase space and emittance studies at PITZ[C]//Proceedings of the 27th FEL Conference. 2005: 556-559. [9] Anderson S G, Rosenzweig J B. Space-charge effects in high brightness electron beam emittance measurements[J]. Phys Rev ST Accel Beams, 2002, 5: 014201. doi: 10.1103/PhysRevSTAB.5.014201 [10] Kim Y, Andersson A, Dach M, et al. Low thermal emittance measurements at the PSI-XFEL low emittance gun test facility[C]//Proceedings of FEL08. 2008: 110-113. [11] Graves W S, DiMauro L F, Heese R, et al. DUVFEL photoinjector dynamics: measurement and simulation[C]//Proceedings of the 2001 Particle Accelerator Conference. 2001: 2230-2232. [12] Sannibale F, Filippetto D, Cork C, et al. Recent result from the APEX project at LBNL[C]//Proceedings of the PAC.2013: 709-713. [13] Xiang Dao, Du Yingchao, Yan Lixin, et al. Transverse phase space tomography using a solenoid applied to a thermal emittance measurement[J]. Phys Rev ST Accel Beams, 2009, 12: 022801. doi: 10.1103/PhysRevSTAB.12.022801 [14] Chao A W, Tigner M. Handbook of accelerator physics and engineering[M]. 3rd Ed. World Scientific Publishing, 2006: 71-75. [15] Kobayashi S, Nomizu K. Foundations of differential geometry[M]. John Wiley & Sons, 1963. [16] Flottmann K. A space charge tracking algorithm[R]. https://www.desy.de/~mpyflo/Astra_manual/Astra-Manual_V3.2.pdf. -
点击查看大图
图(2) / 表(2)
计量
- 文章访问数: 1300
- HTML全文浏览量: 264
- PDF下载量: 72
- 被引次数: 0
