基于图像识别技术的随机线缆束建模及分布参数统计分析

戎帆; 钟龙权; 刘强; 闫丽萍; 赵翔

doi:10.11884/HPLPB202133.210007

基于图像识别技术的随机线缆束建模及分布参数统计分析

doi: 10.11884/HPLPB202133.210007

戎帆^1,,
钟龙权²,
刘强³,
闫丽萍¹,
赵翔^1, ,

1.
四川大学电子信息学院，成都 610064
2.
中国工程物理研究院应用电子学研究所，四川绵阳 621900
3.
北京应用物理与计算数学研究所，北京 100088

基金项目: 国家自然科学基金面上项目（61877041）

详细信息

作者简介:
戎　帆（1994—），男，硕士，主要从事电磁兼容方面的研究工作

通讯作者:
赵　翔（1973—），女，博士，主要从事电磁效应评估与电磁兼容方面的研究工作

中图分类号: TM152
计量
- 文章访问数: 1030
- HTML全文浏览量: 277
- PDF下载量: 63
- 被引次数: 0
出版历程
- 收稿日期: 2021-01-05
- 修回日期: 2021-03-20
- 网络出版日期: 2021-04-10
- 刊出日期: 2021-05-20

Modeling and statistical analysis of distribution parameters of random cable bundles based on image recognition technology

1.
College of Electronic Information, Sichuan University, Chengdu 610064, China
2.
Institute of Applied Electronics, CAEP, Mianyang 621900, China
3.
Beijing Institute of Applied Physics and Computational Mathematics, Beijing 100088, China

摘要

摘要: 提出了一种对实际弯曲随机捆扎线束的建模方法，该方法首先基于图像识别技术，使用实际线束在侧视和俯视方向的两幅照片来实现弯曲线束轴心三维坐标的重建，然后再基于随机转移路径方法实现弯曲线束的捆扎随机性。基于该建模方法，通过蒙特卡洛模拟来分析弯曲随机线束分布参数的统计特征，发现自电感、互电感和互电容均值沿线变化趋势与线束高度变化趋势一致，自电容均值则趋势相反；自电容、自电感和互电感的变异系数与线束高度存在负相关特征；捆扎随机性不会改变自电感和自电容均值，但是会降低互电容与互电感均值。
- 线束 /
- 随机 /
- 图像识别 /
- 分布参数
Abstract: In this paper, a modeling method of actual bending random bundled wire harness is proposed. Firstly, based on image recognition technology, the three-dimensional coordinates of bending wire harness axis are reconstructed by using two photos of actual wire harness in side view and top view; then the random bundled wire harness is realized based on random transfer path method. Based on this modeling method, this paper analyzes the statistical characteristics of distribution parameters of bending random wire harness by Monte Carlo simulation, and finds that the variation trend of self inductance, mutual inductance and mutual capacitance along the line is consistent with the variation trend of wire harness height, while the trend of self capacitance is opposite; the coefficient of variation of self capacitance, self inductance and mutual inductance has negative correlation with wire harness height; the bundling randomness is not obvious It will change the mean value of self inductance and self capacitance, but reduce the mean value of mutual capacitance and mutual inductance.
- wire-bundles /
- random /
- image recognition /
- distributed parameter

HTML全文

图 1 线束照片与建模效果图

Figure 1. Wiring harness photos and modeling drawings

下载: 全尺寸图片幻灯片

图 2 线束斜视照片与建模效果图

Figure 2. Squint photos and modeling drawings

下载: 全尺寸图片幻灯片

图 3 线束横截面示意图

Figure 3. Schematic diagram of wire harness cross section

下载: 全尺寸图片幻灯片

图 4 一组弯曲随机线束样本建模效果图

Figure 4. Modeling effect picture of a group of bending random harness samples

下载: 全尺寸图片幻灯片

图 5 本文分布参数计算与商业软件结果对比图

Figure 5. The comparison between the results of distribution parameter calculation and commercial software in this paper

下载: 全尺寸图片幻灯片

图 6 一组平直随机线束样本建模效果图

Figure 6. Modeling effect picture of a group of straight random harness samples

下载: 全尺寸图片幻灯片

图 7 弯曲未随机线束分布参数的沿线变化

Figure 7. Variation of distribution parameters of bending non random wire harness along the line

下载: 全尺寸图片幻灯片

图 8 平直随机线束分布参数沿线变化

Figure 8. Variation of distribution parameters of straight random wire harness along the line

下载: 全尺寸图片幻灯片

图 9 弯曲随机线束分布参数沿线变化

Figure 9. Variation of distribution parameters of bending random wire harness along the line

下载: 全尺寸图片幻灯片

图 10 4种线束分布参数均值沿线变化

Figure 10. The variation along the mean value of four kinds of harness distribution parameters

下载: 全尺寸图片幻灯片

参考文献(20)

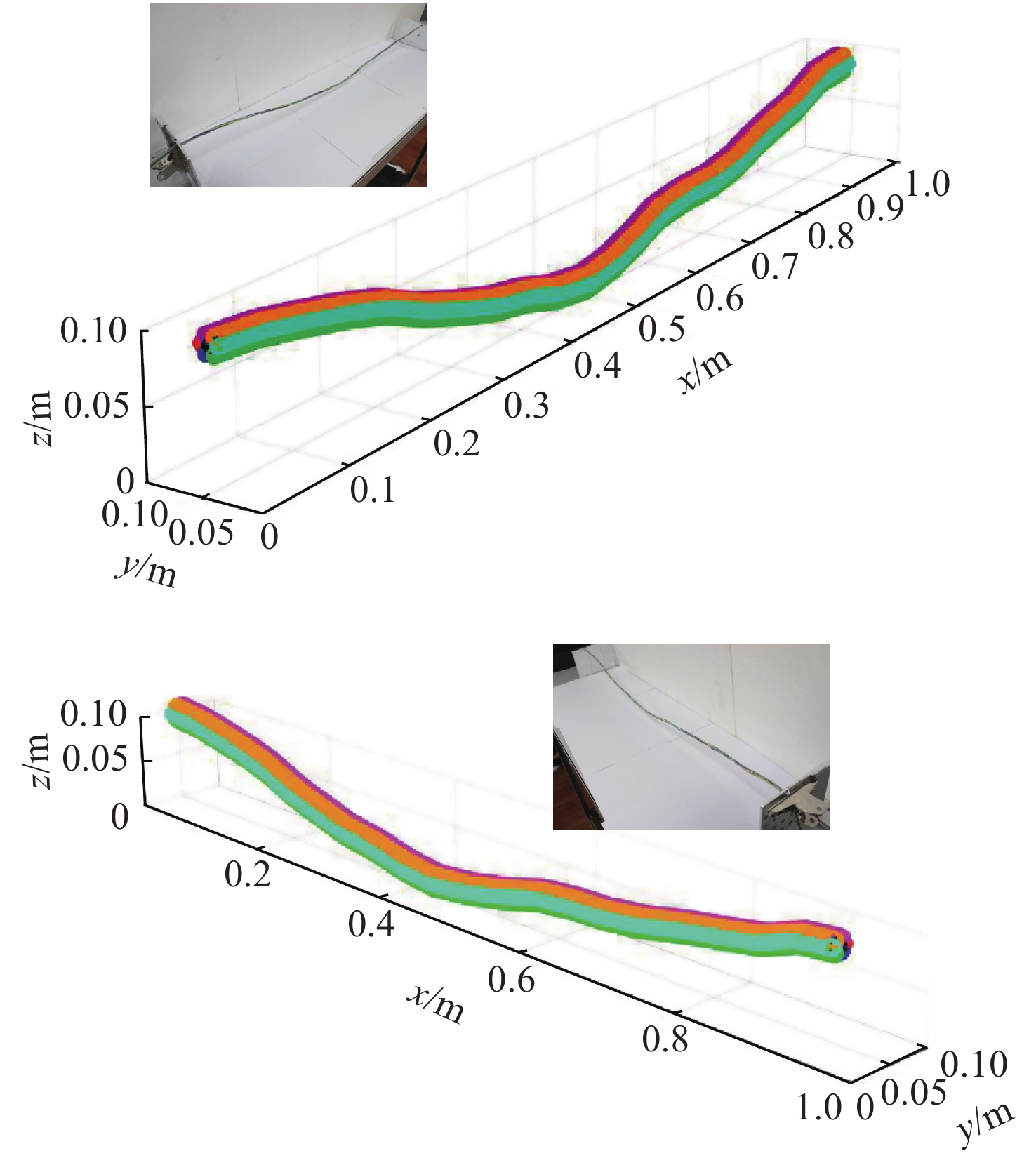
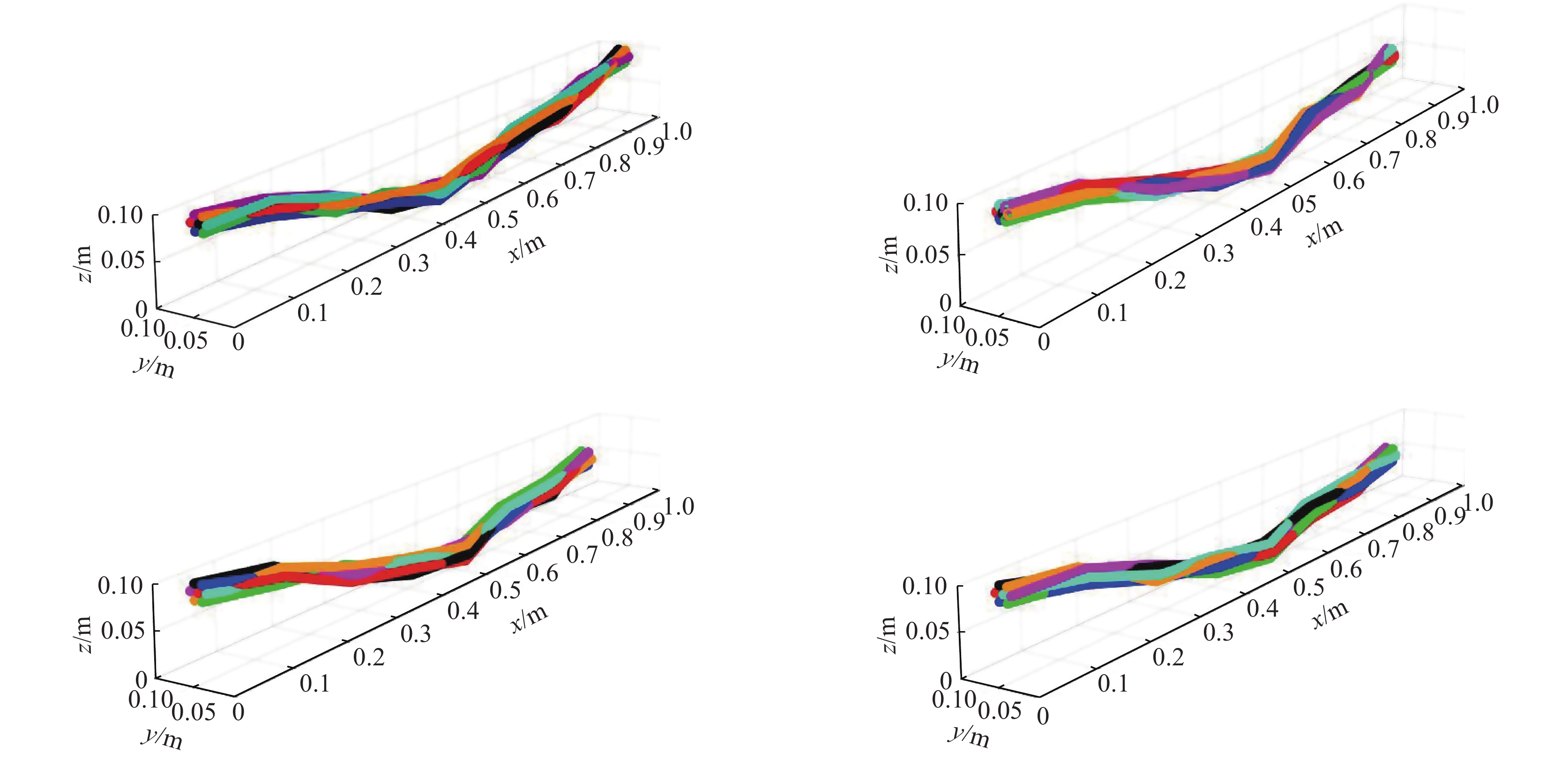
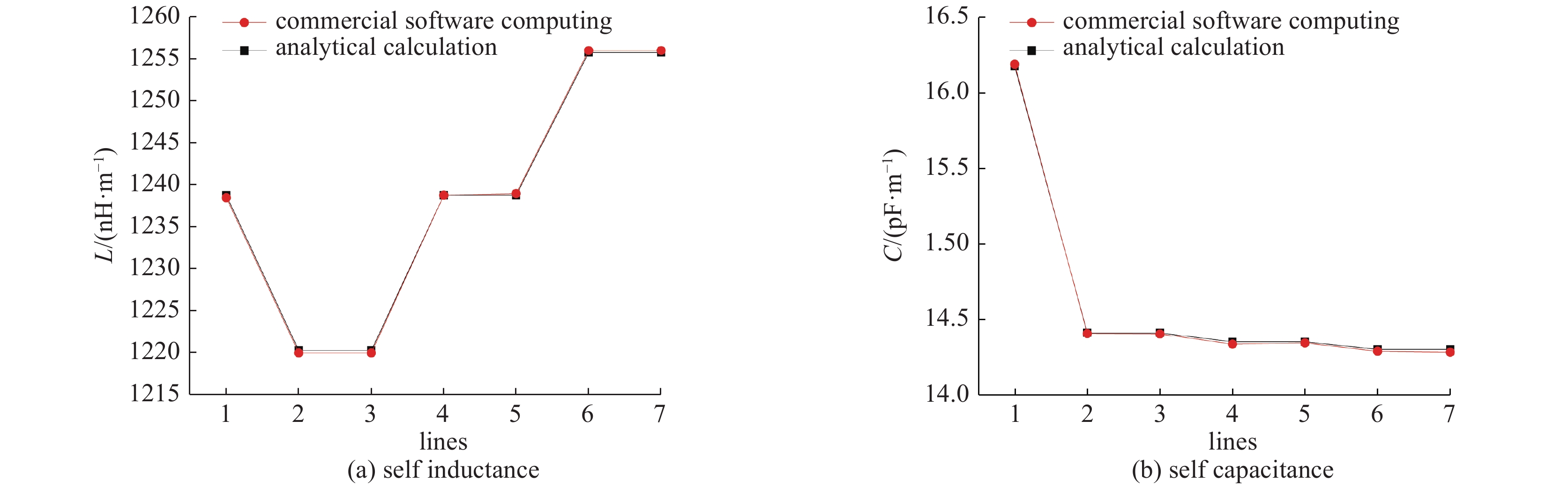
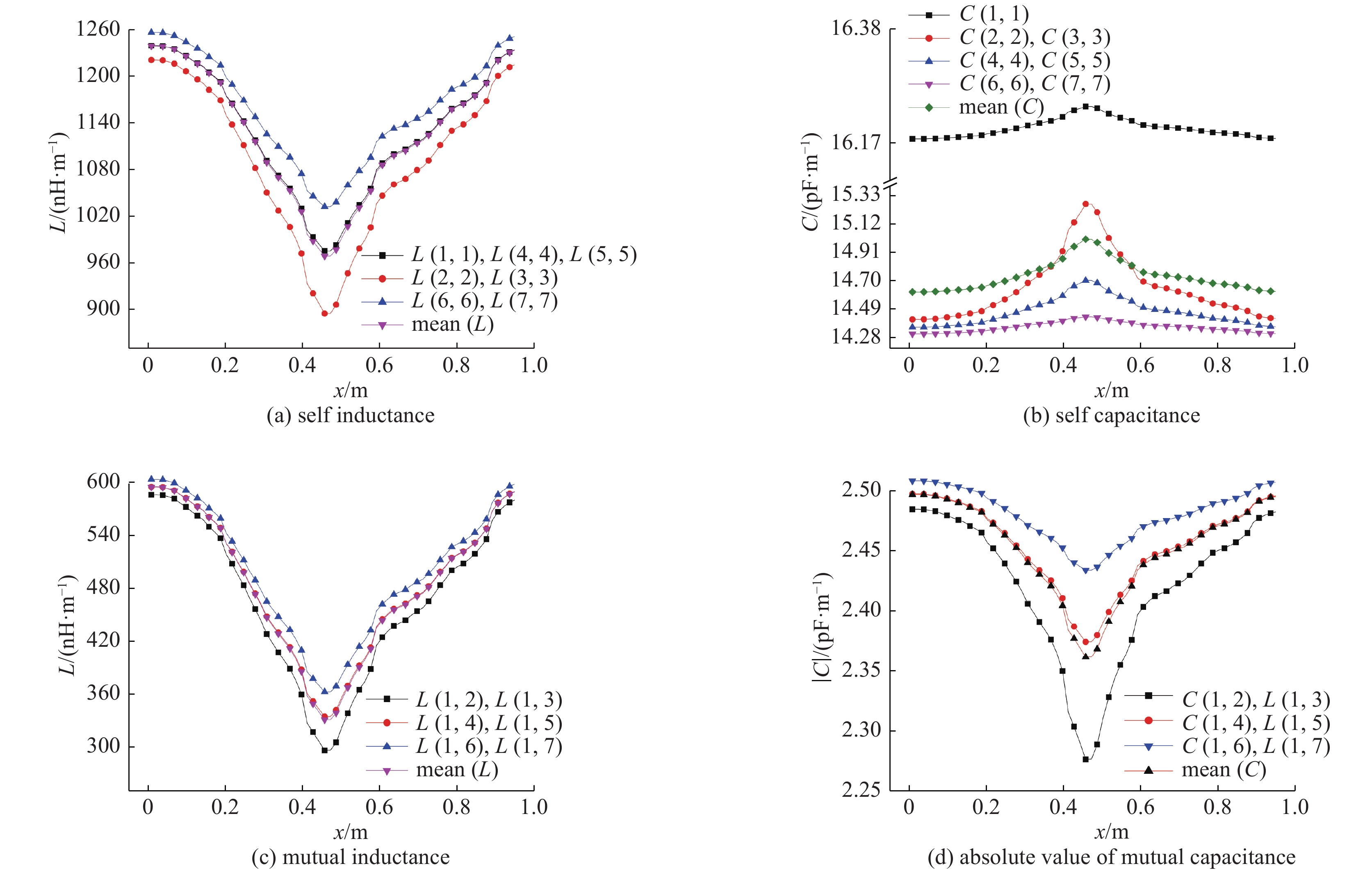
[1]	Ciccolella A, Canavero F G. Stochastic prediction of wire coupling interference[C]//Proceedings of International Symposium on Electromagnetic Compatibility. 1995: 51-56.
[2]	Goodchild M F. Fractals and the accuracy of geographical measures[J]. Journal of the International Association for Mathematical Geology, 1980, 12(2): 85-98. doi: 10.1007/BF01035241
[3]	Salio S, Canavero F, Lefèbvre J, et al. Statistical description of signal propagation on random bundles of wires[C]//Presented at the 13th International Zurich Symposium and Technical Exhibition on Electromagnetic Compatibility. Zurich, Switzerland: EMC, 1999.
[4]	Salio S, Canavero F, Lecointe D, et al. Crosstalk prediction on wire bundles by Kriging approach[C]//Proceedings of the IEEE International Symposium on Electromagnetic Compatibility. Washington: IEEE, 2000.
[5]	Sun Shishuang, Liu Geping, Drewniak J L, et al. Hand-assembled cable bundle modeling for crosstalk and common-mode radiation prediction[J]. IEEE Transactions on Electromagnetic Compatibility, 2007, 49(3): 708-718. doi: 10.1109/TEMC.2007.897142
[6]	Bellan D, Pignari S A. Efficient estimation of crosstalk statistics in random wire bundles with lacing cords[J]. IEEE Transactions on Electromagnetic Compatibility, 2011, 53(1): 209-218. doi: 10.1109/TEMC.2010.2093147
[7]	Spadacini G, Grassi F, Pignari S A. Statistical properties of low frequency voltages induced by a plane-wave field across the terminal loads of a random wire-bundle[C]//Proceedings of 2015 IEEE International Symposium on Electromagnetic Compatibility. Dresden, Germany: IEEE, 2015: 824-829.
[8]	马喜来. 汽车电磁兼容性预估计的研究[D]. 长春: 吉林大学, 2008: 5-12. Ma Xilai. Research on the pre-assessments of electromagnetic compatibility in automobile[D]. Changchun: Jilin University, 2008: 5-12
[9]	王瑞宝, 周诚祥. 船舶线束寄生参数和串扰动态变化估计[J]. 中国修船, 2013, 26(2):34-36, 40. (Wang Ruibao, Zhou Chengxiang. Estimation of parasitic parameters and crosstalk dynamics of ship harness[J]. China Ship Repair, 2013, 26(2): 34-36, 40 doi: 10.3969/j.issn.1001-8328.2013.02.014
[10]	高印寒, 王天皓, 杨开宇, 等. 汽车线束的动态串扰特性预测[J]. 吉林大学学报(工学版), 2014, 44(5):1258-1263. (Gao Yinhan, Wang Tianhao, Yang Kaiyu, et al. Prediction of the dynamic crosstalk of automotive wiring hardness[J]. Journal of Jilin University (Engineering and Technology Edition), 2014, 44(5): 1258-1263
[11]	张昭, 王世山, 赵亮, 等. 多导体线束内串扰概率分布的预测[J]. 电工技术学报, 2017, 32(7):204-214. (Zhang Zhao, Wang Shishan, Zhao Liang, et al. Prediction of crosstalk probability distribution in cable bundles[J]. Transactions of China Electrotechnical Society, 2017, 32(7): 204-214
[12]	Huang Chao, Zhao Yang, Yan Wei, et al. A new method for predicting crosstalk of random cable bundle based on BAS-BP neural network algorithm[J]. IEEE Access, 2020, 8: 20224-20232. doi: 10.1109/ACCESS.2020.2969221
[13]	袁赛, 赵海龙, 曹海丽, 等. 基于MATLAB的带噪图像的中值滤波[J]. 电子世界, 2016(18):185. (Yuan Sai, Zhao Hailong, Cao Haili, et al. Median filtering of noisy image based on MATLAB[J]. Electronics World, 2016(18): 185 doi: 10.3969/j.issn.1003-0522.2016.18.132
[14]	曾建华, 黄时杰. 典型图像边缘检测算子的比较与分析[J]. 河北师范大学学报(自然科学版), 2020, 44(4):295-301. (Zeng Jianhua, Huang Shijie. Comparison and analysis of typical image edge detection operators[J]. Journal of Hebei Normal University (Natural Science Edition), 2020, 44(4): 295-301
[15]	纪执楷, 赵翔, 闫丽萍, 等. 随机线缆束的建模及其场线耦合分析[J]. 无线电工程, 2018, 48(9):788-792. (Ji Zhikai, Zhao Xiang, Yan Liping, et al. Modeling of random wire-bundle and analysis of field-to-line coupling[J]. Radio Engineering, 2018, 48(9): 788-792 doi: 10.3969/j.issn.1003-3106.2018.09.14
[16]	Danielson G. On finding the simple paths and circuits in a graph[J]. IEEE Transactions on Circuit Theory, 1968, 15(3): 294-295. doi: 10.1109/TCT.1968.1082837
[17]	Paul C R. Analysis of multiconductor transmission lines[M]. New York: John Wiley & Sons, Inc., 1994.
[18]	Haase H. Full-wave field interactions of nonuniform transmission lines[D]. Magdeburg: der Otto-von-Guericke-Universität Magdeburg, 2005.
[19]	弗雷德里卡·M·特奇, 米歇尔·V·艾诺茨, 托比杰恩·卡尔松. EMC分析方法与计算模型[M]. 吕英华, 王旭莹, 译. 北京: 北京邮电大学出版社, 2009: 348-350. Tesche F M, Ianoz M V, Karlsson T. EMC analysis methods and computational models[M]. Lü Yinghua, Wang Xuying, trans. Beijing: Beijing University of Posts and Telecommunications Press, 2009: 348-350
[20]	谢处方, 饶克谨. 电磁场与电磁波[M]. 北京: 高等教育出版社, 2003: 67-68. Xie Chufang, Rao Kejin. Electromagnetic field and electromagnetic wave[M]. Beijing: Higher Education Press, 2003: 67-68