Bunch-by-bunch phase measurement and longitudinal instabilities diagnostics at Hefei Light Source
-
摘要: 为了研究储存环束流多束团纵向运动特性，在合肥光源（HLS-II）上研制了一套逐束团相位测量系统。该系统利用示波器直接采集BPM和信号，采用过零点检测法、时间差分（Temporal Difference, TD）法相结合的方法从BPM和信号中提取出逐束团相位。介绍了逐束团相位测量系统的系统构架、相位提取方法以及在HLS-II上的一些实验结果。通过对该系统记录的5 ms时间长度的逐束团多圈相位数据的离线分析，得到多束团纵向运动的同步振荡的频率、纵向工作点、束团振荡的模式信息以及振荡模式增长率等特征信息，诊断出HLS-II在top-off恒流运行期间存在2个较强的纵向耦合束团不稳定模式，并提取出了2个振荡模式的增长率。该系统的逐束团相位测量结果及相关纵向不稳定性分析可为机器研究、纵向反馈系统调试评估及高频RF系统的性能评估等提供参考。Abstract: To study the longitudinal motion characteristics of multi bunches in a storage ring, a bunch-by-bunch phase measurement system is developed at HLS-II. The system uses oscilloscope to collect BPM sum-signal directly, and take zero-crossing detection method combined with temporal difference (TD) method to extract the phase of bunch-by-bunch from BPM sum-signal. The system architecture, phase extraction method and some experimental results at HLS-II are introduced in this paper. In the experiment, through off-line analysis of the phase data of 5 ms bunch-by-bunch multi turns recorded by the system, the characteristic information of the synchrotron oscillation frequency, the longitudinal tuning value, the modes information of bunches oscillation and the growth rate of the oscillation mode are obtained. It is diagnosed that there are two strong longitudinal coupled-bunch unstable modes at HLS-II during the top-off constant-current operation, and the growth rates of the two oscillation modes are extracted. The results of bunch-by-bunch phase measurement and the analysis of longitudinal instability can provide references for machine study, longitudinal feedback system tuning and performance evaluation of high frequency RF system.
-
图 3 过零检测法测量束团相位示意图
Figure 3. Schematic of bunch phase measurement by zero-crossing detection
图 4 单束团多圈相位振荡时域波形及功率谱
Figure 4. Single bunch multi turns time-domain waveform and power spectrum
图 7 逐束团相位测量分辨率分布
Figure 7. Measurement resolution distribution of bunch-by-bunch phase
-
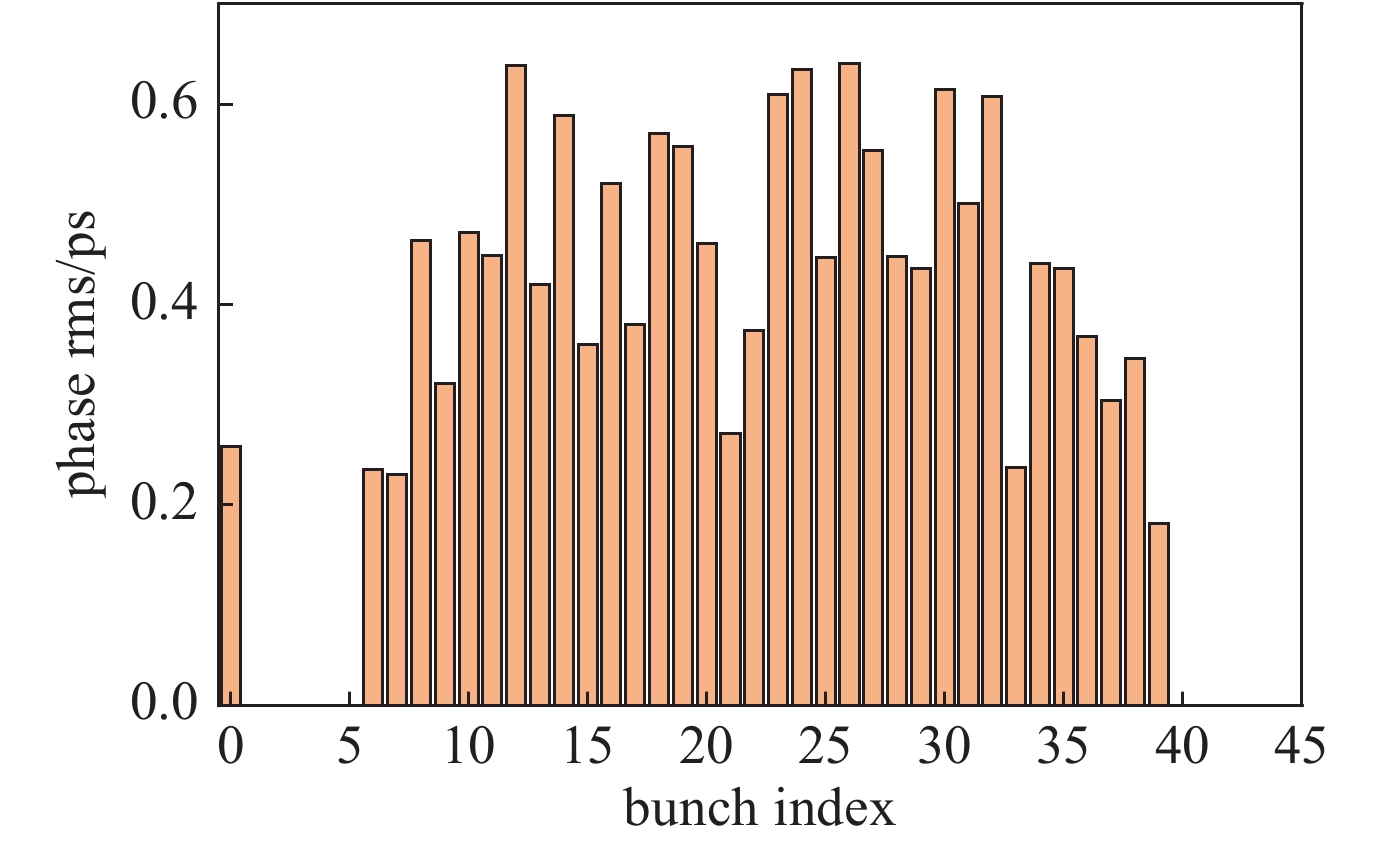
[1] Xu Wei, Jia Dachun, Jiang Shiping, et al. Upgrade project on top-off operation for Hefei Light Source[C]//Proceedings of the 8th International Particle Accelerator Conference. 2017: 2719-2722. [2] Zhou Yimei, Chen Hanjiao, Cao Shanshan, et al. Bunch-by-bunch longitudinal phase monitor at SSRF[J]. Nuclear Science and Techniques, 2018, 29: 113. doi: 10.1007/s41365-018-0445-6 [3] Zhou Yimei, Chen Zhichu, Gao Bo, et al. Bunch-by-bunch phase study of the transient state during injection[J]. Nuclear Instruments and Methods in Physics Research Section A:Accelerators, Spectrometers, Detectors and Associated Equipment, 2020, 955: 163273. doi: 10.1016/j.nima.2019.163273 [4] Fox J, Larsen R, Prabhakar S, et al. Multi-bunch instability diagnostics via digital feedback systems at PEP-II, DAΦNE, ALS and SPEAR[C]//Proceedings of the 1999 Particle Accelerator Conference. 1999: 636-640. [5] Farias R H A, Lin Liu, Rodrigues A R D, et al. Oscilloscope measurement of the synchronous phase shift in an electron storage ring[J]. Physical Review Special Topics—Accelerators and Beams, 2001, 4: 072801. doi: 10.1103/PhysRevSTAB.4.072801 [6] Lee J, Chun M H, Kim G J, et al. Bunch-by-bunch position measurement and analysis at PLS-II[J]. Journal of Synchrotron Radiation, 2017, 24(1): 163-167. doi: 10.1107/S1600577516018154 [7] Yang Yongliang, Ma Tianji, Sun Baogen, et al. HLS bunch current measurement system[J]. Science China: Physics, Mechanics and Astronomy, 2011, 54(2): 309-312. [8] Qin Zengguang, Chen Liang, Bao Xiaoyi. Wavelet denoising method for improving detection performance of distributed vibration sensor[J]. IEEE Photonics Technology Letters, 2012, 24(7): 542-544. doi: 10.1109/LPT.2011.2182643 [9] 郑凯, 王筠华, 刘祖平, 等. 小波分析方法在合肥光源逐束团测量系统中的应用[J]. 强激光与粒子束, 2012, 24(5):1155-1159. (Zheng Kai, Wang Junhua, Liu Zuping, et al. Application of Hilbert-transform-phase-space-reconstruction in HLS bunch-by-bunch system[J]. High Power Laser and Particle Beams, 2012, 24(5): 1155-1159 doi: 10.3788/HPLPB20122405.1155 [10] Daubechies I. Ten lectures on wavelets[M]. Philadelphia: SIAM, 1992: 194-202. [11] Xu Xingyi, Leng Yongbin, Zhou Yimei, et al. Bunch-by-bunch three-dimensional position and charge measurement in a storage ring[J]. Physical Review Accelerators and Beams, 2021, 24: 032802. doi: 10.1103/PhysRevAccelBeams.24.032802 [12] Li W B, Zhou Z R, Sun B G, et al. Digital longitudinal bunch-by-bunch feedback system for the HLS II[C]//Proceedings of IBIC2012. 2012: 434-436. [13] Fox J D, Claus R, Hindi H, et al. Observation, control and modal analysis of longitudinal coupled bunch instabilities in the ALS via a digital feedback system[C]//Proceedings of AIP Conference. 1997: 207-214. [14] 郑凯, 王筠华, 刘祖平, 等. 基于Hilbert变换的相空间重建方法在HLS逐束团测量系统中的应用[J]. 高能物理与核物理, 2007, 31(3):307-310. (Zheng Kai, Wang Junhua, Liu Zuping, et al. Application of Hilbert-transform-phase-space-reconstruction in HLS bunch-by-bunch system[J]. High Energy Physics and Nuclear Physics, 2007, 31(3): 307-310 doi: 10.3321/j.issn:0254-3052.2007.03.018 [15] Prabhakar S, Fox J D, Teytelman D, et al. Phase space tracking of coupled-bunch instabilities[J]. Physical Review Special Topics—Accelerators and Beams, 1999, 2: 084401. doi: 10.1103/PhysRevSTAB.2.084401 -
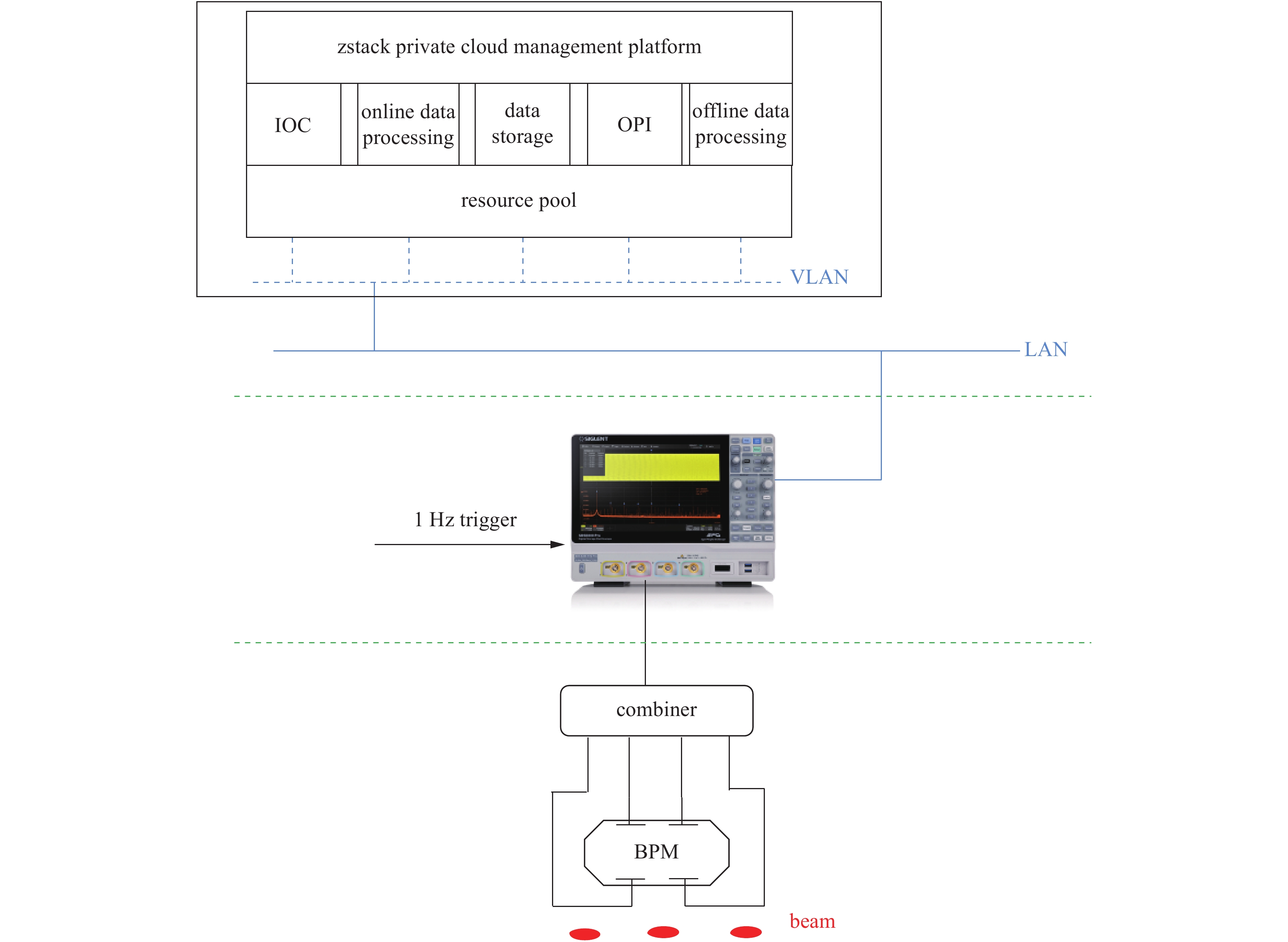
下载:
点击查看大图
图(8)
计量
- 文章访问数: 765
- HTML全文浏览量: 305
- PDF下载量: 50
- 被引次数: 0
