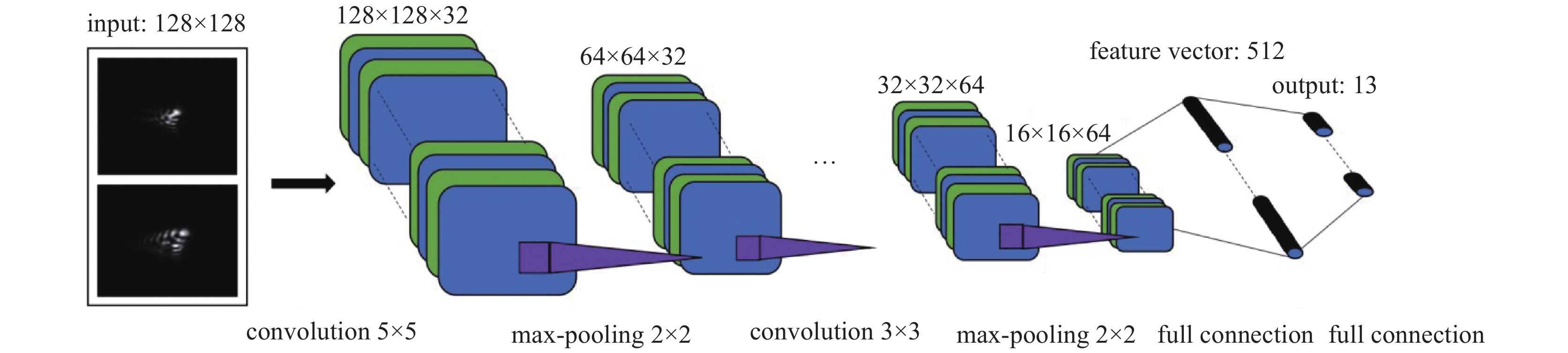
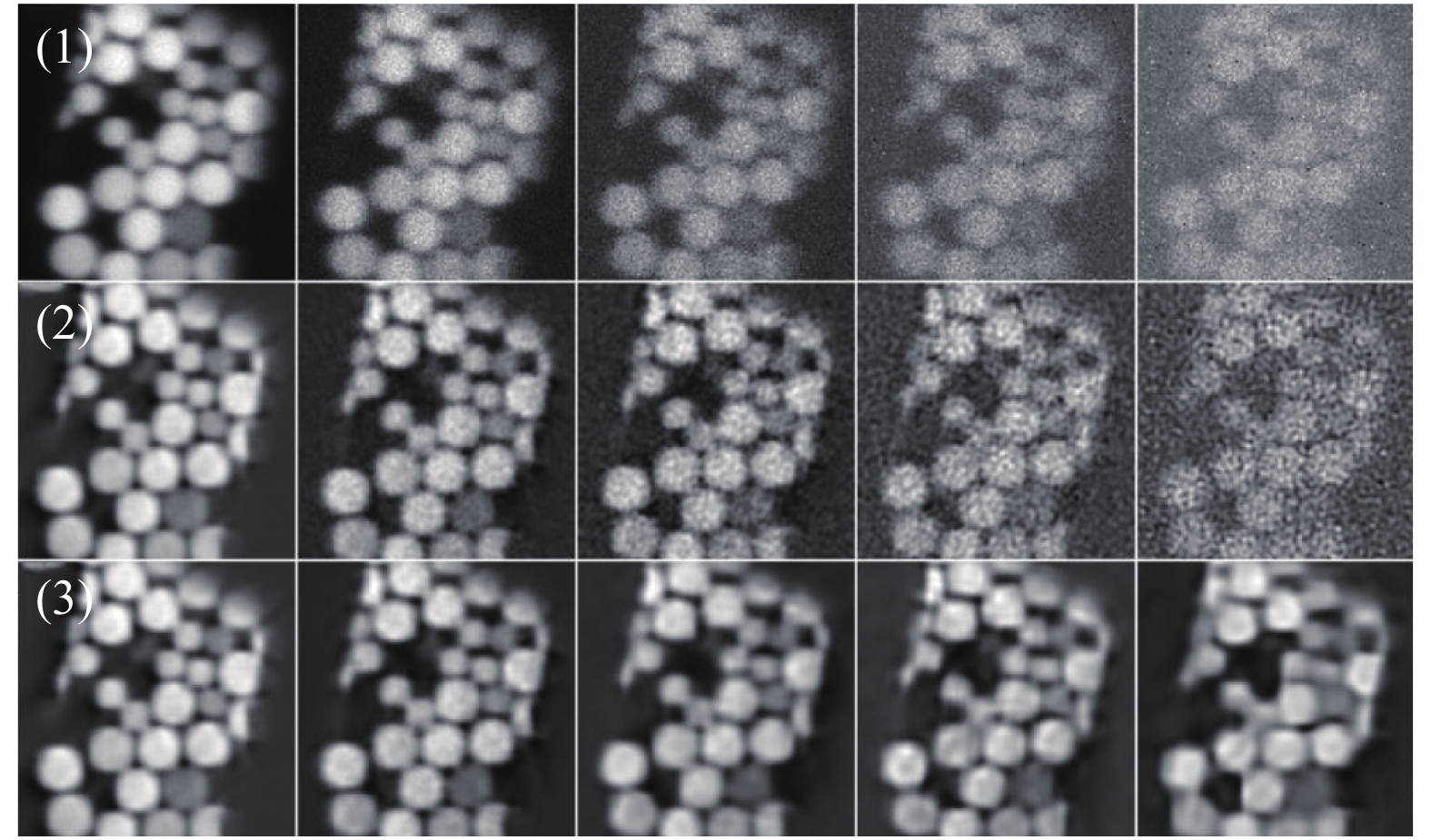
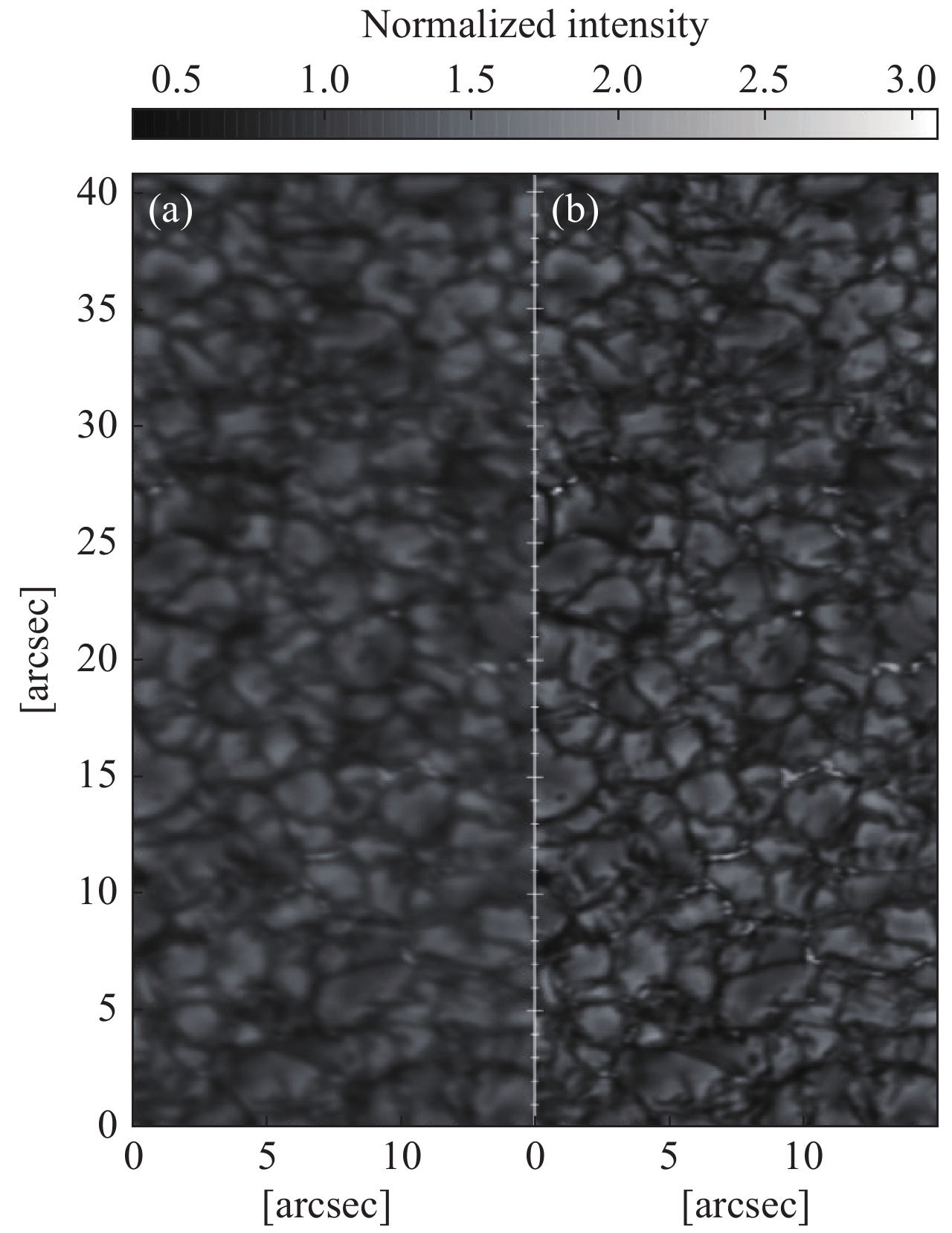
Phase diversity wavefront sensing and image reconstruction
-
摘要: 相位差技术可以直接利用两幅或多幅图像的强度信息，重构出波前相位信息和目标清晰图像，具有光路简单、成本较低、适用于扩展目标等优点，在望远镜的系统像差检测和目标图像重建方面得到了大量应用。相位差波前探测的关键在于求解非线性代价函数的最优化问题，需要避免陷入局部极值并降低计算时间，才能满足动态变化波前实时探测的需求。同时在重建目标清晰图像时，通常需要做正则化和去噪处理，来提高重建图像的质量。本文主要介绍相位差技术的基本原理，以及近年来的研究进展，并对该技术未来的发展进行了展望。Abstract: Phase diversity technology can directly use the intensity information of two or more images to reconstruct the wavefront information and high-resolution image of the target. It has the advantages of simple optical setup, low cost and suitable for extended targets. It has been widely used in system aberration detection and target image reconstruction of telescopes. The key point of phase diversity wavefront sensing is to solve the optimization problem of nonlinear cost function. It needs to avoid falling into local extremum and reduce the calculation time to meet the demand of real-time sensing of dynamic wavefronts. Meanwhile, regularization and denoising are usually needed to improve the quality of reconstructed image. This paper mainly introduces the basic principle of phase diversity technology, as well as the research progresses in recent years, and prospects for future development of this technology.
-
Key words:
- phase diversity /
- wavefront sensing /
- image reconstruction /
- telescopes
-
显示屏是现代电子设备的重要组成部分，是实现人机交互的重要平台，随着新技术的发展，显示屏的应用和使用越来越广泛，其需求不断增长[1]。触摸屏是通过手指触摸的方式进行操作，很容易遇到人体静电放电(ESD)问题。静电放电可以导致显示屏和触摸屏软硬故障，目前针对显示屏静电放电的研究有：使用F65电流钳测量不同类型显示屏瞬态放电电流及其比较[2]；利用铜片对显示屏各个位置的静电放电电流的测量[3]；显示屏静电放电电流全波模型的建立[4]；移动显示驱动芯片的系统级ESD软硬故障研究[5]；液晶显示器(LCD)面板驱动芯片的失效研究[6]；移动设备液晶显示器的静电放电扰乱模型的建立[7]；静电放电软故障诊断方法研究[8]；使用2.5D EM工具对ESD软故障进行调试和近场仿真[9]。
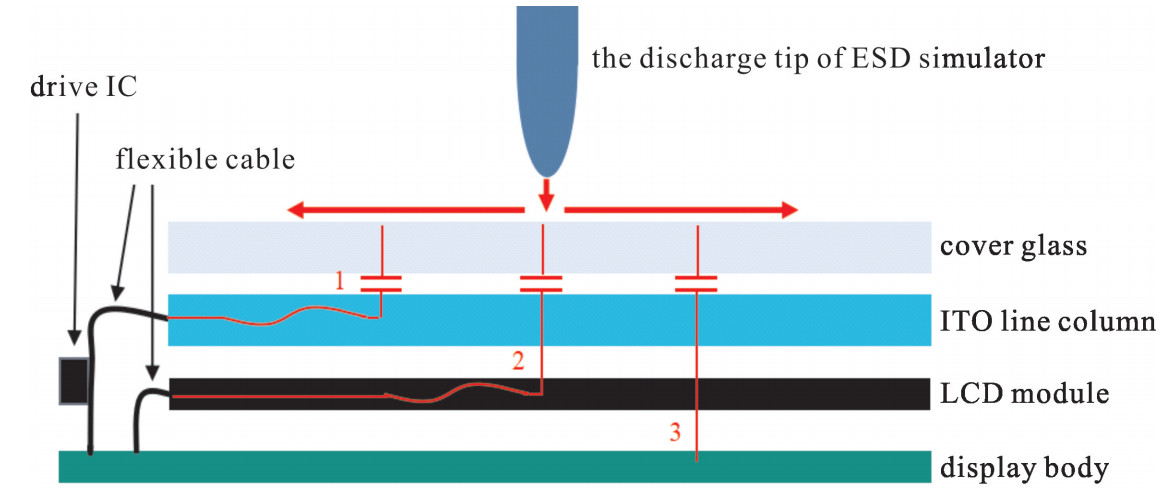
静电放电火花不能穿透玻璃，然而，静电放电和显示屏及其驱动电路之间存在着各种各样的通道和耦合路径[3]。如果显示屏没有良好的隔热结构，放电火花就可能会到达连接显示屏和主板的柔性电缆。如果表面玻璃与显示层之间边缘封装结构受到电击穿，火花就可以进入显示屏。放电火花还可以到达其他金属结构，如系统框架[4]。特别是当火花到达柔性电缆或玻璃层之间的封装电路中时，会损坏或扰乱显示屏。然而，许多显示屏设计都由绝缘结构包围显示屏，这样静电放电就不能直接放电到接地结构。对于设计完好的显示屏，ESD测试人员如果在空气放电模式下使用ESD发生器进行放电，将不会观察到任何火花。未观察到火花往往会误以为没有电流。ESD发生器放电尖端靠近显示屏表面时将引起表面充电，在＜1 ns上升时间内达到10 A电流[2]。显示屏上的非火花放电电流会在显示屏表面玻璃上向各个方向扩散，并以位移电流的形式通过玻璃层，进入显示屏内部。放电火花还可以在显示屏边缘的表面传播，到达柔性电缆或穿透用来连接显示屏不同结构单元的胶水，到达显示器的内部结构，并破坏这些结构。在设计完好的系统中，绝缘屏障良好，火花不能在显示器的边缘传播。因此，最可能扰乱或损坏显示屏的路径是通过玻璃的位移电流。
无火花放电在显示屏表面玻璃产生的电荷可以用Lichtenberg粉尘图法可视化。正极性电压放电的粉尘图为分支扩展结构；负极性电压放电的粉尘图为密度更大的圆形结构，而不是分支结构[10]。虽然粉尘图能够在放电后使电荷分布可视化，但却不能分析电极周围的瞬态位移电流。为了测量电荷密度随时间的变化与分布，Mueller设计了一种利用分段地平面来测量局部电流的装置[11]。基于相同的概念，同时为了使测量速度更快，本文工作中使用了类似的分段地平面来捕捉位移电流和电荷分布。
本文主要对显示屏进行空气式静电放电实验，通过一个自制装置捕获通过显示屏表面玻璃的位移电流，研究了放电电流、位移电流和电荷密度及其分布的主要特性，同时研究了不同放电电压、不同放电极性以及不同ESD发生器接近速度对放电电流和位移电流的影响。
1. 实验装置与测试方案设计
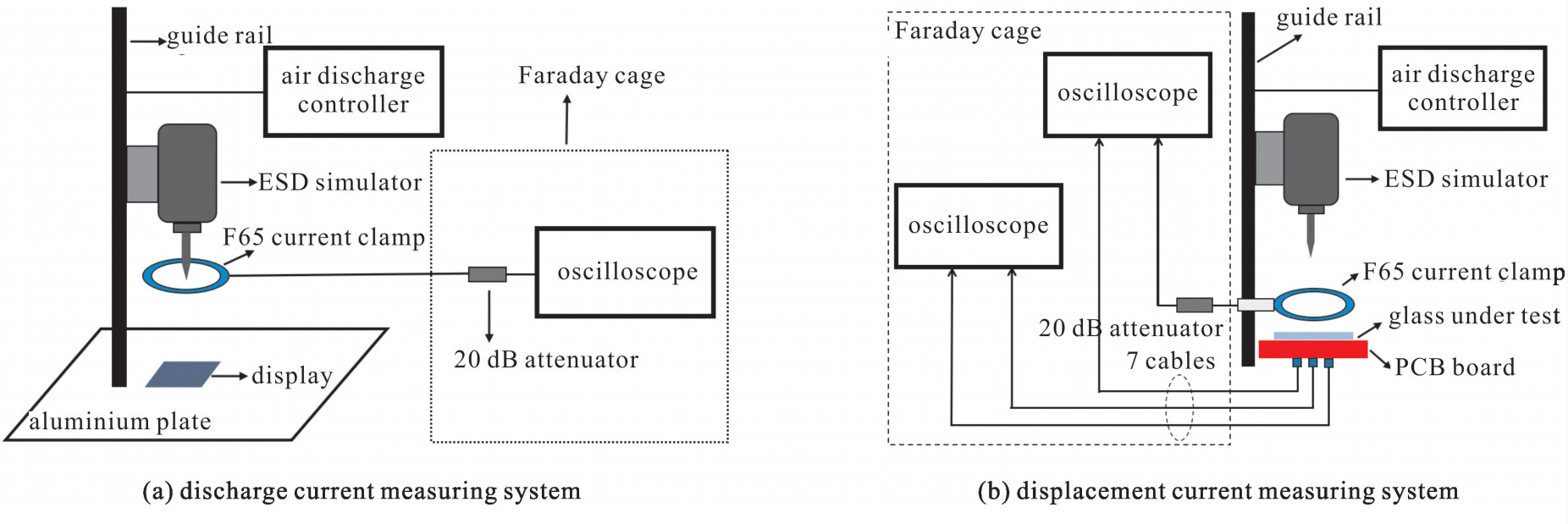
实验分为两部分，显示屏空气式静电放电放电电流与表面玻璃位移电流的测量，测量系统如图 1所示。测量系统主要包括：ESD模拟器、ESD空气式静电放电速度控制器、采样率5 GS/s和带宽2 GHz示波器、20 dB衰减器、法拉第笼。空气式静电放电速度控制器由空气放电控制监测仪和导轨组成。ESD模拟器由特制的夹具固定于导轨上，由空气放电控制监测仪控制上下移动，速度0.05~0.5 m/s可调。F-65电流钳置于ESD模拟器夹具之中，位于ESD模拟器放电尖端处。测量系统整体放置在标准静电放电抗扰度测试平台上。法拉第笼、示波器接地。图 1(a)为放电电流测量系统，放电电流使用F-65电流钳测量，显示屏放置于铝板上。图 1(b)为位移电流测量系统，为了测量通过表面玻璃的位移电流，设计了一块PCB板。
在玻璃表面上扩散的放电电流会导致玻璃表面电位的快速变化。这种快速的电位变化将使位移电流通过电容扩散到显示器的内部结构，例如触摸层或显示层。无火花的电流路径如图 2所示。此外，火花还可能发生在ESD发生器的放电尖端与显示器的内部结构之间，例如柔性电缆。在设计完好的系统中，显示屏边缘的绝缘屏障可以防止出现这种类型的火花。
图 2 无火花表面电晕电流和通过显示器的位移电流Figure 2. Sparkless surface corona current and displacement current paths into the displayESD模拟器对显示屏放电后，显示屏表面的静电放电电流从放电点沿着显示屏表面玻璃向四周扩散，形成位移电流通过玻璃到达下一层。为了测量通过表面玻璃的位移电流，设计了一块PCB板，如图 3所示。PCB板的正面有8个排成一排的贴片天线，尺寸为3 mm ×3 mm。贴片天线的周围为参考地平面。每个贴片天线通过中间位置一个尺寸很小的过孔与PCB板背面的微带线相连。测量位移电流时，将显示屏表面玻璃层紧紧按压在PCB板的正面，使玻璃与PCB板之间的缝隙尽量小。ESD模拟器对表面玻璃放电后，放电电流在玻璃表面向四周扩散，形成位移电流通过玻璃，PCB板上的贴片天线感应到位移电流。然后经过导线、衰减器连接到示波器通道，实现位移电流的采集。
实验之前先校准ESD模拟器、电流钳和衰减器，实验时湿度为40%，温度为25 ℃。ESD模拟器选择人体金属模型和空气式放电模式，在不同放电电压水平、极性(±4 kV，±6 kV，±8 kV，±10 kV和±12 kV)以及不同放电电极接近速度(慢速：0.1 m/s，中速：0.3 m/s，快速：0.5 m/s)条件下分别进行放电实验，记录放电电流波形和位移电流的特征值并通过示波器保存波形数据。
2. 实验结果与讨论
2.1 放电电流
有大量学者研究了空气式静电放电特性，实验中通常是采用ESD模拟器对电流靶进行放电，其实验结果表明，不同放电电压、不同极性、不同接近速度条件下，放电电流峰值和上升时间差距较大[12-13]。本文中，研究了ESD模拟器对显示屏放电时，不同放电电压、不同放电极性以及不同ESD模拟器接近速度对放电结果的影响。
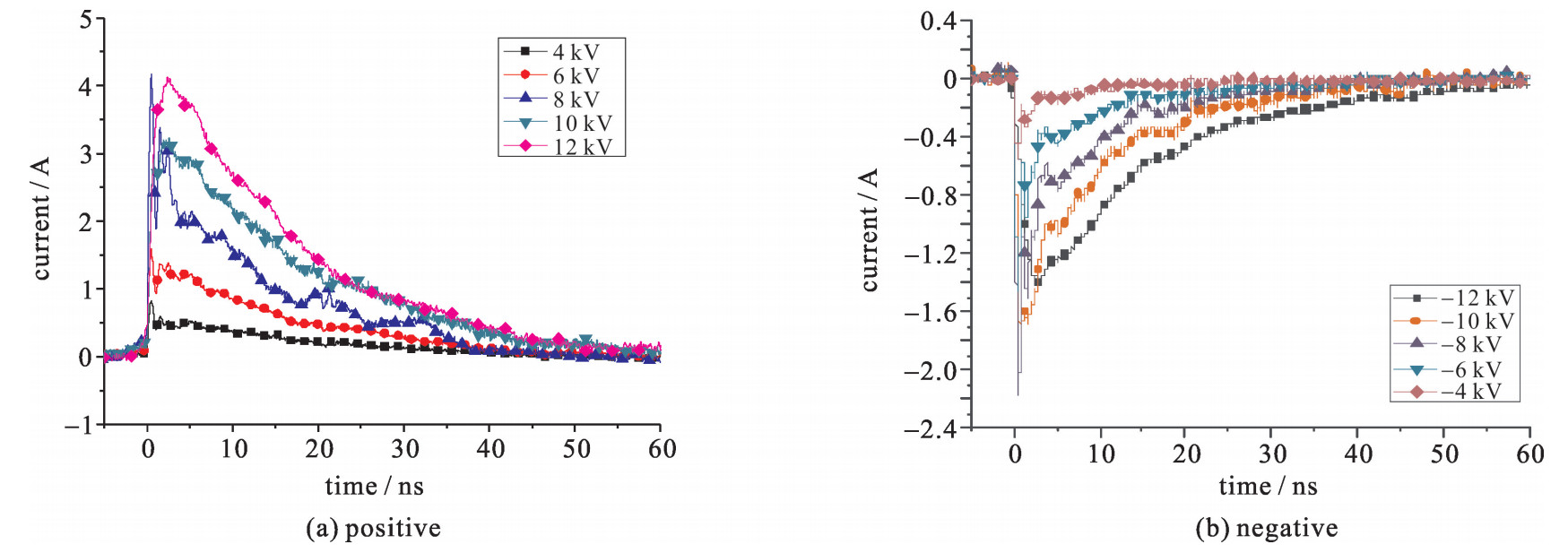
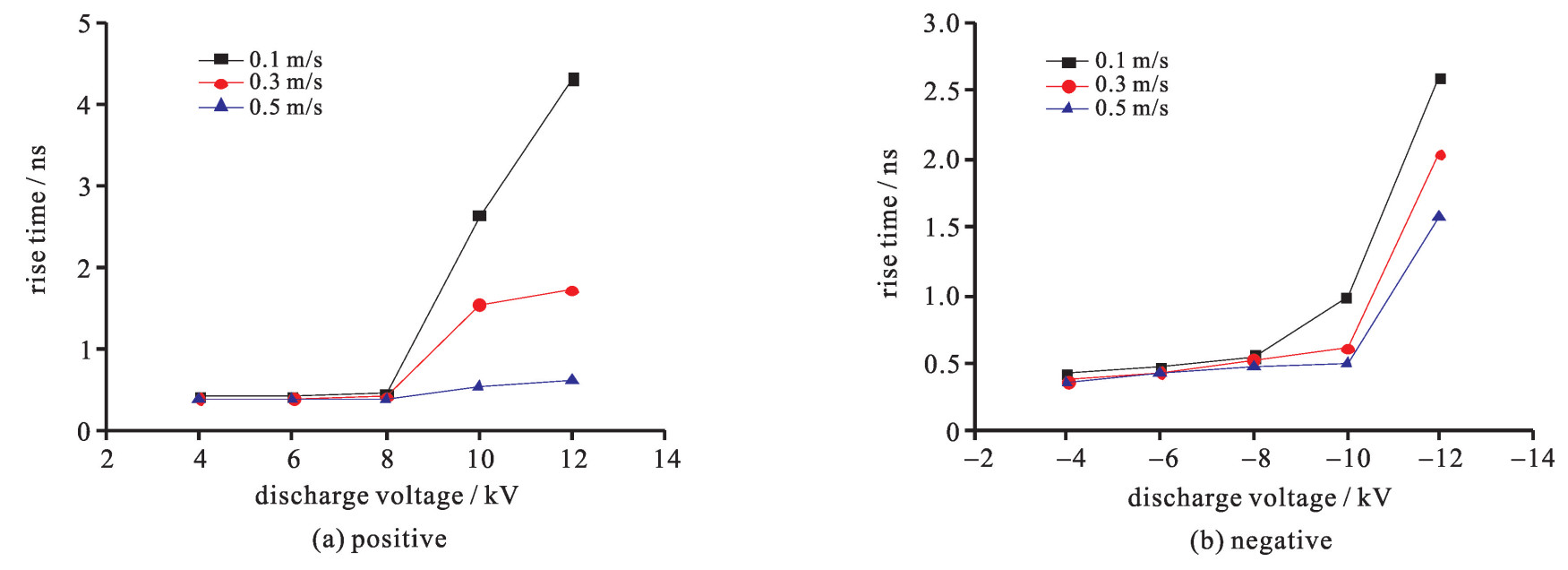
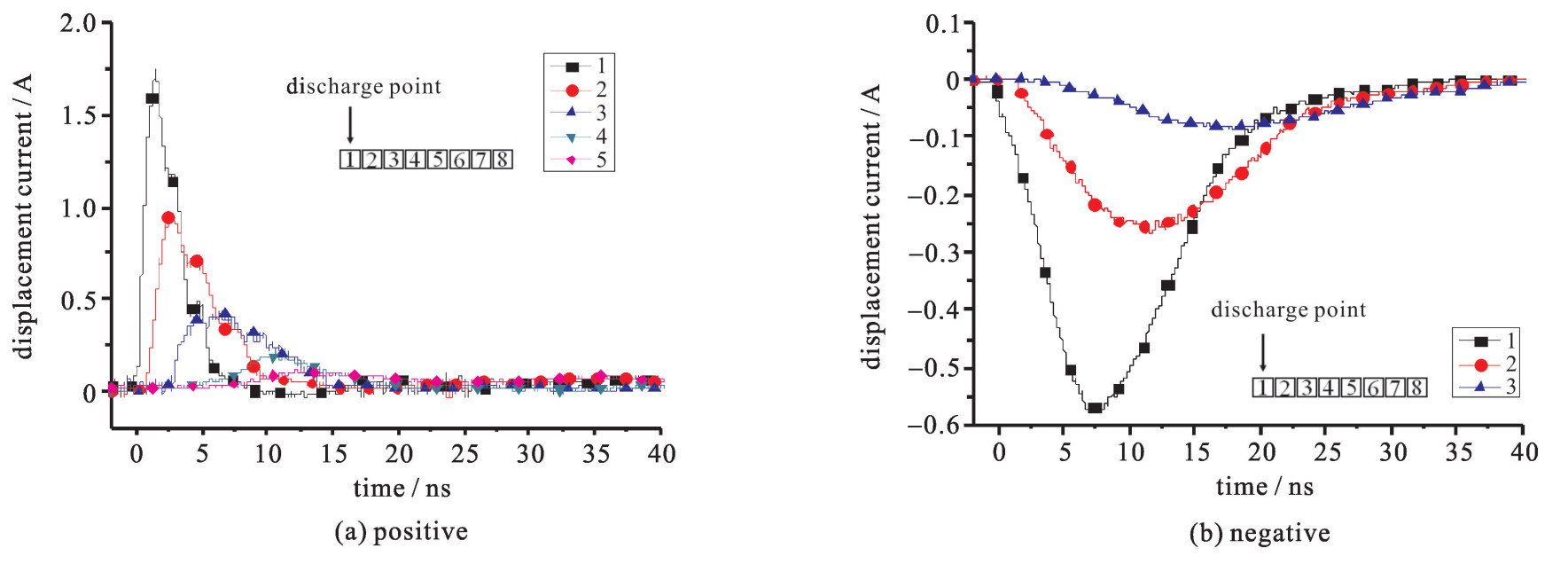
图 4给出的是ESD模拟器对显示屏表面的放电电流，ESD模拟器接近速度为0.3 m/s。在放电电压为±2 kV时，电流钳没有捕捉到电流波形，所以图中放电电压从±4 kV开始。显然，放电电流峰值并不总是随放电电压的增大而增大，上升时间也不总是在小范围内波动。为了研究不同放电电压、不同放电极性和不同接近速度对放电电流波形的影响，在不同条件下进行放电实验。图 5、图 6分别为不同放电电压、不同放电极性和不同接近速度的放电电流波形的电流峰值与上升时间。放电电流峰值随接近速度的增加而增加，上升时间随接近速度的增加而减小。在±4~±8 kV，电流峰值随放电电压的增加而增加，而在±10~±12 kV，电流峰值随放电电压的增加而减小；上升时间随放电电压的增加而增大。
上述现象出现原因是电弧长度的影响。放电电压较低时，电弧长度很短，而在放电电压较高时电弧长度较长[12]。放电电压相同时，放电能量相同，当电弧长度较长时，上升时间增加，能量在更长时间释放，则电流峰值减小；同时电晕也会造成部分能量的损耗，导致放电电流波形总能量减少，电流峰值随之减小。接近速度会影响统计时间延迟，进而会影响到电弧长度[13]，放电电压一定时，接近速度越快，在相同时间延迟内电极移动距离越长，电弧长度越短，放电能量释放越集中，则放电电流峰值越大，上升时间越小。
2.2 位移电流
在设计完好的显示系统中，绝缘屏障良好，火花不能在显示器的边缘传播。因此，最可能扰乱或损坏显示屏的路径是通过玻璃的位移电流。为了捕捉电流分布，设计了一个PCB板，其中包含8个3 mm×3 mm的贴片天线，贴片天线的周围为参考地平面，如图 3所示。PCB板安装在玻璃背面。
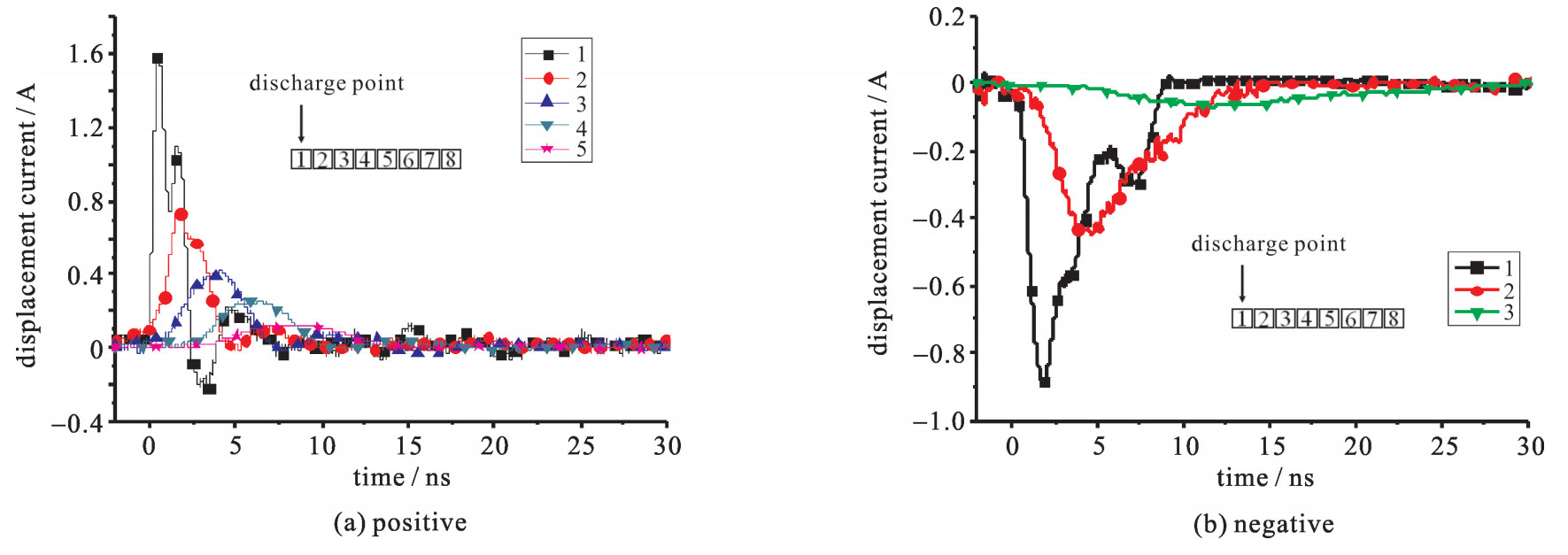
将一块显示屏表层玻璃紧紧贴在位移电流测量PCB板上，调整ESD模拟器放电尖端位置，使放电尖端与PCB板上的贴片天线#1的中心位置重合。静电放电发生器的电压设置为±8 kV，该值是ESD测试典型的电压等级。相对湿度控制在40%左右，位于ESD测试要求的范围(30%~60%)内。为获得位移电流风险评估的上限，ESD模拟器的接近速度设为快速0.5 m/s，如图 7所示。由2.1小节可知，在±10-±12 kV放电电压，电流峰值随放电电压的增大而减小，于是列出了放电电压±12 kV、接近速度0.5 m/s的位移电流，如图 8。
为了更直观的了解位移电流，从各贴片捕获的位移电流波形中提取出电流峰值与上升时间，如表 1、表 2所示。由表 1可知，位移电流波形的峰值随测量点与放电点之间距离的增大而减小，相对于负极性放电，正极性位移电流峰值更大且扩散范围更广。由表 2可知，位移电流上升时间随测量点与放电点之间距离的增大而增大，负极性位移电流上升时间增大更加明显。由于玻璃是绝缘体，ESD模拟器对显示屏表面玻璃放电后，电荷在玻璃表面向四周扩散，并残留在玻璃表面，同时随着扩散距离的增加，扩散面积增大，电荷分散，因此位移电流峰值随距离的增加而减小。
表 1 位移电流波形电流峰值Table 1. Peak of displacement current waveformdischarge voltage/kV total peak current/A peak current/A patch 1 (discharge center) patch 2 patch 3 patch 4 patch 5 8 5.57 1.59 0.74 0.43 0.26 0.12 -8 -2.43 -0.89 -0.46 -0.07 — — 12 5.40 1.75 0.96 0.43 0.19 0.11 -12 -1.35 -0.57 -0.21 -0.07 — — 表 2 位移电流波形上升时间Table 2. Rise time of displacement current waveformdischarge voltage/kV rise time of total current/ns rise time/ns patch1 (discharge center) patch2 patch3 patch 4 patch5 8 0.41 0.46 1.67 3.78 4.05 4.27 -8 0.34 0.69 2.08 9.24 — — 12 0.62 0.95 1.27 1.67 4.40 6.57 -12 1.57 4.08 4.58 7.39 — — 2.3 电荷密度
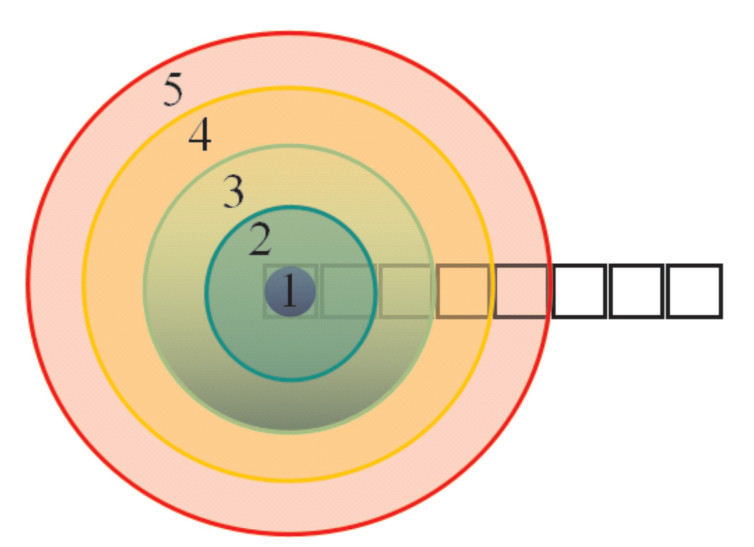
2.2小节中的结果显示，相同放电电压的正、负极性放电电流峰值差异较大，负极性放电时电流峰值和放电能量虽然较小，但其影响的范围也更小。因此，仅考虑放电电流是不妥当的。通过2.2小节中测量的位移电流，对静电放电的电荷密度进行分析计算。电荷密度与位移电流有关，是一种测量局部干扰触点或显示层风险的方法，比放电电流更能说明静电放电对显示屏局部区域的损伤风险。由文献[10]可知，粉尘图的形状以放电点为中心呈对称形状，因此根据粉尘图的对称特性，可以认为与放电点距离相等的圆环上，放电电荷密度近似相等。因此可以通过贴片上测量的位移电流和贴片相关的环面区域来计算电荷密度，测量图形如图 9所示。放电点位于贴片天线#1中心的正上方，对应图 9中编号为1的方格。以贴片天线的边长为环径，将放电区域划分为1，2，3，4，5五个区域，1区域为贴片天线#1所处的圆，圆半径为1.5 mm。2~5区域为环径为3 mm(大小为贴片天线尺寸)的同心圆环，环心为放电点。
各区域电荷密度计算公式为
σ=∫I dtπr2−π(r−3)2 (1) 式中：I为各区域对应的贴片天线所测位移电流；r为圆环外半径。例如，对于区域2，r = 4.5 mm。由式(1)结合图 7、图 8所示的位移电流测量波形，分别计算放电电压为±8 kV, ±12 kV时显示屏各区域的放电电荷密度，结果见表 3。
表 3 不同的贴片和极性的表面电荷密度(单位: nC/cm2)Table 3. Surface charge densities for different patches and polarities(units: nC/cm2)discharge voltage/kV center circle (discharge center) annulus 2 annulus 3 annulus 4 annulus 5 8 47.06 6.05 2.46 1.2 0.67 -8 59.78 5.06 0.71 — — 12 75.73 8.60 2.93 1.06 0.45 -12 94.77 7.00 1.42 — — 电荷密度的这种计算方法是基于位移电流的测量结果而得，比放电电流更能说明静电放电对显示屏局部区域的损伤风险。从表 3的结果中可以看出，静电放电的电荷密度随着距放电点位置的增大而减小。在相同放电条件下，虽然正极性的放电电流峰值比负极性大(+8 kV，+12 kV时分别为5.57 A，5.40 A，-8 kV，-12 kV时分别为-2.43 A，-1.35 A)，但是负极性放电在放电点处的电荷密度比正极性的结果高。从结果中还可以看出，负极性放电的电荷密度消减速度比正极性要快得多。这说明负极性放电具有造成更高等级损伤风险的危害。虽然±12 kV时放电电流峰值小于±8 kV，但是电流密度更大，因此危害更大。
3. 结论
本文通过自主设计的装置测量了对显示屏表面玻璃空气式静电放电的放电电流和通过显示屏的位移电流，研究了不同放电电压、不同放电极性和不同ESD模拟器接近速度对放电结果的影响；基于位移电流的测量结果，计算出显示屏上非火花放电的电荷密度。
放电电流峰值随接近速度的增加而增加，上升时间随接近速度的增加而减小。在±10~±12 kV放电电压，电流峰值随放电电压的增加而减小；上升时间随放电电压的增加而增大。其原因是电弧长度的影响。放电电压较低时，电弧长度很短，而在放电电压较高时，电弧长度较长。接近速度会影响电弧长度，接近速度越快，电弧长度越短。当电弧长度较长时，上升时间增加，电流峰值减小。
位移电流与放电电流有关，位移电流波形的峰值随测量点与放电点之间距离的增大而减小；位移电流波形的上升时间随测量点与放电点之间距离的增大而增大。正极性位移电流峰值更大且扩散范围更广，而负极性位移电流上升沿时间增大更加明显。
电荷密度比放电电流更能说明静电放电对显示屏局部区域的损伤风险。电荷密度随着距放电点位置的增大而减小。与正极性放电相比，尽管负极性放电电流峰值较低，但电荷密度较高，说明负极性放电具有造成更高等级损伤风险的危害。同样的，虽然±12 kV时放电电流峰值小于±8 kV，但是电流密度更大，因此危害更大。
-
图 1 离焦作为相位差函数的PD技术示意图
Figure 1. Schematic diagram of PD technology with defocus as the phase diversity function
图 2 代价函数具有单个极值（a）和多个极值（b）的示意图
Figure 2. Schematic diagram of cost function with single extremum (a) and multiple extremum (b)
图 3 三种不同波前幅值的实验结果：从左至右分别为焦面图像、离焦面图像、重建相位和重建图像
Figure 3. Experimental results of three different wavefront amplitudes: (from left to right) focal plane images, defocus plane images, reconstructed phases and reconstructed images
图 5 基于LSTM网络的相位差波前探测
Figure 5. Phase diversity wavefront sensing based on LSTM network
图 6 基于卷积神经网络的相位差波前探测
Figure 6. Phase diversity wavefront sensing based on convolution neural network
图 7 第一行：焦面图像；第二行：未去噪重建图像；第三行：去噪后重建图像
Figure 7. First line: focal plane images; Second line: reconstructed images without denoising; Third line: reconstructed images after denoising
图 8 PD标定NFIRAOS系统非共光路像差
Figure 8. Calibration of non-common path aberrations in NFIRAOS system with PD technology
图 9 太阳观测结果：左-PD恢复前；右-PD恢复后
Figure 9. Solar observation data: (left) unreconstructed image; (right) PD reconstructed image
-
[1] 张逸新, 迟泽英. 光波在大气中的传播与成像[M]. 北京: 国防工业出版社, 1997.Zhang Yixin, Chi Zeying. Propagation and imaging of light waves in atmosphere[M]. Beijing: National Defense Industry Press, 1997. [2] 杨磊. Phase diversity波前重构的研究及在高分辨图像复原中的应用[D]. 云南: 中国科学院研究生院云南天文台, 2007.Yang Lei. Researches of the Phase diversity wave-front reconstruction techniques and its application in the high resolution image restoration[D]. Yunnan: Yunnan Observatory, Graduate School of Chinese Academy of Sciences, 2007 [3] Gonsalves R A, Chidlaw R. Wavefront sensing by phase retrieval[C]//Applications of Digital Image Processing III, 1979: 32-39. [4] Fusco T, Michau V, Mugnier L, et al. Comparative theoretical and experimental study of a Shack-Hartmann and a phase diversity sensor, for high-precision wavefront sensing dedicated to space active optics[C]//International Conference on Space Optics, 2014. [5] Gonsalves R A. Phase retrieval and diversity in adaptive optics[J]. Optical Engineering, 1982, 21(5): 829-832. [6] Zhang Peiguang, Yang Chengliang, Xu Zihao, et al. High-accuracy wavefront sensing by phase diversity technique with bisymmetric defocuses diversity phase[J]. Scientific Reports, 2017, 7(1): 15361. doi: 10.1038/s41598-017-15597-x [7] Nesterov Y. Gradient methods for minimizing composite objective function[J]. Core Discussion Papers, 2007, 140(1): 125-161. [8] Shewchuk J R. An introduction to the conjugate gradient method without the agonizing pain[M]. Pittsburgh (USA): Carnegie Mellon University, 1994. [9] 张小鸣, 李永新. 基于牛顿迭代法的高精度快速开方算法[J]. 电力自动化设备, 2008, 28(3):75-77. (Zhang Xiaoming, Li Yongxin. High-precision and fast square root algorithm based on Newton iteration method[J]. Electric Power Automation Equipment, 2008, 28(3): 75-77 doi: 10.3969/j.issn.1006-6047.2008.03.018 [10] Li Donghui, Fukushima M. On the global convergence of the BFGS method for nonconvex unconstrained optimization problems[J]. SIAM Journal on Optimization, 2001, 11(4): 1054-1064. doi: 10.1137/S1052623499354242 [11] Kirkpatrick S, Gelatt C D, VecchiM P. Optimization by simulated annealing[J]. Science, 1983, 220(4598): 671-680. doi: 10.1126/science.220.4598.671 [12] Holland J H. Adaptation in natural and artificial systems: an introductory analysis with applications to biology, control, and artificial intelligence[M]. Cambridge: MlT Press, 1992. [13] Eberhart R C, Kennedy J. A new optimizer using particle swarm theory[C]//Proceedings of the Sixth International Symposium on Micro Machine and Human Science. 1995. [14] Zhang Peiguang, Yang Chengliang, Xu Zihao, et al. Hybrid particle swarm global optimization algorithm for phase diversity phase retrieval[J]. Optics Express, 2016, 24(22): 25704. doi: 10.1364/OE.24.025704 [15] 徐梓浩. 基于相位差法的高分辨率液晶自适应光学技术研究[D]. 长春: 中国科学院长春光学精密机械与物理研究所, 2018.Xu Zihao. Research on high-resolution liquid crystal adaptive optics technique with phase diversity[D]. Changchun: Changchun Institute of Optics, Fine Mechanics and Physics, Chinese Academy of Sciences, 2018. [16] Ge Yingjian, Wang Shengqian, Xian Hao. Phase diversity method based on an improved particle swarm algorithm used in co-phasing error detection[J]. Applied Optics, 2020, 59(31): 9735-9743. doi: 10.1364/AO.404707 [17] Qi Xin, Ju Guohao, Zhang Chunyue, et al. Object-independent image-based wavefront sensing approach using phase diversity images and deep learning[J]. Optics Express, 2019, 27(18): 26102-26119. doi: 10.1364/OE.27.026102 [18] Wu Yu, Guo Youming, Bao Hua, et al. Sub-millisecond phase retrieval for phase-diversity wavefront sensor[J]. Sensors, 2020, 20(17): 4877. doi: 10.3390/s20174877 [19] Wu Daosheng, Yang Chengliang, Zhang Peiguang, et al. Phase diversity technique with sparse regularization in liquid crystal adaptive optics system[J]. Journal of Astronomical Telescopes Instruments and Systems, 2018, 4(1). [20] 李斐, 饶长辉. 相位差法波前传感系统自身误差的分析及消除方法[J]. 强激光与粒子束, 2011, 23(3):599-605. (Li Fei, Rao Changhui. Analysis and elimination of errors in phase diversity wavefront sensing system[J]. High Power Laser and Particle Beams, 2011, 23(3): 599-605 doi: 10.3788/HPLPB20112303.0599 [21] 王欣, 赵达尊. 图像噪声对相位变更波前传感的影响研究[J]. 光学学报, 2009, 29(8):2142-2146. (Wang Xin, Zhao Dazun. Influence of noise to phase diversity wavefront sensing[J]. Acta Optica Sinica, 2009, 29(8): 2142-2146 doi: 10.3788/AOS20092908.2142 [22] Yu Hongli, Yang Chengliang, Xu Zihao, et al. Analysis and reduction of errors caused by Poisson noise for phase diversity technique[J]. Optics Express, 2016, 24(19): 22034-22042. doi: 10.1364/OE.24.022034 [23] Li Dequan, Xu Shuyan, Wang Dong, et al. Phase diversity algorithm with high noise robust based on deep denoising convolutional neural network[J]. Optics Express, 2019, 27(16): 22846-22854. doi: 10.1364/OE.27.022846 [24] Paxman R G, Fienup J R. Optical misalignment sensing and image reconstruction using phase diversity[J]. Journal of the Optical Society of America A, 1988, 5(5): 914-923. [25] Lofdahl M G, Duncan A L, Paxman R G, et al. Phase diversity experiment to measure piston misalignment on the segmented primary mirror of the Keck II Telescope[J]. Astronomical Telescopes & Instrumentation, 1998, 3356: 1190-1201. [26] Blanc A, Fusco T, Hartung M, et al. Calibration of NAOS and CONICA static aberrations. Application of the phase diversity technique[J]. Astronomy & Astrophysics, 2003, 399: 373-83. [27] Georges J A, Dorrance P, Gleichman K, et al. High-speed closed-loop dual deformable-mirror phase-diversity testbed[C]. Proceedings of SPIE, 2007, 6711: 671105. [28] Lamb M, Correia C, Sauvage J F, et al. Exploring the operational effects of phase diversity for the calibration of non-common path errors on NFIRAOS[C]//SPIE Astronomical Telescopes + Instrumentation, 2016. [29] Carreras R A, Restaino S R. Field experimental results using phase diversity on a binary star[J]. NASA Technical Report, 1996: 97. [30] Hirzberger J, Feller A, Riethmüller T, et al. Performance validation of phase diversity image reconstruction techniques[J]. Astronomy & Astrophysics, 2011, 529: 1-5. [31] 鲍华, 饶长辉, 田雨, 等. 自适应光学图像事后重建技术研究进展[J]. 光电工程, 2018, 45(3):58-67. (Bao Hua, Rao Changhui, Tian Yu, et al. Research progress on adaptive optical image post reconstruction[J]. Opto-Electronic Engineering, 2018, 45(3): 58-67 [32] Wu Daosheng, Yang Chengliang, Li Hao, et al. Astronomical observation by 2-meter telescope based on liquid crystal adaptive optics with phase diversity[J]. Optics Communications, 2019, 439: 129-132. doi: 10.1016/j.optcom.2019.01.036 [33] 明名, 陈涛, 徐天爽. 基于相位差异技术的车载白天高分辨成像系统[J]. 光子学报, 2019, 48(3):129-137. (Ming Ming, Chen Tao, Xu Tianshuang. Vehicular daytime high-resolution imaging system based on phase-diversity technology[J]. Acta Photonica Sinica, 2019, 48(3): 129-137 -
下载:
下载:
点击查看大图
计量
- 文章访问数: 1954
- HTML全文浏览量: 443
- PDF下载量: 210
- 被引次数: 0
