Calculation model of critical radiated interference E-field intensity in reverberation chamber
-
摘要: 为解决均匀场与混响室内辐射敏感度测试结果相关性较差的问题，利用统计学理论对混响室内场强直角分量及天线接收功率的统计特性进行分析，理论推导出基于受试设备干扰概率的混响室条件下临界辐射干扰场强计算模型。为验证该模型的正确性，以ETS 3142E型天线为受试设备分别在混响室及均匀场中进行临界辐射干扰场强测试，实验结果表明，利用该计算模型得出的临界辐射干扰场强值与均匀场测试结果吻合良好，平均相对误差可控制在2 dB以内，可将该模型应用于实际辐射敏感度测试。Abstract: In order to solve the problem of the poor correlation of the radiation susceptibility test results between uniform field and reverberation chamber, this paper analyzes the statistical characteristics of the E-field rectangular component and the antenna's received power in reverberation chamber with statistical theory, and a calculation model of critical radiated interference E-field intensity base on the failure probability of equipment under test is derived. To verify the correctness of the calculation model, the ETS 3142E antenna is taken as equipment under test to perform the critical radiated interference E-field intensity test in uniform field and reverberation chamber. The experiment result shows that the critical radiated interference E-field intensity obtained by the calculation model is well consistent with the test result in uniform field, and the average relative error can be decreased to 2 dB, which indicates that this calculation model can be applied to' actual radiation susceptibility test.
-
Key words:
- reverberation chamber /
- uniform field /
- radiation susceptibility /
- correlation
-
图 2 混响室内统计规律验证
Figure 2. Statistical property validation of experiment data in reverberation chamber
图 4 临界辐射干扰场强的理论值与实测值比较
Figure 4. Comparison of theoritical and measured values of critical radiated interference E-field intensity
表 1 混响室试验数据
Table 1. Experiment data in reverberation chamber
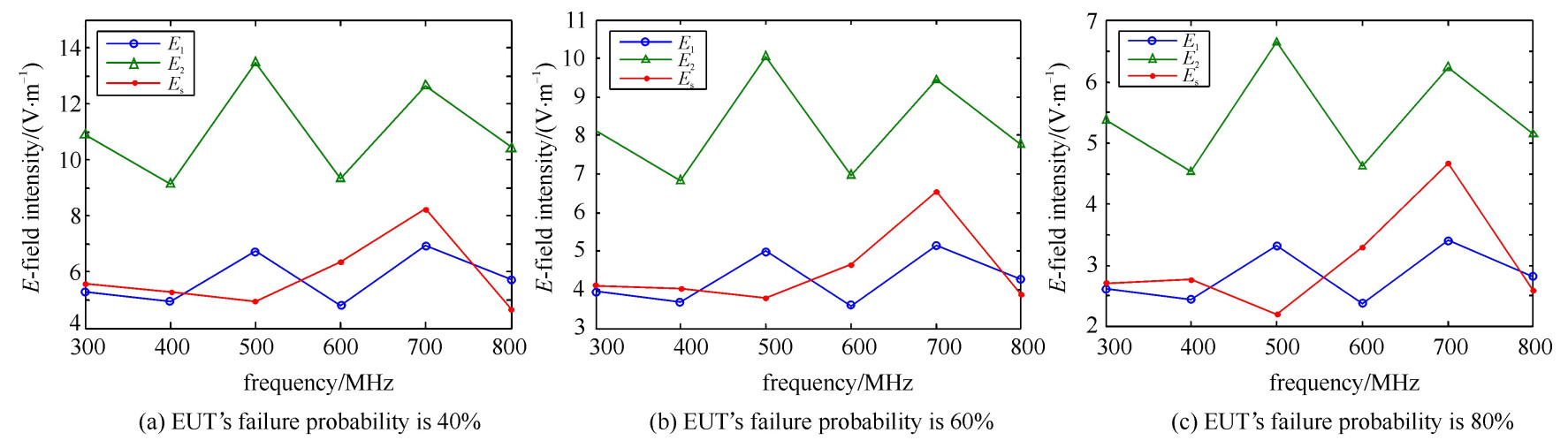
frequency/MHz G/dBi σ/(V·m-1) Ws/dBm P=40% P=60% P=80% 300 6.3 8.04 -44.56 -47.06 -50.58 400 5.2 6.76 -42.20 -44.76 -48.12 500 6 9.93 -42.26 -44.95 -49.70 600 5.7 6.89 -42.39 -45.02 -48.03 700 5 9.34 -42.20 -45.60 -48.32 800 5 7.68 -41.70 -43.2 -46.80 
下载: 导出CSV
表 2 开阔场试验数据
Table 2. Experiment data in open area
frequency/MHz Pout/dBm Es/(V·m-1) P=40% P=60% P=80% P=40% P=60% P=80% 300 -7.5 -10.2 -13.7 5.58 4.08 2.71 400 -3.9 -0.2 -3.6 5.28 4.05 2.77 500 -5.1 -7.4 -12.1 2.70 2.06 1.18 600 -1.2 -3.9 -6.8 6.35 4.65 3.3 700 -2 -0.6 -3.4 8.24 7.65 5.56 800 -1 -2.5 -6.1 4.65 3.90 2.58
下载: 导出CSV
表 3 不同频率下相对误差及均值
Table 3. Relative errors at different frequency and mean value
frequency/MHz ε1/dBm ε2/(V·m-1) P=40% P=60% P=80% P=40% P=60% P=80% 300 -0.43 -0.25 -0.29 5.80 5.98 5.94 400 -0.62 -0.86 -1.15 4.78 4.54 4.25 500 2.72 2.47 3.54 8.74 8.49 9.56 600 -2.45 -2.28 -2.89 3.35 3.52 2.90 700 -1.51 -2.03 -2.71 3.72 3.20 2.52 800 1.77 0.76 0.75 7.00 5.99 5.98 mean value 1.48 1.32 1.71 5.77 5.48 5.53
下载: 导出CSV
-
[1] 庄信武, 余志勇, 刘光斌, 等. 混沌腔体中电场边缘概率分布模型[J]. 高电压技术, 2014, 40(9): 2791-2796. https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201409026.htmZhuang Xinwu, Yu Zhiyong, Liu Guangbin, et al. Marginal probability distribution models of electric field in chaotic cavity. High Voltage Engineering, 2014, 40(9): 2791-2796 https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201409026.htm [2] 谢鑫, 王庆国, 贾锐. 混响室漫射场条件下树型线缆网络耦合规律仿真分析[J]. 科学技术与工程, 2015, 15(21): 131-135. doi: 10.3969/j.issn.1671-1815.2015.21.025Xie Xin, Wang Qingguo, Jia Rui, et al. Analyze of field to cable networks coupling in reverberation chamber's diffuse field. Science Technology and Engineering, 2015, 15(21): 131-135 doi: 10.3969/j.issn.1671-1815.2015.21.025 [3] Arnaut L R. Mode-stirred reverberation chambers: A paradigm for spatio-temporal complexity in dynamic electromagnetic environments[J]. Wave Motion, 2014, 51: 673-684. https://www.sciencedirect.com/science/article/pii/S0165212513001340 [4] Selemani K, Gros J B, Richalot E, et al. Comparison of reverberation chamber shapes inspired from chaotic cavities[J]. IEEE Trans Electromagnetic Compatibility, 2015, 57(1): 3-11. https://hal.archives-ouvertes.fr/hal-00980455/document [5] Fall A K, Besnier P, Lemoine C, et al. Design and experimental validation of a mode-stirred reverberation chamber at millimeter waves[J]. IEEE Trans Electromagnetic Compatibility, 2015, 57(1): 12-21. doi: 10.1109/TEMC.2014.2356712 [6] 魏光辉, 卢新福, 潘晓东. 强场电磁辐射效应测试方法研究进展与发展趋势[J]. 高电压技术, 2016, 42(5): 1347-1355. https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201605002.htmWei Guanghui, Lu Xinfu, Pan Xiaodong. Recent progress and development in test methods for high intensity electromagnetic field radiation effect. High Voltage Engineering, 2016, 42(5): 1347-1355 https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201605002.htm [7] IEC 61000-4-21: Electromagnetic compatibility (EMC)—Part4—21: Testing and measurement techniques—Reverberation chamber test methods[S]. 2008. [8] 张成怀. 混响室和GTEM室中电路射频辐照效应及其相关性研究[D]. 石家庄: 军械工程学院, 2009.Zhang Chenghuai. Research of the radio-frequency radiation effect on a circuit and their correlation in reverberation chamber and GTEM cell. Shijiazhuang: The Ordnance Engineering College, 2009 [9] 贾锐, 王庆国, 王树峤, 等. 混响室条件下辐射敏感度表征方法研究[J]. 北京理工大学学报, 2016, 36(1): 100-104. https://www.cnki.com.cn/Article/CJFDTOTAL-BJLG201601019.htmJia Rui, Wang Qingguo, Wang Shuqiao, et al. Research on the characterization of susceptibility threshold in reverberation chamber. Transactions of Beijing Institute of Technology, 2016, 36(1): 100-104 https://www.cnki.com.cn/Article/CJFDTOTAL-BJLG201601019.htm [10] 王庆国, 贾锐, 程二威. 混响室连续搅拌工作模式下的辐射抗扰度测试方法[J]. 高电压技术, 2010, 36(12): 2954-2959. https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201012019.htmWang Qingguo, Jia Rui, Cheng Erwei. Test methods of radiated immunity in reverberation chamber with continuously moving stirrer. High Voltage Engineering, 2010, 36(12): 2954-2959 https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201012019.htm [11] 陈京平, 贾锐, 唐斌, 等. 混响室条件下雷达电磁辐照效应[J]. 微波学报, 2014, 30(6): 55-58. https://www.cnki.com.cn/Article/CJFDTOTAL-WBXB201406014.htmChen Jingping, Jia Rui, Tang Bin, et al. Research on radiation effects to radar in reverberation chamber. Journal of Microwaves, 2014, 30(6): 55-58 https://www.cnki.com.cn/Article/CJFDTOTAL-WBXB201406014.htm [12] 熊久良, 刘心愿. 基于位置替代法的无线电引信混响室敏感度测试方法[J]. 高电压技术, 2015, 41(1): 320-326. https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201501046.htmXiong Jiuliang, Liu Xinyuan. Susceptibility test method of radio fuze in reverberation chamber based on position substitution method. High Voltage Engineering, 2015, 41(1): 320-326 https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201501046.htm [13] 魏光辉, 刘心愿, 孙永卫, 等. 混响室与均匀场中引信电磁辐射抗扰度测试相关性研究[J]. 高电压技术, 2015, 41(1): 287-293 https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201501041.htmWei Guanghui, Liu Xinyuan, Sun Yongwei, et al. Research on correlation of electromagnetic radiation susceptibility for radio fuze in uniform field and reverberation chamber. High Voltage Engineering, 2015, 41(1): 287-293 https://www.cnki.com.cn/Article/CJFDTOTAL-GDYJ201501041.htm [14] Amador E, Krauthauser H G, Besnier P. A binomial model for radiated immunity measurements[J]. IEEE Trans Electromagnetic Compatibility, 2013, 55(4): 683-691. https://hal.archives-ouvertes.fr/docs/00/85/04/51/PDF/article_proof.pdf [15] Amador E, Miry C, Bouyge N. Compatible susceptibility measurements in fully anechoic room and reverberation chamber[C]//Proc of the 2014 International Symposium on Electromagnetic Compatibility. 2014: 860-865. [16] Hoijer M. Maximum power available to stress onto the critical component in the equipment under test when performing a radiated susceptibility test in the reverberation chamber[J]. IEEE Trans Electromagnetic Compatibility, 2006, 48(2): 372-384. https://ieeexplore.ieee.org/document/1634752/ -
点击查看大图
图(4) / 表(3)
计量
- 文章访问数: 879
- HTML全文浏览量: 218
- PDF下载量: 339
- 被引次数: 0
