A passive efficiency calibration method with Monte Carlo simulation in segmented gamma scanning
-
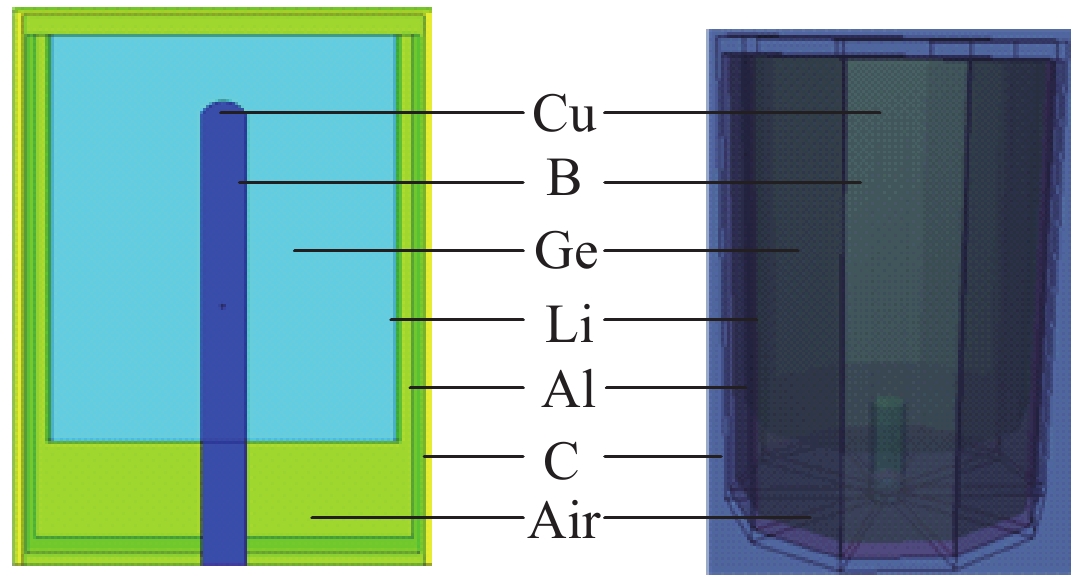
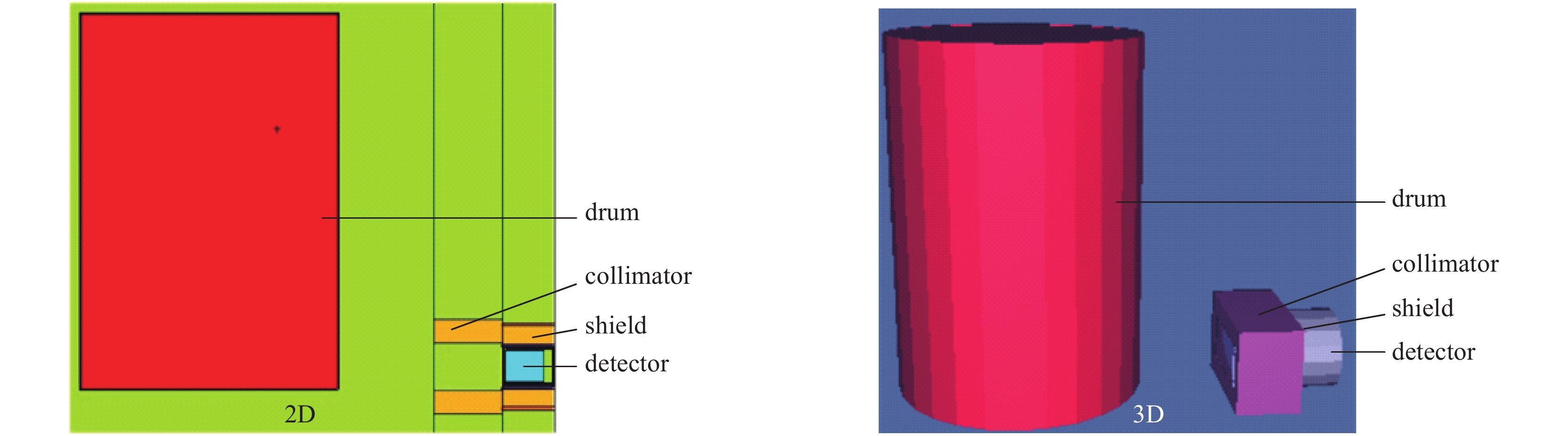
摘要: 针对200 L核废物桶分段γ扫描(SGS)过程中的效率刻度问题,提出了一种效率刻度函数模型,采用MCNP程序计算不同基质密度和γ射线能量条件下的离散断层效率,经过多元非线性回归获取函数参数,从而建立效率刻度函数,实现核废物桶SGS断层效率刻度。对核废物桶样品进行实验分析,结果表明:对于桶内基质分别为密度0.310 g·cm−3的硅酸铝、密度0.595 g·cm−3的木质纤维,桶内核素分别为活度3.110×105 Bq的点源137Cs、活度1.371×105 Bq的点源60Co,在桶内仅有单个点源存在的核素分布极端不均匀情况下,桶内核素活度重建误差在−37.68%~31.52%范围内。本文的方法能够准确有效实现核废物桶SGS断层效率矩阵计算,并确定核废物桶内放射性核素活度,满足实际检测要求。Abstract: In this work, an efficiency calibration function model is presented to calculate the efficiency of segment in segmented gamma scanning (SGS) for 200 L nuclear waste drum. Discrete SGS efficiencies are simulated with MCNP for different densities and gamma ray energies. Parameters of function are determined by using multivariate nonlinear regression method with the efficiencies. The SGS efficiency calibration function is constructed to calculate the efficiency matrix. Aluminum silicate with density of 0.310 g·cm−3, wood fiber of 0.595 g·cm−3, point sources 137Cs with activity of 3.110×105 Bq and 60Co of 1.371×105 Bq are used to construct samples of drum for SGS analysis. Result shows: for the extremely heterogeneous radioisotope distribution of only a point source placed at 8 different positions in the drum, errors of reconstructed activities are −37.68%~31.52%. Overall, the reconstructed activity is in agreement with the true activity. This method effectively and accurately achieves SGS efficiency calculation and reconstruction of activity.
-
Key words:
- nuclear waste drum /
- segmented gamma scanning /
- Monte Carlo /
- efficiency calibration /
- function /
- MLEM algorithm
-
激光自被发明以来,在材料加工[1-3]、国防军事和其他科技领域都具有巨大潜力,研究激光烧蚀作用的影响因素具有很强的理论与实际意义。近年来对激光的研究手段日益成熟[4],其中关于激光辐照时间[5]、入射强度、靶材材料等[6]影响烧蚀速率因素的理论和实验研究已有成果,然而对气压条件的研究均局限于环境气体[7-8]以及冲击压强等因素[9],蒸气压在其中的影响大多忽略处理[10],这有碍于对烧蚀过程的进一步研究。关于蒸气压对激光烧蚀作用的影响,已有的文献很少涉及。本文采用傅里叶热传导模型计算激光对靶材的烧蚀速率,然后分析烧蚀靶材产生的蒸气粒子运动情况并列出质量连续方程,计算了Knudsen层两侧的间断状态参数和考虑蒸气压时激光对靶材的烧蚀速率。
1. 强激光辐照靶材的热传导模型
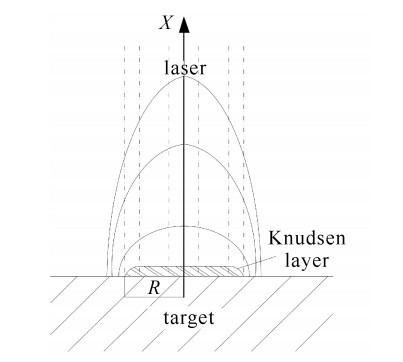
百兆瓦级功率激光辐照靶材时,沿着靶材厚度方向形成了梯度非常大的温度场,靶面温度在数秒内上升到气化温度并开始烧蚀。烧蚀掉的靶面物质高速喷溅,物质粒子相互碰撞,在靶面前方数个分子平均自由程(μm级)内达到平衡,并发生温度超过标准状况下气化温度的现象,这个区域被称作Knudsen层[11](靶材上方阴影部分)。粒子经过Knudsen层后形成稳定的高温蒸气流(如图 1)。
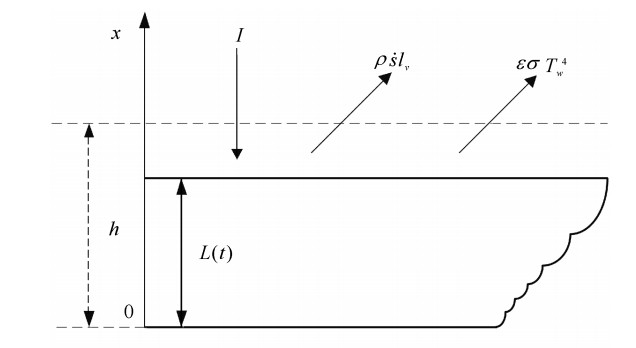
图 2上表面为靶材表面,发生烧蚀时以一定速度后退,沿激光辐照方向引起的温度场梯度比平行于靶材表面方向的温度场梯度要大得多,同时激光与靶材作用时间很短暂,故采用一维热传导控制方程[12],物性参数为常数,并且不考虑内热源。材料的热弛豫时间在ps到ns量级,而通常纳秒激光脉宽不小于10 ns, 一般不会发生非Fourier效应,因此计算采用一维的Fourier热传导控制方程[13]
ρc∂T∂t=K∂2T∂x2 (1) 式中:x是与热量传递方向相平行的坐标;K为热导率;ρ为介质密度;c为比热容;T为温度。假定材料出现升华烧蚀,且暂不考虑蒸气压对烧蚀的影响。考虑烧蚀时入射热流、向靶材内部传导的能量以及材料升华带走的能量三者能量守恒,得到边界条件:
(1)未发生烧蚀时:q=K∂T(x,t)∂x=Q−εσT4w,(T<Ts,x=h,t⩾0) (2) (2)发生烧蚀时:q=K∂T(x,t)∂x=Q−εσT4w−ρ˙slv,(T⩾Ts,x=L(t),t⩾0) (3) 靶材背面采用绝缘假设,边界条件为∂T(x,t)∂x=0(x=0,t⩾0),初始条件为T(x, t)=300 K(t=0, 0≤x≤L)。其中:Q=φI为激光功率等效的靶材入射热流,φ为吸收系数;ε为辐射系数,σ为Boltzman常数;Tw为受激光辐照时表面的瞬时温度;Ts为升华温度;h为初始板厚;L(t)为发生烧蚀后t时刻板厚; ˙s为线升华,即烧蚀速率;lv为受热靶材升华潜热。
对数理模型离散后运用基于Gauss消元法的Thomas算法(TDMA)可求解靶材在任意时刻任意位置的温度[14]。
2. 基于蒸气压的烧蚀过程分析
2.1 Knudsen层的间断条件
激光辐照使靶材表面气化形成稳定蒸气流,过渡区域为Knudsen层, 其内侧是靶材蒸发表面,外侧是稳定的蒸气流,两侧粒子温度、密度和压力形成了间断[15-16]。由Maxwell分布规律分别计算靶表面和Knudsen层外表面粒子速率:
f+s=ρ(2πRT)−3/2exp[−m(v2x+v2y+v2z)2σT] (4) f+k=ρ(2πRT)−3/2exp{−m[(vx−u)2+v2y+v2z]2σT} (5) 式中: vx,vy,vz为蒸气粒子速度分量;垂直离开靶表面的运动速度vx=√2σT/πm;m为原子质量;u为蒸气粒子平均速度;R是气体常数。
对两侧应用质量、动量和能量守恒条件,并将速率分布方程(4)~(5)代入,得到两侧温度和密度间断条件:
TkTs={[1+π(γ−1γ+1α2)2]12−π12γ−1γ+1α2}2 (6) ρkρs=√TsTk[(α2+12)eα2erfc(α)−α√π]+12TsTk[1−√παeα2erfc(α)] (7) 式中:下标k,s分别表示层外边界和靶材相变表面;α=u/√2kTk/m;比热比γ=Cp/Cv取5/3,Cp为定压比热容,Cv为定容比热容;erfc(α)为余误差函数。
2.2 连续方程
Knudsen层外表面的马赫数为
Mak=ukck=α√2γ (8) 式中:声速ck=√γkTk/m。
蒸气粒子流会推动外层空气形成冲击波,当激光功率足够强时,就必须考虑超声速情形。考虑各区域粒子运动情况并参考超声速波点燃阈值[17-18]后,取Mak=1。
由于蒸气粒子不全是沿垂直方向离开靶面方向,还有部分从界面上返回的,因此用β(β < 0)表示Knudsen层向内返回粒子的因子,可以列出质量连续方程:
ρcuc=(1−β)ρkuk (9) β=ρsρk√TsTkexp[(2α2+1)−α√πTsTk][α√πeα2erfc(α)−1] (10) 式中:ρcuc为靶材质量损失速率。
Knudsen层界面上的物理量可以用Clasius-Clayperon方程描述:
pkp0=exp[MlvRTv(1−TvTk)] (11) 式中:p0,Tv分别为ρk/ρs标准状况下饱和蒸气压力和温度。由该方程可知压力会影响气化温度进而影响质量损失率,因此计算时要耦合进去。
蒸气流的状态可以用气体状态方程描述:
p=nkT (12) 式中:n为粒子的密度。
结合式(9)~(12),并应用间断条件可以得到Knudsen层界面处的温度和压力,将新的温度和压力值带回到热传导方程(1)和边界条件(2)和(3),可以得到受蒸气压影响的烧蚀速率。
3. 计算分析
由于强激光辐照下,表面温度在很短时间内就会达到升华温度,所以参数取为常数。采用C/C复合材料作为靶材,工况参数如下:密度ρ=1800 kg/m3,比热容c=1870 J/(kg·K),热导率K=70 W/(m·K),潜热lv=5.903 4×107 J/kg,升华温度Tv=3873 K,表面热辐射系数ε=0.78,Boltzman常数σ=5.67×10-8 W/(m2·K4),吸收系数φ=0.7,初始温度T0=300 K。
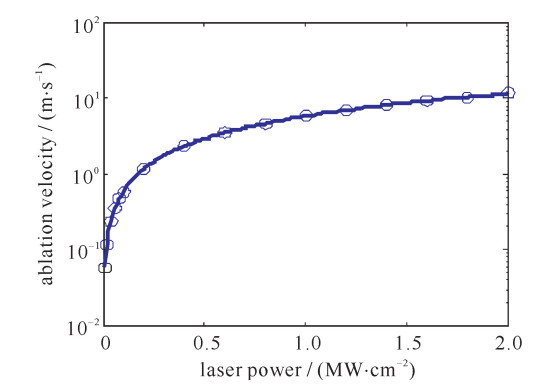
对上述热传导模型进行模拟,在实验得到烧蚀初始时间的基础上,从105~108 W/cm2量级间选取不同功率密度值,研究了忽略蒸气压时烧蚀速率随激光功率密度的变化规律,数据点拟合结果见图 3(纵坐标为对数)。可以看出随着功率密度的提升,烧蚀速率的增长几乎呈线性。
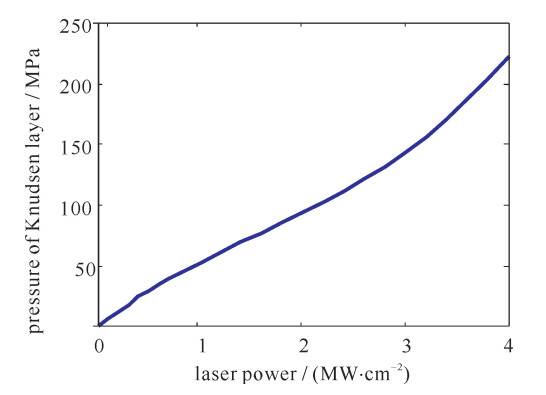
取直径为1 cm的靶材,得到表面功率不同时靶材烧蚀规律,图 4和图 5分别为Knudsen层蒸气压和表面温度随激光功率密度变化的结果,均显示为随激光功率密度的变大而增长。这是因为功率变大时表面激光转化成的等效热流值变大,烧蚀速率随之增加,溅射出来的粒子数变大,导致蒸气的饱和压力变大,由Clasius-Clayperon方程可知温度也会随之增加。
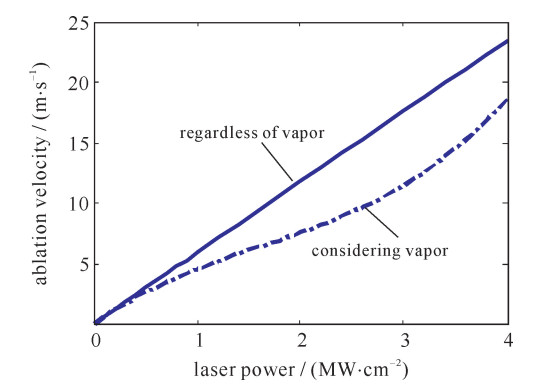
图 6显示了表面蒸气压力对烧蚀速率的影响,实线是未考虑蒸气压的计算结果,而虚线考虑了蒸气压的影响。蒸气压对烧蚀速率的影响比较大,并使其随功率变化表现出非线性规律,这是因为功率密度较高时饱和蒸气压非常大,影响了靶材的升华烧蚀,而烧蚀速率的变化反过来又会影响饱和蒸气压的值[15, 19-20]。
4. 结论
(1) 分析了离开靶材表面粒子形成的蒸气流,包括Knudsen层的形成及其两侧参数变化,计算发现靶材表面饱和蒸气温度高于标准气压下材料气化温度,随着激光功率的增大,靶材表面蒸气压力变大,表面温度也随之增加。
(2) 随着表面温度的增加,进一步分析对烧蚀过程的影响,与不考虑蒸气压条件的情况对比发现:蒸气压对烧蚀速率的影响比较大,使其随功率变化表现出非线性规律。
-
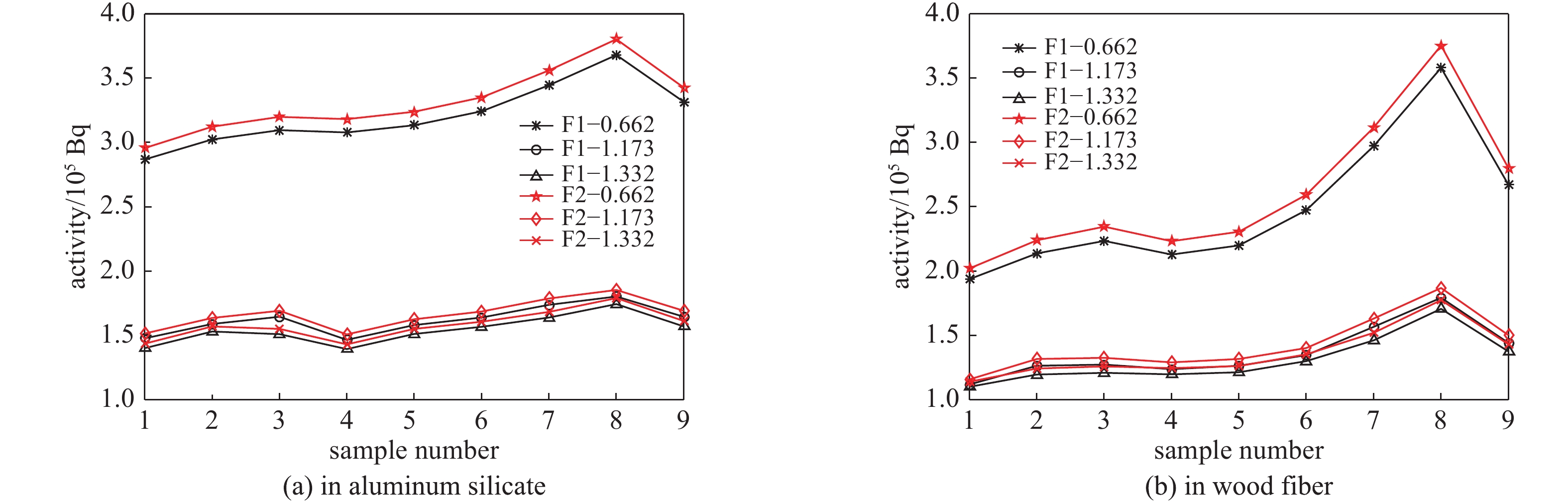
图 8 两种刻度函数参数下活度重建结果对比
Figure 8. Comparison of reconstructed activity with two sets of parameters (F1 and F2 represent parameters calibrated by aluminum silicate and wood fiber respectively, as shown in Table 1)
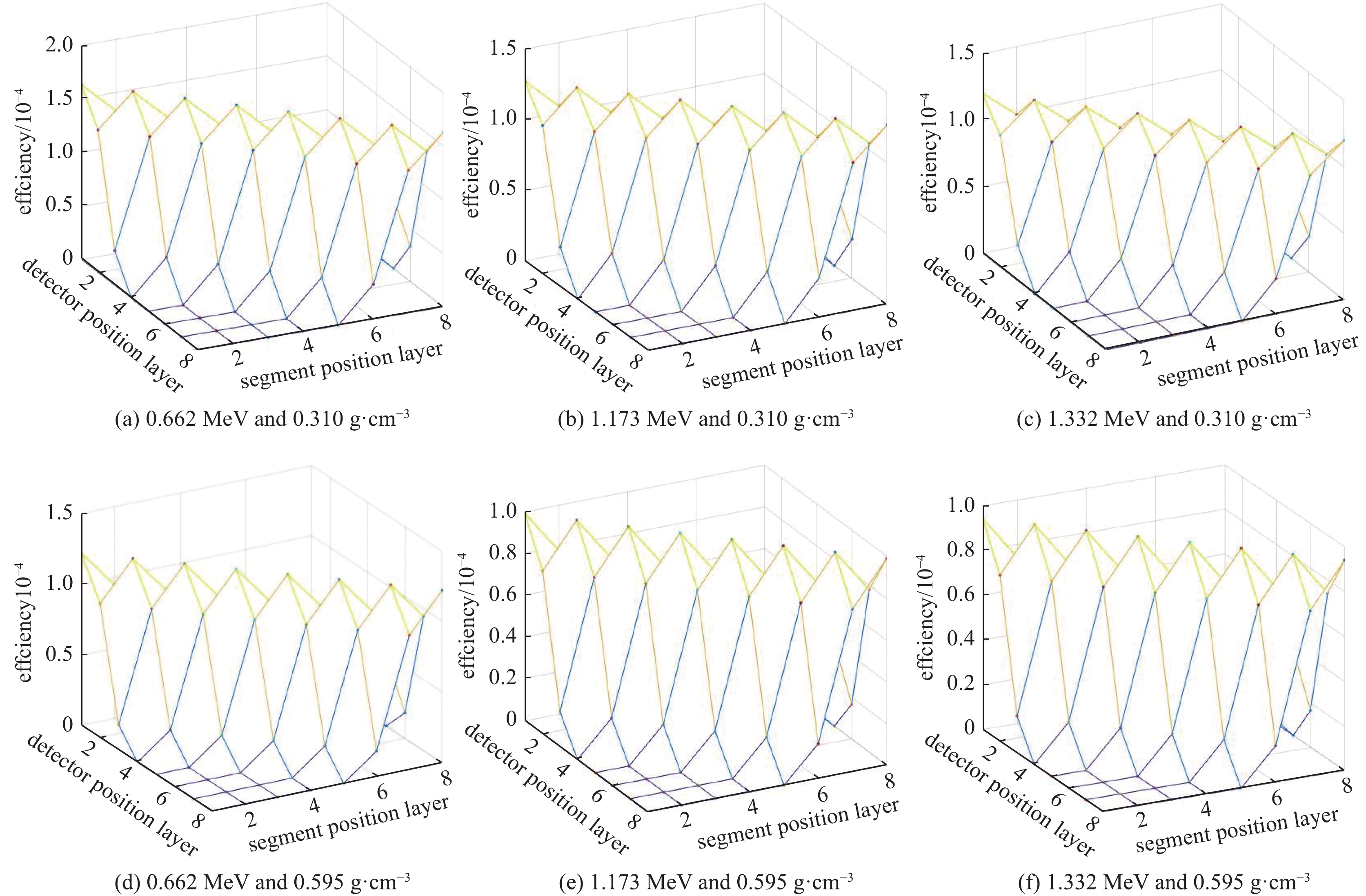
表 1 效率刻度函数参数
Table 1. Parameters of efficiency function
calibrating material spacing parameter a1 a2 a3 a4 a5 a6 R2 aluminum silicate 0 layer between detector and segment −8.214 −0.322 −0.681 −0.034 0.101 −0.601 0.998 1 layer between detector and segment −8.376 −0.303 −0.870 −0.051 0.110 −0.564 0.998 2 layers between detector and segment −10.094 −0.015 −2.014 −0.014 0.224 0.346 0.996 wood fiber 0 layer between detector and segment −8.152 −0.312 −0.676 −0.031 0.102 −0.713 0.999 1 layer between detector and segment −8.341 −0.293 −0.888 −0.047 0.111 −0.637 0.998 2 layers between detector and segment −10.052 0.011 −2.098 −0.015 0.231 0.276 0.996 表 2 137Cs和60Co核素的重建活度和误差
Table 2. Reconstructed activities and errors of 137Cs and 60Co
samples
No.activity in aluminum silicate/Bq(error/%) activity in wood fiber/Bq 0.662 MeV 1.173 MeV 1.332 MeV 0.662 MeV 1.173 MeV 1.332 MeV 1# 2.869×105(−7.74) 1.479×105(7.89) 1.403×105(2.33) 1.938×105(−37.68) 1.119×105(−18.35) 1.102×105(−19.54) 2# 3.025×105(−2.74) 1.591×105(16.08) 1.532×105(11.77) 2.138×105(−31.25) 1.265×105(−7.68) 1.197×105(−12.6) 3# 3.096×105(−0.46) 1.646×105(20.08) 1.512×105(10.32) 2.235×105(−28.15) 1.273×105(−7.09) 1.211×105(−11.64) 4# 3.079×105(−0.99) 1.468×105(7.07) 1.396×105(1.79) 2.129×105(−31.56) 1.239×105(−9.6) 1.199×105(−12.48) 5# 3.134×105(0.78) 1.582×105(15.39) 1.513×105(10.36) 2.199×105(−29.3) 1.265×105(−7.64) 1.215×105(−11.34) 6# 3.243×105(4.29) 1.640×105(19.61) 1.568×105(14.34) 2.474×105(−20.44) 1.347×105(−1.7) 1.302×105(−4.93) 7# 3.445×105(10.78) 1.739×105(26.81) 1.640×105(19.66) 2.972×105(−4.43) 1.567×105(14.35) 1.463×105(6.81) 8# 3.680×105(18.32) 1.803×105(31.52) 1.745×105(27.24) 3.579×105(15.07) 1.794×105(30.97) 1.708×105(24.65) 9# 3.316×105(6.63) 1.646×105(20.07) 1.572×105(14.68) 2.670×105(14.13) 1.442×105(5.28) 1.377×105(0.54) -
[1] 刘哲, 张丽. γ射线CT放射性废物桶检测技术综述[J]. CT理论与应用研究, 2014, 23(6):1025-1040. (Liu Zhe, Zhang Li. Review of γ-ray CT for radioactive waste assay[J]. CT Theory and Applications, 2014, 23(6): 1025-1040 [2] 张全虎. 层析γ扫描(TGS)重建技术的研究[D]. 北京: 中国原子能科学研究院, 2003.Zhang Quanhu. Research of tomographic gamma scanning (TGS) reconstruction technique[D]. Beijing: China Institute of Atomic Energy, 2003 [3] 莫继锋, 李美山, 张存平, 等. SGS技术在放射性固体废物整备检测中的应用[J]. 核电子学与探测技术, 2014, 34(8):950-953. (Mo Jifeng, Li Meishan, Zhang Cunping, et al. Application on the SGS technology in the measurement of radioactive solid waste conditioning[J]. Nuclear Electronics and Detection Technology, 2014, 34(8): 950-953 doi: 10.3969/j.issn.0258-0934.2014.08.008 [4] 周志波. 桶装核废物快速检测方法研究[D]. 北京: 中国原子能科学研究院, 2007.Zhou Zhibo. Research on the analysis method for the fast measurement of nuclear waste with γ spectrum[D]. Beijing: China Institute of Atomic Energy, 2007 [5] Parker J L. Use of calibration standards and the correction for sample self-attenuation in gamma-ray nondestructive assay[J]. Inorganic Organic Physical & Analytical Chemistry, 1984. [6] Prettyman T H, Reilly T D, Miller M C, et al. Advances in nuclear instrumentation for safeguards[R]. LA-UR-96-3757, 1996 [7] Camp D C, Martz H E, Roberson G P, et al. Nondestructive waste-drum assay for transuranic content by gamma-ray active and passive computed tomography[J]. Nuclear Instruments and Methods in Physics Research A, 2002, 495: 69-83. doi: 10.1016/S0168-9002(02)01315-3 [8] Hsue S T, Stewart J E. Guide to nondestructive assay standard preparation, criteria, availability and practical considerations[R]. La-13340-Ms, 2000. [9] 刘月恒, 屠荆, 仲云红. 环境样品测量中的一种自吸收校正方法研究[C]//全国第5届核仪器及其应用学术会议. 2005.Liu Yueheng, Tu Jing, Zhong Yunhong. A method of self-sorption correction for environmental radioactive measurement[C]//Proceedings of the 5th National Conference on Nuclear Instrument & Its Application. 2005 [10] Bosko A, Geurkov G, Croft S, et al. Advanced approach for calibration of the segmented gamma scanner for the radioassay of drummed waste[C]//2006 IEEE Nuclear Science Symposium Conference Record. 2006: 212-213. [11] Nakazawa D, Bronson F, Croft S, et al. The efficiency calibration of non-destructive gamma assay systems using semi-analytical mathematical approaches[C]//WM2010 Conference. 2010. [12] 易珂. 中低放射性废物活度无源γ测量实验及模拟计算研究[D]. 上海: 上海交通大学, 2009.Yi Ke. Experimental study and simulation of the passive gamma measurement on low-and-intermediate-level radioactive waste[D]. Shanghai: Shanghai Jiao Tong University, 2009 [13] Liang J H, Jiang S H, Chou G T, et al. A theoretical investigation of calibration methods for radwaste radioactivity detection systems[J]. Applied Radiation and Isotopes, 1996, 47(7): 669-675. doi: 10.1016/0969-8043(96)00031-0 [14] Bruggeman M, Gerits J, Carchon R. A minimum biased shell-source method for the calibration of radwaste assay systems[J]. Applied Radiation and Isotopes, 1999, 51(3): 255-259. doi: 10.1016/S0969-8043(99)00041-X [15] 徐利军, 叶宏生, 张卫东, 等. 分段γ扫描装置校准用桶状标准源的设计[J]. 核技术, 2015, 38:050502. (Xu Lijun, Ye Hongsheng, Zhang Weidong, et al. Design of reference radioactive source of waste drum used in calibration of segmented gamma scan device[J]. Nuclear Techniques, 2015, 38: 050502 [16] 许淑艳. 蒙特卡罗方法在实验核物理中的应用[M]. 北京: 原子能出版社, 2006.Xu Shuyan. Application of Monte Carlo method in nuclear physics experiment[M]. Beijing: Atomic Energy Press, 2006 [17] Dung T Q. Calculation of the systematic error and correction factors in gamma waste assay system[J]. Annals of Nuclear Energy, 1997, 24(1): 33-47. doi: 10.1016/0306-4549(96)00059-X 期刊类型引用(4)
1. 章航洲,曹俊杰,张永领,孙志军,胡冬梅,林力,吴耀,杜德福,王帅,陈戏三. 反应堆退役技术现状与展望. 核动力工程. 2024(03): 1-13 .  百度学术
百度学术2. 袁骏,何志军. 放射性环境下废物桶无损检测设备的设计. 自动化仪表. 2023(S1): 326-328+332 .  百度学术
百度学术3. 肖斌,范军林,杨庆华. 无人机航空放射性资源勘查环境监测系统及应用. 测绘通报. 2021(02): 140-143 .  百度学术
百度学术4. 苟家元,王力,吴耀,郑洪龙,王建强,黎皖豪,王鲁丰. HPGeγ谱仪测量土壤中~(60)Co活度的无源效率刻度. 核电子学与探测技术. 2021(06): 986-989 .  百度学术
百度学术其他类型引用(1)
-






 下载:
下载:






 下载:
下载: