Analysis on deformation of partial control network of particle accelerator
-
摘要: 基于泰勒展开的方法实现局部控制网的拟合，使用卡方检验判断局部控制网中是否存在形变点。若存在形变点，则在选权迭代的过程中找到局部控制网中所有的形变点，并将卡方检验通过作为迭代终止条件。对哈尔滨工业大学空间地面模拟装置2号终端控制网的两期观测成果进行了分析，实验表明，将卡方检验和选权迭代法加入控制网拟合之后，可以很好地探测出局部控制网中的形变点。在找出所有形变点之后，可以求得更为准确的局部控制网拟合参数。Abstract: The fitting of the local control network is realized based on the method of Taylor expansion, and the chi-square test is used to judge whether there is a deformation point in the local control network. If there are deformation points, find all the deformation points in the local control network in the process of weight selection iteration, repeat the above process until the chi-square test passes. The two-phase observation results of the Harbin Institute of Technology Space Environment Simulation and Research Infrastructure (SESRI) No.2 terminal control network are analyzed. The experiments show that after adding the chi-square test and the weight selection iteration method to the control network fitting, the deformation points in the local control network can be well detected. After finding all the deformation points, more accurate local control network fitting parameters can be obtained.
-
图 1 三维坐标系变换示意图
Figure 1. Schematic diagram of three-dimensional coordinate system transformation
图 3 初次平差后各点残差和权
Figure 3. Residuals and weights of each point after the initial adjustment
图 4 二次平差后各点残差与权
Figure 4. Residuals and weights of each point after the second adjustment
表 1 模拟计算
Table 1. Simulation calculation
calculations translation factor/mm rotation factor/(°) factor number of iterations ΔX ΔY ΔZ α β γ k 1 0 0 0 0 0 0 1 1 0 0 0 0 0 0 1 2 5 5 5 5 5 5 1.002 947 5 5 5 5 4.999 999 5 1.002 3 10 10 10 10 10 10 1.001 1005 10 10 10 10 9.999 999 10 1.001 4 20 20 20 20 20 20 0.999 1085 20 20 20 20 20 20 0.999 5 40 40 40 40 40 40 0.998 1521 40 40 39.999 999 40 40 40 0.998 6 60 60 60 60 60 60 0.997 1068 60 60 60 59.999 999 60 60 0.997 
下载: 导出CSV
表 2 SESRI实验终端2的两期观测值
Table 2. Two-phase observations of SESRI Experimental Terminal 2
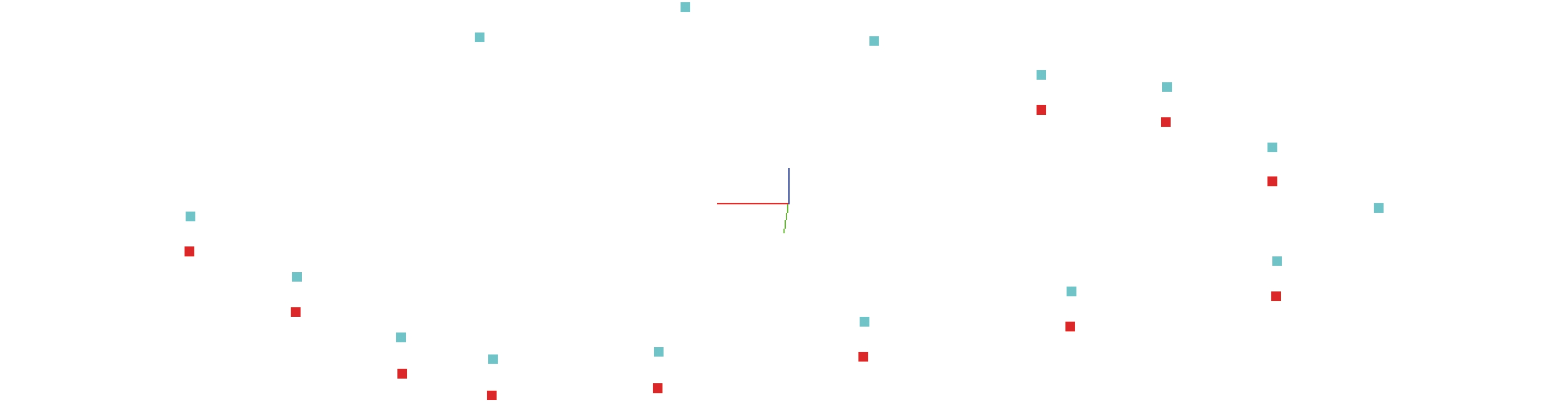
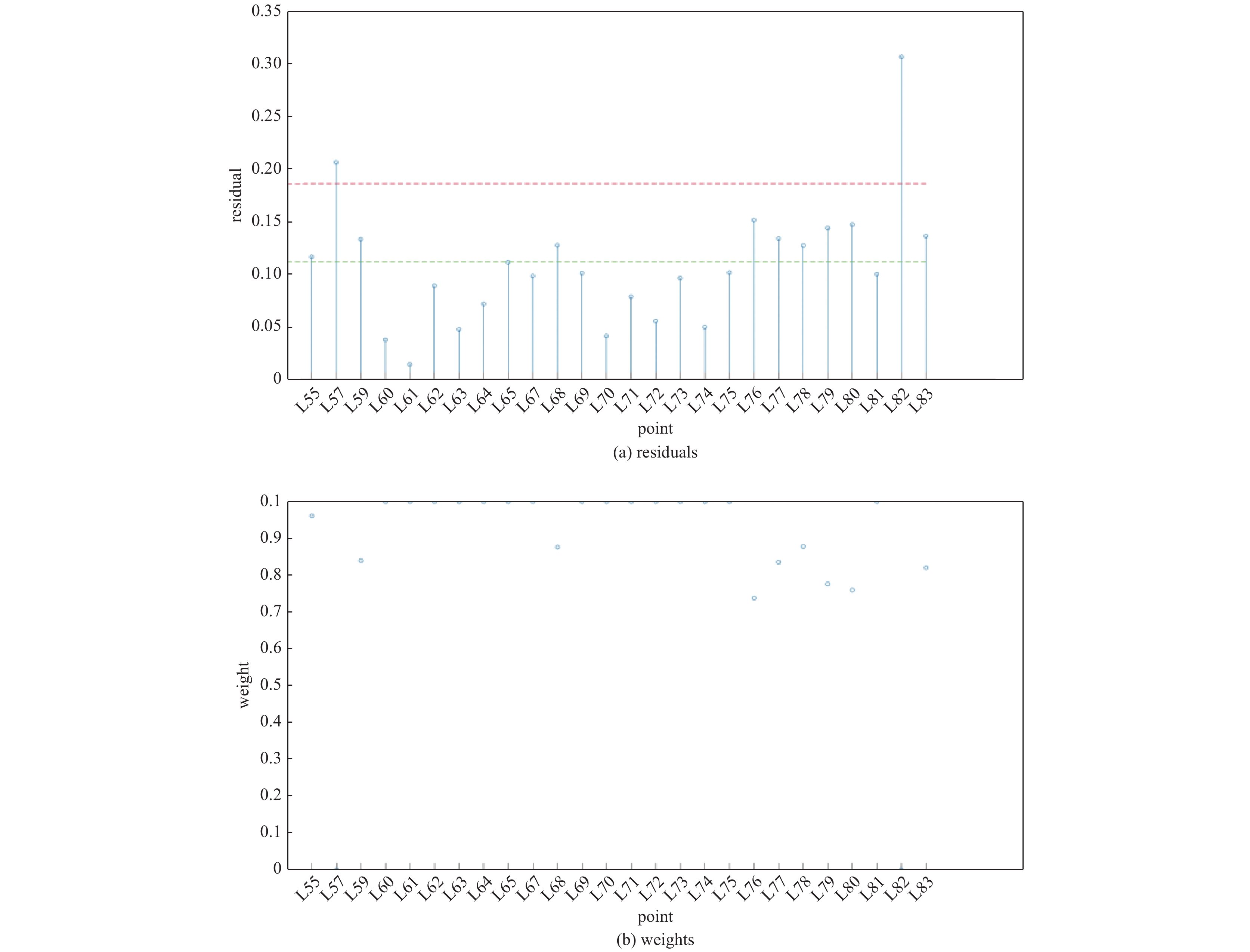
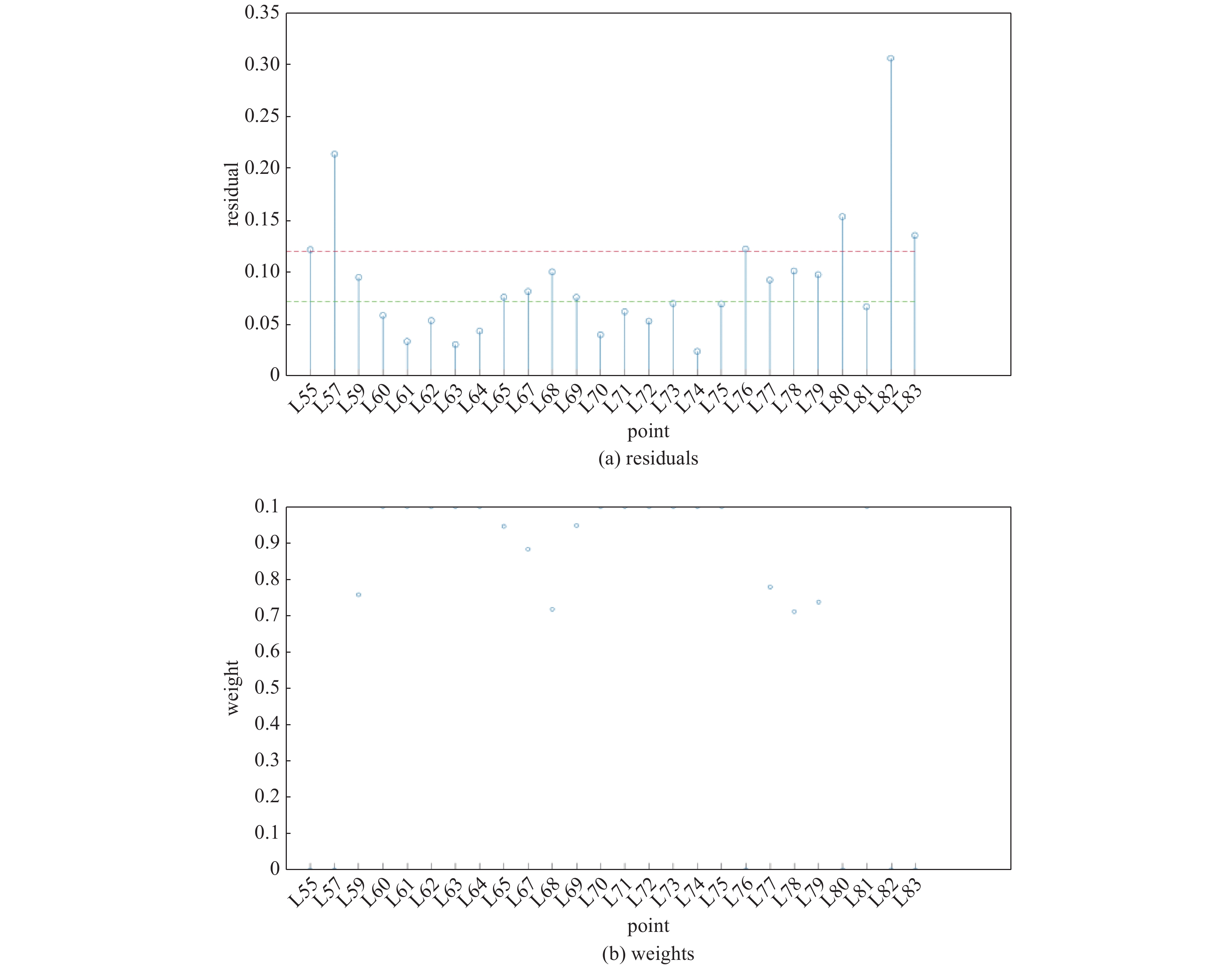
point the first phase (2021.4) the second phase (2021.6) X/mm Y/mm Z/mm X/mm Y/mm Z/mm L55 −5092.17 879.62 14895.65 6286.72 −15526.63 821.79 L57 −2 261.04 879.28 17717.09 2815.70 −17509.32 822.15 L59 −3120.62 879.22 21412.56 −520.04 −15701.26 822.40 L60 −4107.95 −821.72 24779.41 −3505.58 −13857.21 −878.36 L61 −4107.01 877.20 24777.54 −3503.76 −13858.44 820.70 L62 −4074.18 −817.67 27037.53 −5692.02 −13291.53 −874.05 L63 −4077.77 876.91 27033.99 −5687.46 −13288.83 820.64 L64 −6897.19 −773.87 29870.60 −7676.14 −9818.57 −830.23 L65 −6897.30 880.72 29867.91 −7673.23 −9819.01 824.42 L67 −9746.56 894.02 32716.15 −9665.07 −6316.66 837.73 L68 −13217.29 −822.38 31985.43 −8041.08 −3163.51 −879.28 L69 −13215.11 879.59 31991.11 −8046.88 −3164.01 822.76 L70 −16050.40 −820.92 29166.76 −4572.37 −1178.27 −878.59 L71 −16047.74 874.53 29165.73 −4571.81 −1180.86 816.96 L72 −18878.70 −809.52 26339.60 −1096.73 800.14 −867.90 L73 −18879.30 879.65 26340.53 −1097.19 801.22 821.38 L74 −21711.32 −841.15 23519.47 2373.33 2784.62 −900.23 L75 −21710.28 846.76 23519.11 2373.62 2783.72 787.79 L76 −23062.45 −815.86 20935.80 5222.75 3403.02 −875.45 L77 −23061.11 882.09 20947.33 5211.70 3405.02 822.63 L78 −22368.90 −818.96 19093.13 6816.01 2245.99 −878.70 L79 −22309.74 883.25 19037.43 6854.43 2174.44 823.70 L80 −19511.28 −781.72 16233.69 8816.34 −1267.34 −841.25 L81 −19509.35 875.00 16238.17 8811.89 −1267.75 815.53 L82 −16684.40 −808.82 13405.92 10794.26 −4742.76 −868.25 L83 −16690.55 881.77 13407.80 10794.50 −4735.99 822.43
下载: 导出CSV
表 3 两种方法求得的拟合参数
Table 3. Fitting parameters obtained by two methods
calculation translation factor/mm rotation factor/(°) factor ΔX ΔY ΔZ α β γ k SA −18 397.56 58.4 25 070.31 89.974 03 74.637 72 179.9675 0.999 926 this article −18 397.55 58.40 25 070.31 89.9743 74.637 71 179.967 8 0.999 926 difference 0.009 4 −0.001 5 0.000 3 0.00027 −0.000 01 0.000 234 −0.000 000 4
下载: 导出CSV
-
[1] 王岩, 岳建平, 周保兴, 等. 工程控制网点位稳定性分析方法的研究[J]. 测绘通报, 2004(8):12-14. (Wang Yan, Yue Jianping, Zhou Baoxing, et al. Research on the stability analysis method of engineering control network points[J]. Bulletin of Surveying and Mapping, 2004(8): 12-14 doi: 10.3969/j.issn.0494-0911.2004.08.005 [2] 周江文, 欧吉坤. 名次法及拟稳点的选定[J]. 测绘学报, 1987(2):10-16. (Zhou Jiangwen, Ou Jikun. Ranking method and selection of pseudo-stable points[J]. Journal of Surveying and Mapping, 1987(2): 10-16 [3] 周江文, 欧吉坤. 拟稳点的更换——兼论自由网平差若干问题[J]. 测绘学报, 1984(3):3-12. (Zhou Jiangwen, Ou Jikun. Replacement of pseudo-stable points—Also on some problems of free network adjustment[J]. Journal of Surveying and Mapping, 1984(3): 3-12 [4] 张广伟, 李鹏, 宫辉. 城市地铁控制网稳定性分析及应用[J]. 测绘科学, 2008, 33(4):98-99. (Zhang Guangwei, Li Peng, Gong Hui. Stability analysis and application of urban subway control network[J]. Science of Surveying and Mapping, 2008, 33(4): 98-99 doi: 10.3771/j.issn.1009-2307.2008.04.033 [5] 孙丕川, 黄声享, 李冠青. 港珠澳大桥岛隧工程GPS控制点稳定性研究[J]. 测绘通报, 2014(S2):58-59. (Sun Pichuan, Huang Shengxiang, Li Guanqing. Research on GPS control point stability of Hong Kong-Zhuhai-Macao Bridge Island Tunnel Project[J]. Bulletin of Surveying and Mapping, 2014(S2): 58-59 [6] 崔家武, 张兴福, 周波阳, 等. 改进的GNSS/水准点优化选择的逐步剔除法[J]. 武汉大学学报(信息科学版), 2019, 44(10):1505-1510. (Cui Jiawu, Zhang Xingfu, Zhou Boyang, et al. Stepwise elimination method for improved GNSS/leveling point optimization selection[J]. Journal of Wuhan University (Information Science Edition), 2019, 44(10): 1505-1510 [7] 吴迪军, 熊伟, 何婵军. 港珠澳大桥首级控制网四期测量成果比较与分析[J]. 测绘科学, 2013, 38(4):83-85. (Wu Dijun, Xiong Wei, He Chanjun. Comparison and analysis of the fourth-phase survey results of the first-level control network of the Hong Kong-Zhuhai-Macao Bridge[J]. Science of Surveying and Mapping, 2013, 38(4): 83-85 [8] 陈文军. 重离子治疗装置的准直关键技术研究与应用[D]. 兰州: 中国科学院大学(中国科学院近代物理研究所), 2020: 22-24Chen Wenjun. Research and application of the key technology of heavy ion therapy device alignment[D]. Lanzhou: University of Chinese Academy of Sciences (Institute of Modern Physics, Chinese Academy of Sciences), 2020: 22-24 [9] 郭迎钢, 李宗春, 李广云, 等. 粒子加速器工程控制网研究进展与展望[J]. 测绘通报, 2020(1):136-141. (Guo Yinggang, Li Zongchun, Li Guangyun, et al. Research progress and prospects of particle accelerator engineering control network[J]. Bulletin of Surveying and Mapping, 2020(1): 136-141 [10] Guo Yinggang, Li Zongchun. A sectional control method to decrease the accumulated survey error of tunnel installation control network[J]. American Journal of Modern Physics, 2021, 10(1): 7. [11] 李方, 邹进贵, 王铜, 等. 粒子直线加速器精密三维控制网研究[J]. 地理空间信息, 2018, 16(2):87-89,110. (Li Fang, Zou Jingui, Wang Tong, et al. Research on precision three-dimensional control network of particle linear accelerator[J]. Geospatial Information, 2018, 16(2): 87-89,110 doi: 10.3969/j.issn.1672-4623.2018.02.029 [12] 马娜, 董岚, 梁静, 等. 基于加速器控制网的GPS绝对测量精度探讨[J]. 北京测绘, 2014(6):23-27,43. (Ma Na, Dong Lan, Liang Jing, et al. Discussion on GPS absolute measurement accuracy based on accelerator control network[J]. Beijing Surveying and Mapping, 2014(6): 23-27,43 doi: 10.3969/j.issn.1007-3000.2014.06.007 [13] 郭迎钢, 李宗春, 刘忠贺, 等. 加速器隧道控制网变形可监测性及稳定性分析[J]. 原子能科学技术, 2019, 53(9):1634-1642. (Guo Yinggang, Li Zongchun, Liu Zhonghe, et al. Deformation monitoring and stability analysis of accelerator tunnel control network[J]. Atomic Energy Science and Technology, 2019, 53(9): 1634-1642 doi: 10.7538/yzk.2019.youxian.0216 [14] Chen Y Q, Chrzanowski A, Secord J M. A strategy for the analysis of the stability of reference points in deformation surveys[J]. CISM Journal ACSGC, 1990, 44(2): 141-149. doi: 10.1139/geomat-1990-0016 [15] Nowel K, Kaminski W. Robust estimation of deformation from observation differences for free control networks[J]. Journal of Geodesy, 2014, 88(8): 749-764. [16] Wilkins R, Bastin G, Chrzanowski A. ALERT: A fully automated real time monitoring system[C]//Proceedings of the 11th FIG Symposium on Deformation Measurements. 2003. [17] Bocean V, Coppola G, Ford R, et al. Status report on the survey and alignment activities at Fermilab[C]//Proceedings of the 9th International Workshop on Accelerator Alignment. 2006. [18] Nowel K. Squared M split(q) S-transformation of control network deformations[J]. Journal of Geodesy, 2019, 93(7): 1025-1044. doi: 10.1007/s00190-018-1221-4 [19] Nowel K. Specification of deformation congruence models using combinatorial iterative DIA testing procedure[J]. Journal of Geodesy, 2020, 94(12): 1-23. [20] 武汉大学测绘学院测量平差学科组编著. 误差理论与测量平差基础[M]. 武汉: 武汉大学出版社, 2003: 173-176Edited by the Surveying Adjustment Discipline Group, School of Surveying and Mapping, Wuhan University. Error theory and the foundation of surveying adjustment[M]. Wuhan: Wuhan University Press, 2003: 173-176 [21] 曾文宪, 陶本藻. 三维坐标转换的非线性模型[J]. 武汉大学学报(信息科学版), 2003(5):566-568. (Zeng Wenxian, Tao Benzao. Non-linear model of three-dimensional coordinate transformation[J]. Journal of Wuhan University (Information Science Edition), 2003(5): 566-568 [22] 陈义, 沈云中, 刘大杰. 适用于大旋转角的三维基准转换的一种简便模型[J]. 武汉大学学报(信息科学版), 2004, 29(12):1101-1105. (Chen Yi, Shen Yunzhong, Liu Dajie. A simple model of three-dimensional datum conversion with large rotation angle[J]. Journal of Wuhan University (Information Science Edition), 2004, 29(12): 1101-1105 [23] 姚吉利, 韩保民, 杨元喜. 罗德里格矩阵在三维坐标转换严密解算中的应用[J]. 武汉大学学报(信息科学版), 2006, 31(12):1094-1096,1119. (Yao Jili, Han Baomin, Yang Yuanxi. The application of Rodriguez matrix in the rigorous calculation of three-dimensional coordinate transformation[J]. Journal of Wuhan University (Information Science Edition), 2006, 31(12): 1094-1096,1119 [24] 陆珏, 陈义, 郑波. 总体最小二乘方法在三维坐标转换中的应用[J]. 大地测量与地球动力学, 2008(5):77-81. (Lu Jue, Chen Yi, Zheng Bo. The application of total least square method in three-dimensional coordinate transformation[J]. Journal of Geodesy and Geodynamics, 2008(5): 77-81 [25] 姚宜斌, 黄承猛, 李程春, 等. 一种适用于大角度的三维坐标转换参数求解算法[J]. 武汉大学学报(信息科学版), 2012, 37(3):253-256. (Yao Yibin, Huang Chengmeng, Li Chengchun, et al. A three-dimensional coordinate conversion parameter solving algorithm suitable for large angles[J]. Journal of Wuhan University (Information Science Edition), 2012, 37(3): 253-256 [26] 陈义, 陆珏. 以三维坐标转换为例解算稳健总体最小二乘方法[J]. 测绘学报, 2012, 41(5):715-722. (Chen Yi, Lu Jue. Taking three-dimensional coordinate transformation as an example to solve the robust total least squares method[J]. Journal of Surveying and Mapping, 2012, 41(5): 715-722 [27] 方兴, 曾文宪, 刘经南, 等. 三维坐标转换的通用整体最小二乘算法[J]. 测绘学报, 2014, 43(11):1139-1143. (Fang Xing, Zeng Wenxian, Liu Jingnan, et al. General least squares algorithm for three-dimensional coordinate transformation[J]. Journal of Surveying and Mapping, 2014, 43(11): 1139-1143 [28] 李仕东. 工程测量[M]. 北京: 人民交通出版社, 2005: 20-24Li Shidong. Engineering Surveying[M]. Beijing: People's Communications Press, 2005: 20-24 [29] Huber P J . Robust statistics[ M] . New York : Wiley , 1981: 10-15. [30] 周江文. 经典误差理论与抗差估计[J]. 测绘学报, 1989(2):115-120. (Zhou Jiangwen. Classical error theory and robust estimation[J]. Acta Geomatica Survey and Mapping, 1989(2): 115-120 doi: 10.3321/j.issn:1001-1595.1989.02.005 -
点击查看大图
图(4) / 表(3)
计量
- 文章访问数: 758
- HTML全文浏览量: 351
- PDF下载量: 24
- 被引次数: 0
