Modeling and application of electromagnetic coupling cross section of building walls
-
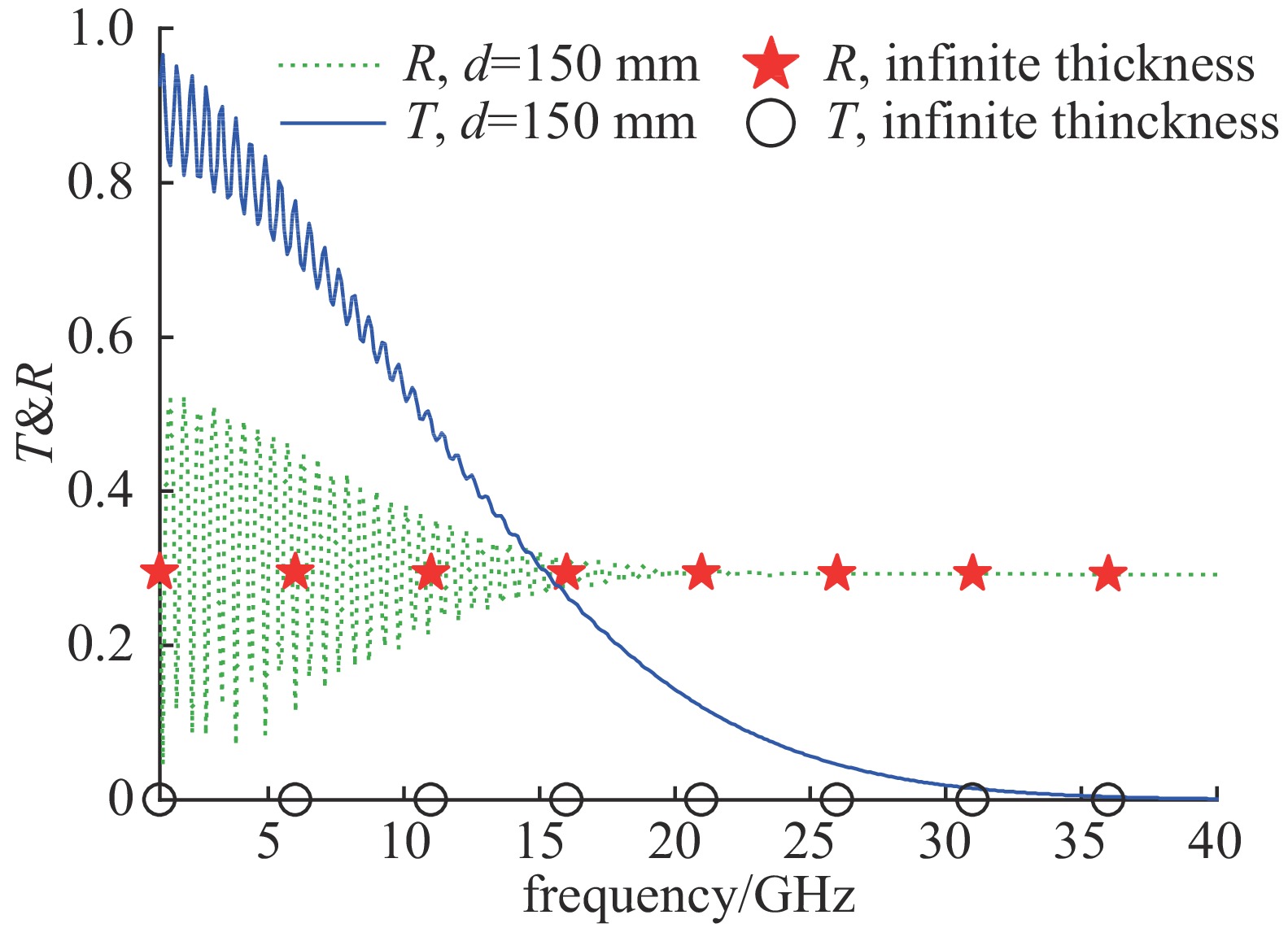
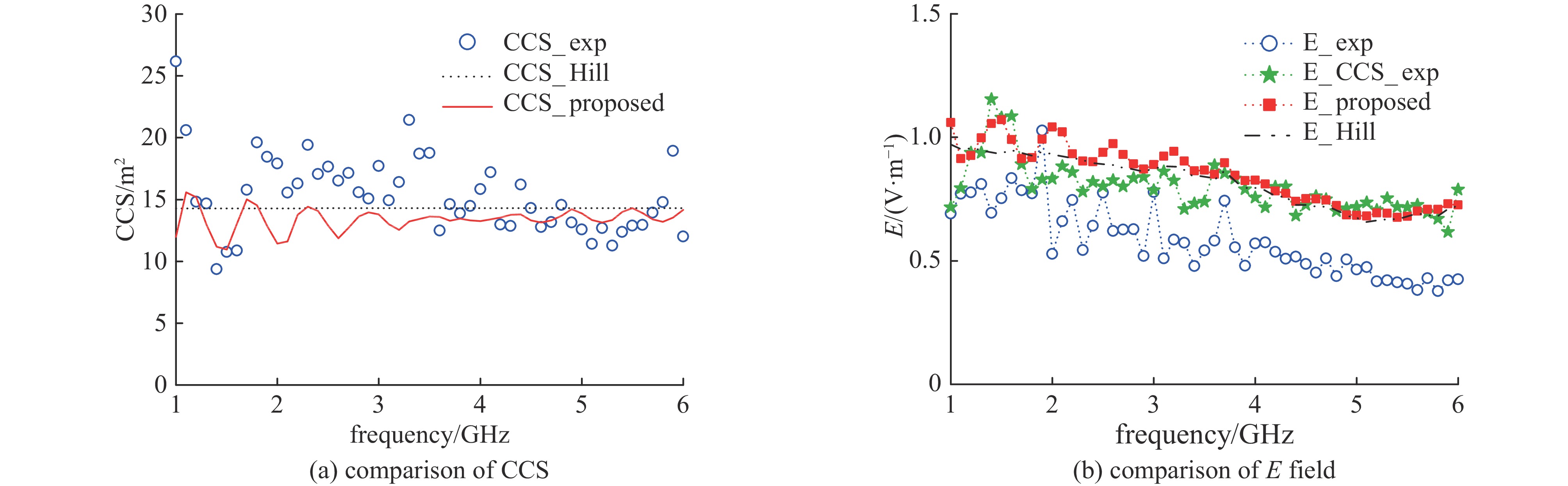
摘要: 电磁波照射下的建筑物室内电磁环境具有混响效果,因此可采用功率平衡法(PWB)快速评估室内电磁环境水平。然而目前PWB方法中电大腔壁耦合截面(CCS)的计算模型建立在腔内电磁波不穿透腔壁的条件下,无法直接用于电磁波可穿透室内建筑物墙壁的耦合截面计算。为此,提出了一种适用于电磁波穿透有限厚度建筑物墙壁的CCS计算新模型。该模型考虑实际建筑物墙体的厚度和材料电磁特性,能够充分反映电磁波因有限厚度墙壁多次反射对室内电磁环境水平的影响。将该模型应用于室内电场水平的快速评估,预测结果与实际测量结果吻合较好,证明了所提有限厚度建筑物墙壁CCS模型的合理性。Abstract: The electromagnetic waves radiating inside a building can cause reverberation effect, which can be evaluated using power balance method (PWB) to quickly determine the field level of indoor electromagnetic environment. However, the current calculation models of wall coupling cross section (CCS) in PWB method for electricallally large enclosure are based on the assumption that electromagnetic waves cannot penetrate through the enclosure walls. As a result, these models are not applicable for calculating the CCS of penetrable indoor building walls. To address this issue, a novel CCS model applicable for building walls with finite thickness is presented. The proposed CCS model considers the thickness and electromagnetic characteristics of building walls and can effectively reflect the effects of electromagnetic wave’s multiple reflections inside the walls on the indoor electromagnetic environment. The proposed model has been employed to estimate the indoor electric field level. The predicted results agree with the measurements, which validates the proposed CCS model for building walls with finite thickness.
-
表 1 材料序列号
Table 1. Material serial number
No. material d/mm No. material d/mm 1 plexiglass 6, 10, 12 2 PP − 3 teflon − 4 polystyrene − 5 pine wood − 6 hardboard − 7 5-ply plywood − 8 MDF 2−30 9 MDF with gray veneer 2−30 10 MDF with brown veneer 2−30 11 chipboard − 12 chipboard with veneer − 13 glass 6, 10, 12 14 wood-cement board − 15 gypsum plaster − 16 plasterboard 6, 8, 9, 12, 14, 16, 18 17 red brick 120, 240, 370, 490 18 yellow brick 120, 240, 370, 490 19 concrete with small gravel 75, 100, 150; 160, 180, 200 20 concrete with large gravel 75, 100, 150; 160, 180, 200 表 2 常见建筑材料归一化耦合截面计算
Table 2. Normalized CCS calculation for common building materials
material d/mm WCCS/m2 TCCS/m2 WCCS/m2 TCCS/m2 WCCS/m2 TCCS/m2 Ref [5]
CCS/m22.4 GHz 5.5 GHz 27 GHz plexiglass 6~12 0.001~0.002 0.214~0.185 0.002~0.004 0.181~0.211 0.012~0.021 0.215~0.187 0.223 glass 6~12 0.001~0.001 0.150~0.120 0.002~0.009 0.122~0.178 0.062~0.084 0.138~0.088 0.194 plasterboard 6~18 0.009~0.016 0.210~0.169 0.013~0.041 0.177~0.179 0.054~0.117 0.176~0.105 0.224 red brick 120~490 0.007~0.025 0.196~0.178 0.026~0.084 0.183~0.128 0.201~0.219 0.018~0.000 0.219 concrete with small gravel 75~200 0.013~0.039 0.163~0.170 0.047~0.099 0.158~0.111 0.209~0.215 0.006~0.000 0.215 concrete with large gravel 75~200 0.016~0.048 0.144~0.145 0.057~0.117 0.142~0.089 0.205~0.210 0.005~0.000 0.210 -
[1] Obeidat H, Alabdullah A, Elkhazmi E, et al. Indoor environment propagation review[J]. Computer Science Review, 2020, 37: 100272. doi: 10.1016/j.cosrev.2020.100272 [2] Steinböck G, Pedersen T, Fleury B H, et al. Experimental validation of the reverberation effect in room electromagnetics[J]. IEEE Transactions on Antennas and Propagation, 2015, 63(5): 2041-2053. doi: 10.1109/TAP.2015.2423636 [3] Yusuf M, Tanghe E, Martinez-Ingles M T, et al. Frequency-dependence characterization of electromagnetic reverberation in indoor scenarios based on 1- 40 GHz channel measurements[J]. IEEE Antennas and Wireless Propagation Letters, 2019, 18(10): 2175-2179. doi: 10.1109/LAWP.2019.2939662 [4] Hill D A, Ma M T, Ondrejka A R, et al. Aperture excitation of electrically large, lossy cavities[J]. IEEE Transactions on Electromagnetic Compatibility, 1994, 36(3): 169-178. doi: 10.1109/15.305461 [5] Hill D A. A reflection coefficient derivation for the Q of a reverberation chamber[J]. IEEE Transactions on Electromagnetic Compatibility, 1996, 38(4): 591-592. doi: 10.1109/15.544314 [6] Junqua I, Parmantier J P, Ridel M. Modeling of high frequency coupling inside oversized structures by asymptotic and PWB methods[C]//Proceedings of 2011 International Conference on Electromagnetics in Advanced Applications. 2011: 68-71. [7] 贾锐, 耿利飞, 王川川, 等. 混响室内加载物损耗特性试验研究[J]. 强激光与粒子束, 2022, 34:113003 doi: 10.11884/HPLPB202234.220039Jia Rui, Geng Lifei, Wang Chuanchuan, et al. Research on the characteristics of lossy objects in a reverberation chamber[J]. High Power Laser and Particle Beams, 2022, 34: 113003 doi: 10.11884/HPLPB202234.220039 [8] 欧阳婷, 刘强, 赵翔. 有球形损耗物的矩形开孔电大腔的PWB分析[J]. 无线电工程, 2017, 47(8):71-74 doi: 10.3969/j.issn.1003-3106.2017.08.17Ouyang Ting, Liu Qiang, Zhao Xiang. Application of PWB method in the analysis of EM-field environment in an electrically large cavity with rectangular aperture when a spherical loss material inside[J]. Radio Engineering, 2017, 47(8): 71-74 doi: 10.3969/j.issn.1003-3106.2017.08.17 [9] Lee H H, Lee J W. Analysis of electromagnetic effect inside large-scaled building by external electromagnetic wave using PWB method[C]//Proceedings of 2018 International Symposium on Antennas and Propagation (ISAP). 2018: 1-2. [10] Balanis C A. Advanced engineering electromagnetics[M]. New York: Wiley, 1989. [11] 德马雷斯特. 工程电磁学[M]. 北京: 科学出版社, 2003Demarest K R. Engineering electromagnetics[M]. Beijing: Science Press, 2003 [12] Jensen P D, Meaney P M, Epstien N R, et al. Cole-Cole parameter characterization of urea and potassium for improving dialysis treatment assessment[J]. IEEE Antennas and Wireless Propagation Letters, 2012, 11: 1598-1601. doi: 10.1109/LAWP.2012.2237536 [13] Zhekov S S, Franek O, Pedersen G F. Dielectric properties of common building materials for ultrawideband propagation studies [measurements corner][J]. IEEE Antennas and Propagation Magazine, 2020, 62(1): 72-81. doi: 10.1109/MAP.2019.2955680 [14] 杨柳, 朱新荣, 刘大龙, 等. 建筑物理[M]. 北京: 中国建材工业出版社, 2014Yang Liu, Zhu Xinrong, Liu Dadong, et al. Architectural physics[M]. Beijing: China Building Materials Industry Press, 2014 [15] 杨春宇, 唐鸣放, 谢辉. 建筑物理(图解版)[M]. 2版. 北京: 中国建材工业出版社, 2021Yang Chunyu, Tang Mingfang, Xie Hui, et al. Building physics (graphic edition)[M]. 2nd ed. Beijing: China Building Materials Industry Press, 2021 [16] 王成平, 王远东. 建筑材料与检测[M]. 北京: 北京理工大学出版社, 2021Wang Chengping, Wang Yuandong. Building materials and testing[M]. Beijing: Beijing Institute of Technology Press, 2021 [17] 郭山红, 孙锦涛, 谢仁宏, 等. 电磁波穿透墙体的衰减特性[J]. 强激光与粒子束, 2009, 21(1):113-117Guo Shanhong, Sun Jintao, Xie Renhong, et al. Attenuation characteristics of electromagnetic wave penetrating walls[J]. High Power Laser and Particle Beams, 2009, 21(1): 113-117 [18] 赵翔, 茹梦圆, 闫丽萍, 等. 电磁混响室搅拌方式研究综述[J]. 强激光与粒子束, 2020, 32:063001 doi: 10.11884/HPLPB202032.200079Zhao Xiang, Ru Mengyuan, Yan Liping, et al. A review of research on stirring methods of electromagnetic reverberation chamber[J]. High Power Laser and Particle Beams, 2020, 32: 063001 doi: 10.11884/HPLPB202032.200079 [19] 胡明浪, 周世华, 闫丽萍, 等. 基于PWB方法的电大尺寸腔体结构电磁耦合求解器的开发与验证[J]. 强激光与粒子束, 2022, 34:053002 doi: 10.11884/HPLPB202234.220026Hu Minglang, Zhou Shihua, Yan Liping, et al. Development and validation of electromagnetic coupling solver for electrically large-sized cavity structure based on power balance method[J]. High Power Laser and Particle Beams, 2022, 34: 053002 doi: 10.11884/HPLPB202234.220026 [20] Junqua I, Parmantier J P, Issac F. A network formulation of the power balance method for high-frequency coupling[J]. Electromagnetics, 2005, 25(7/8): 603-622. -





 下载:
下载: