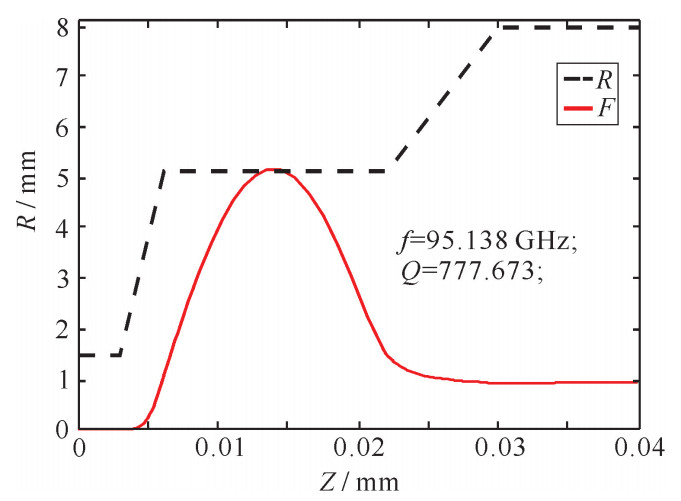
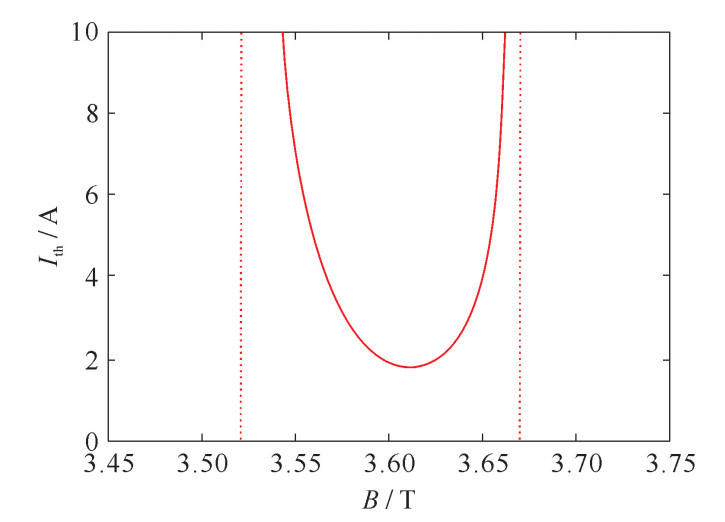
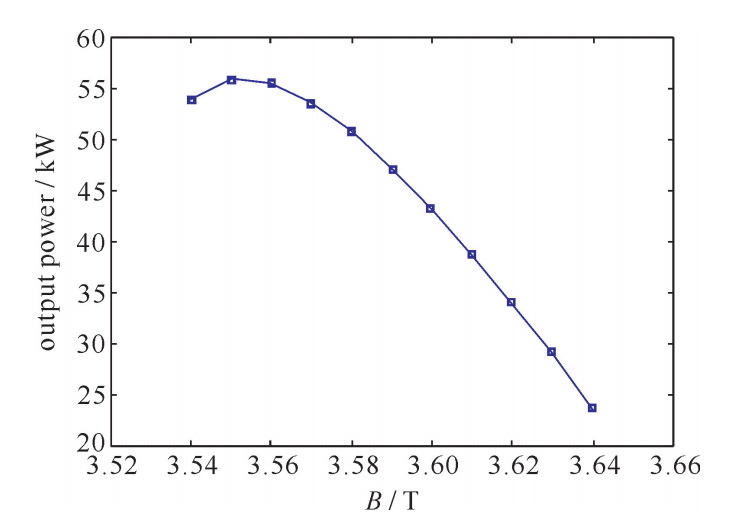
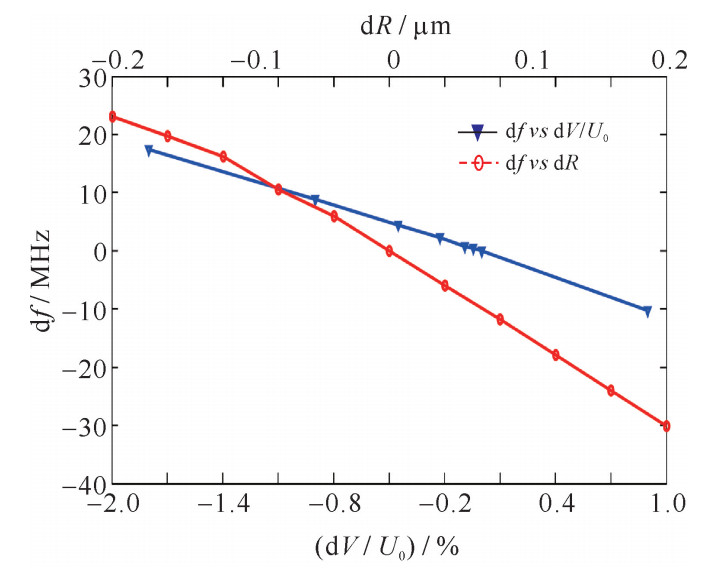
| [1] |
Thumm M. Recent advances in the worldwide fusion gyrotron development[J]. IEEE Trans Plasma Sci, 2014, 42(3): 590-599. doi: 10.1109/TPS.2013.2284026
|
| [2] |
Ergakov V S, Moiseev M A. Theory of synchronization of oscillations in a cyclotron-resonance maser monotron by an external signal[J]. Radiophysics & Quantum Electronics, 1975, 18(1): 89-97.
|
| [3] |
Bazhanov V S, Ergakov V S, Moiseev M A. Synchronization of CRM monotron by electron-beam modulation[J]. Radiophysics & Quantum Electronics, 1977, 20(1): 90-95.
|
| [4] |
Manheimer W M. Theory of the multi-cavity phase locked gyrotron oscillator[J]. International Journal of Electronics, 1987, 63(1): 29-47. doi: 10.1080/00207218708939106
|
| [5] |
Manheimer W M, Fliflet A W, Gold S H, et al. The NRL (Naval Research Laboratory)phase-locked gyrotron oscillator program for SDIO/IST[R]. NRL Memorandum Report 6163, 1998.
|
| [6] |
Gold S H, Fliflet A W, Black W M, et al. Highpower multi-cavity phase-locked gyrotron oscillator experiment[C]//13th Int Conf on Infrared and Millimeter Waves. 1988: 326-327.
|
| [7] |
唐昌建, 杨中海, 刘濮鲲. 高功率回旋管锁相[J]. 强激光与粒子束, 1995, 7(1): 49-56. https://www.cnki.com.cn/Article/CJFDTOTAL-QJGY501.006.htmTang Changjian, Yang Zhonghai, Liu Pukun. Phase-locking of high power gyrotron. High Power Laser and Particle Beams, 1995, 7(1): 49-56 https://www.cnki.com.cn/Article/CJFDTOTAL-QJGY501.006.htm
|
| [8] |
Guo H Z, Hoppe D J, Rodgers J, et al. Phase-locking of a second-harmonic gyrotron oscillator using a quasi-optical circulator to separate injection and output signals[C]//IEEE Int Conf on Plasma Science. 1995: 211-212.
|
| [9] |
Jin Jianbo. Quasi-optical mode converter for a coaxial cavity gyrotron[D]. Chengdu: Southwest Jiaotong University, 2005.
|
| [10] |
刘建卫, 赵青. 准光模式变换器研究与设计[J]. 强激光与粒子束, 2013, 25(10): 2663-2666. doi: 10.3788/HPLPB20132510.2663Liu Jianwei, Zhao Qing. Research and design of quasi-optical mode converter. High Power Laser and Particle Beams, 1995, 7(1): 49-56. doi: 10.3788/HPLPB20132510.2663
|
| [11] |
Danly B G, Temkin R J. Generalized nonlinear harmonic gyrotron theory[J]. Phys Fluids, 1986, 29(2): 561-567.
|
| [12] |
Fliflet A W, Read M E, Chu K R, et al. A self-consistent field theory for gyrotron oscillators: application to a low Q gyromonotron[J]. International Journal of Electronics, 1982, 53(6): 505-521.
|





 下载:
下载: