Numerical study of atmospheric pressure Ar plasma jets under different electrode structures
-
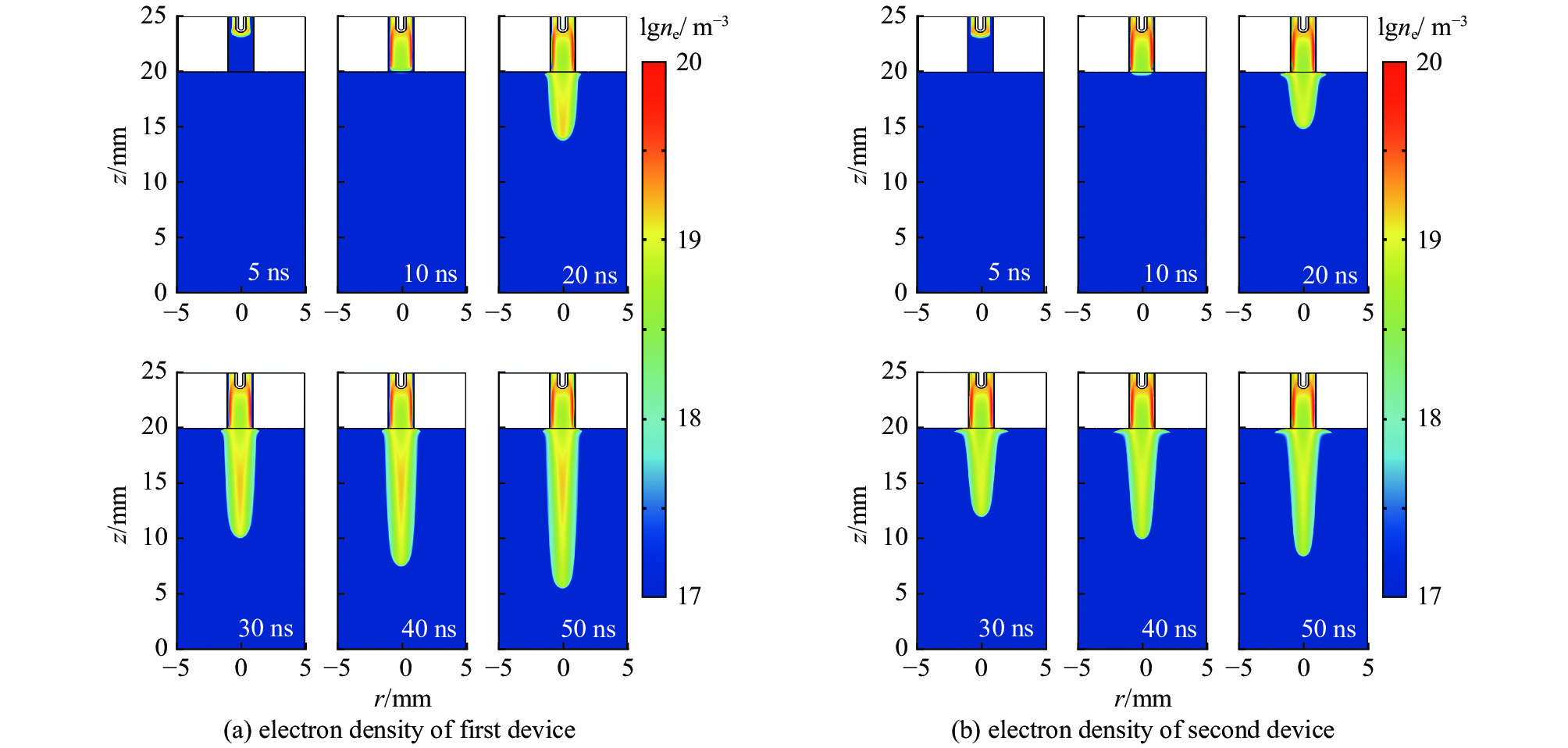
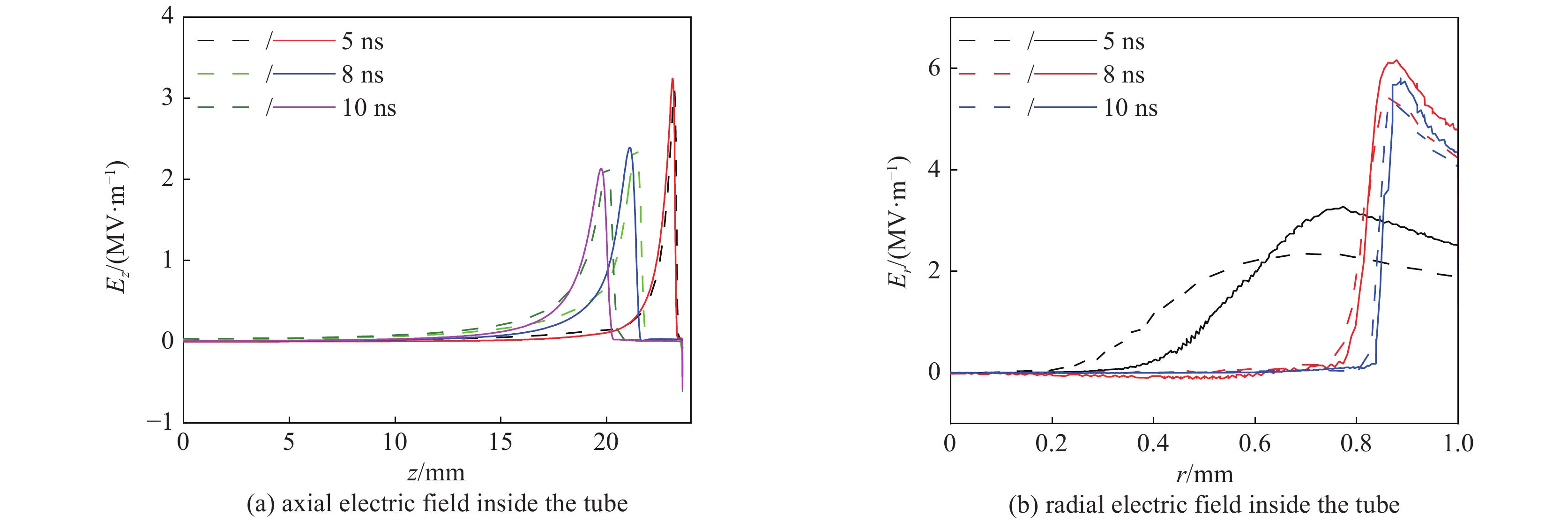
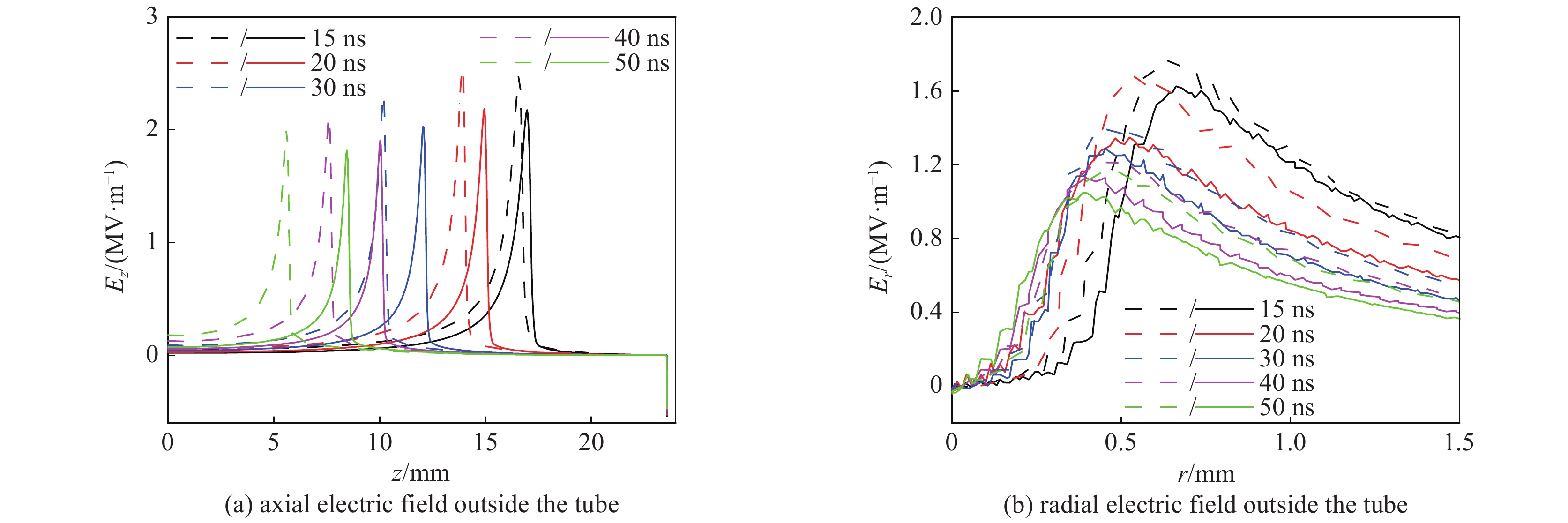
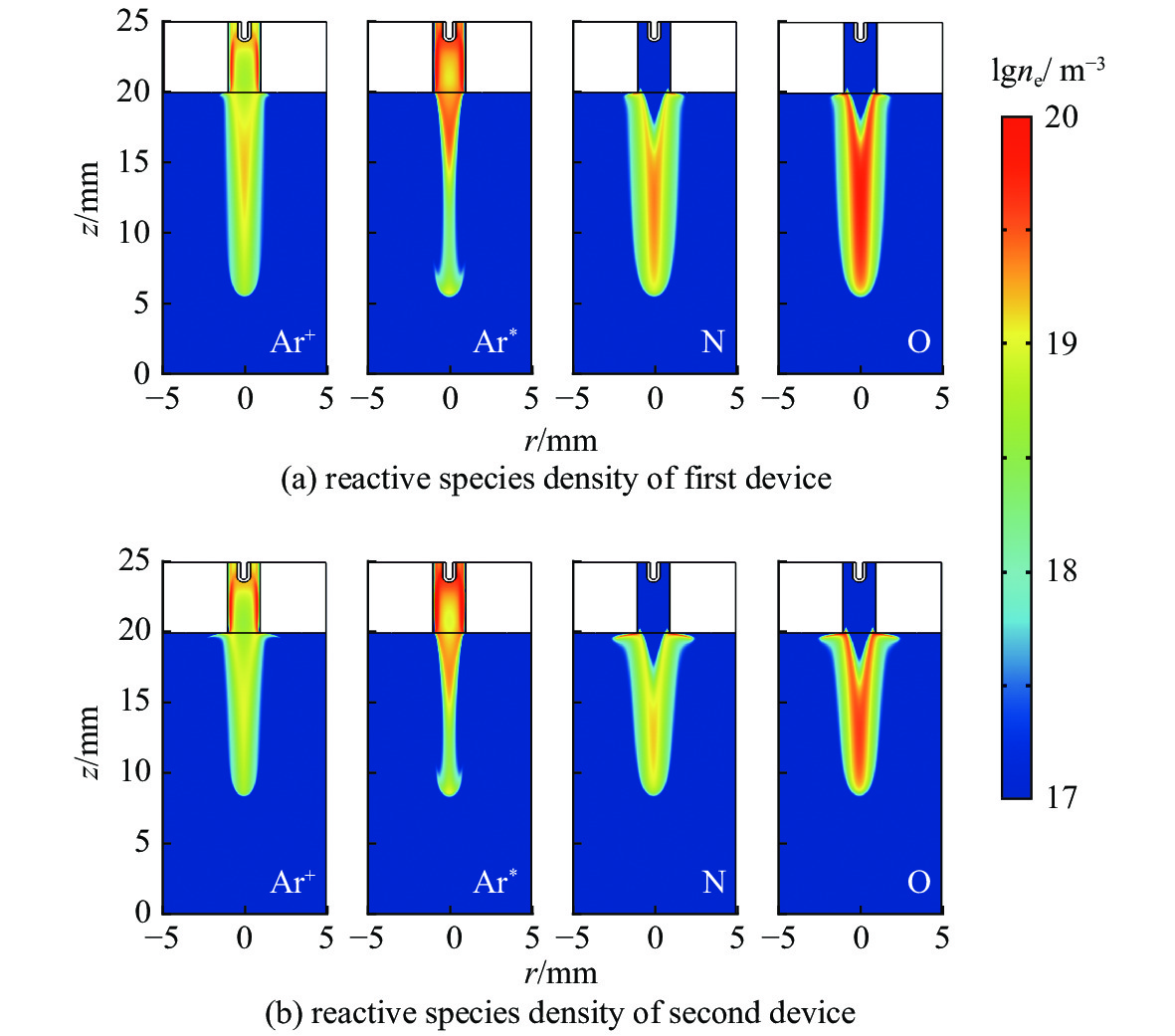
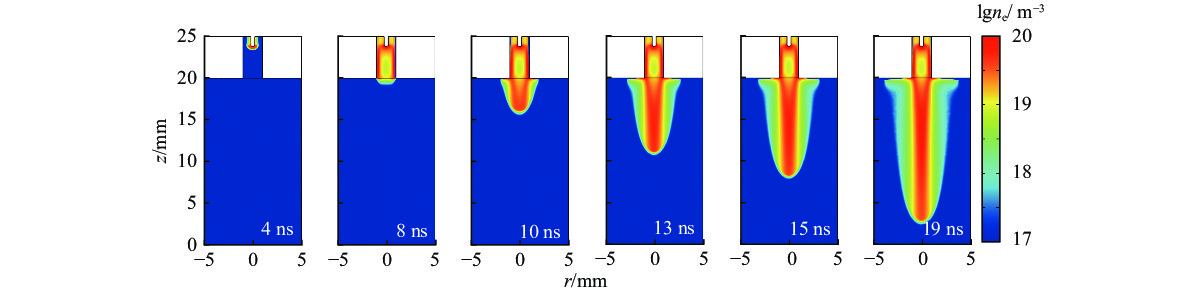
摘要: 采用二维轴对称流体模型对比研究了3种不同电极结构下大气压Ar等离子体射流的基本特性。第一种是带绝缘介质的针电极结构（电场方向和气体流方向平行），第二种是在第一种电极结构的介质管外增加一个垂直气流方向的接地环电极，第三种是不带绝缘介质的裸针电极结构。研究结果表明，接地环电极的引入对介质管内外的射流传播影响不同。在介质管内，接地环电极使管内表面附近的径向电场增加，电子密度升高，射流传播速度加快，但对中心轴附近的电场和电子密度影响很小；然而在介质管外，接地环电极的引入导致轴向和径向电场均减小，从而引起射流的传播长度减小，射流通道径向收缩。通过带绝缘介质的针电极和裸针电极结构的对比研究发现，保持其他条件不变，去掉包裹在针电极上的介质后，由于等离子体电势升高，电场增加，射流的传播长度几乎增加一倍，峰值电子密度增加近一个数量级，而且在整个射流通道内电子密度都保持相对高的值。此外，对3种电极结构下的主要活性粒子的产生和输运进行了比较研究。
-
关键词:
- 大气压Ar等离子体射流 /
- 电极结构 /
- 二维模拟 /
- 活性粒子
Abstract: In this paper, the basic properties of the atmospheric pressure Ar plasma jet with three different electrode structures are comparatively studied using a two-dimensional axisymmetric fluid model. The results show that the introduction of the grounded ring electrode affects the jet propagation both inside and outside the dielectric tube. Inside the dielectric tube, the grounded ring electrode increases the radial electric field near the inner surface of the tube, leading to the increase of the electron density and jet propagation length. However, it has a slight effect on the electric field and electron density near the central axis. Outside the dielectric tube, the introduction of the grounded ring electrode results in the reduction of the electric field both in axial and radial directions. This inevitably causes the decrease of the jet propagation length and a contraction of the jet channel radius. For the bare needle electrode structure, the removal of the medium wrapped around the needle electrode increases the electric field due to the elevated plasma potential. This makes the increase of the jet propagation length. The peak electron density in the jet channel increases about one order of magnitude. Meanwhile, the electron density in the whole channel is relatively higher in the bare needle electrode structure. In addition, the production and transport of the main active particles under the three electrode structures are comparatively studied. -
图 1 计算中采用的装置示意图和模拟域
Figure 1. Discharge device and simulation domain used in our calculation
图 3 第一种和第二种电极装置下电子密度的时空演化
Figure 3. Temporal and spatial evolution of electron density for the first and the second electrode device
图 4 不同电极结构下径向和轴向电子密度时间演化
Figure 4. Radial and axial electron density evolutions for different electrode device
Note: dashed line: the first electrode device; solid line: the second electrode device.
图 5 电离波在介质管中传播时不同电极结构下电离头处轴向电场和径向电场演化
Figure 5. Evolution of the axial and radial electric field in the ionization head when the ionization wave propagates inside the tube for different electrode device
图 6 电离波在介质管外传播时不同电极结构下电离头处轴向电场和径向电场演化
Figure 6. Evolution of the axial and radial electric field in the ionization head when the ionization wave propagates outside the tube for different electrode device
图 7 不同电极结构下50 ns时活性粒子的空间分布
Figure 7. Spatial distribution of reactive species density at 50 ns for two electrode devices
图 8 两种电极装置（分别用虚线和实线表示）下活性粒子轴线数密度演化
Figure 8. Evolution reactive species density on the axis for two electrode devices
图 9 裸针电极装置下电子密度的时空演化
Figure 9. Temporal-spatial evolution of electron density for bare needle electrode device
图 10 裸针电极装置下轴向电子密度演化
Figure 10. Axial electron density evolution for the bare needle electrode device
图 11 裸针电极装置下电离波传播过程中电离头处轴向电场和径向电场的演化
Figure 11. Evolution of the electric field during the propagation of the ionization wave under the bare needle electrode device
图 12 裸针电极（实线）和带绝缘介质针电极（虚线）结构下轴向等离子体电势分布
Figure 12. Axial plasma potential distribution at different moments for the bare needle electrode (solid line) and needle electrode with insulation dielectric (dashed line)
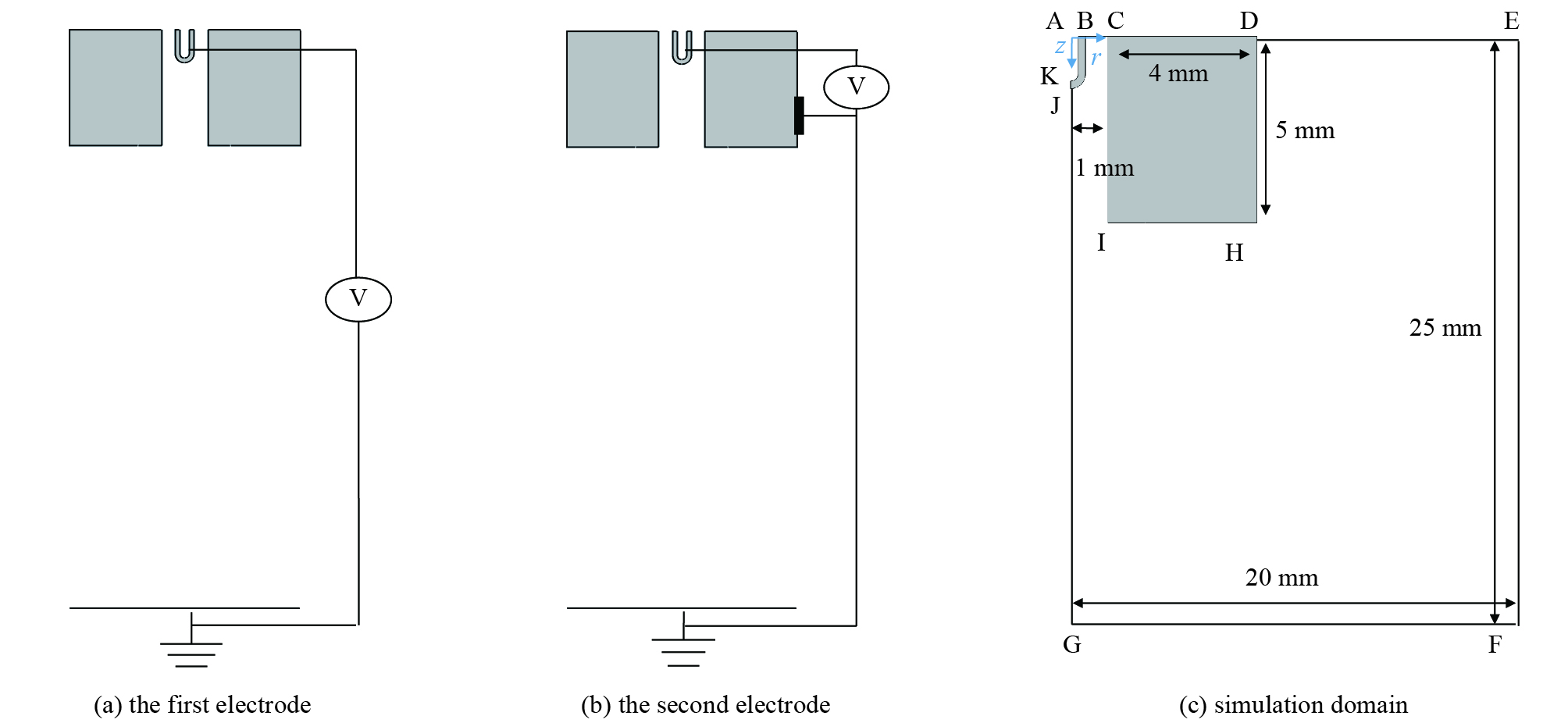
表 1 中性气体流计算边界条件
Table 1. Boundary conditions for the neutral gas flow model
boundary velocity condition background species condition BC ${u_{ {\textit{z}} } } = {u_0},{u_{{r} } } = 0$ ${w_{\rm{Ar} } } = 0.999, {w_{\rm{air} } } = 0.001$ BJ,CI,IH,HD,GF ${u}_{ {\textit{z}} }=0,{u}_{{r} }=0$ ${{n} } \cdot { {{J} }_{{i} } } = 0$ DE ${u}_{ {\textit{z}} }=0.03{u}_{0},{u}_{{r} }=0$ ${w_{\rm{air}}} = 1$ KG ${u_{{r} } } = 0$ $\dfrac{ {\partial {w_{{i} } } }}{ {\partial r} } = 0$ EF 0.1 MPa ${{n} } \cdot { {{J} }_{{i} } } = \rho {w_{{i} } }{u_{{r} } }$ Note: ${u_{{r}}}$ and ${u_{ {\textit{z}} } }$ are the velocity in the axial and radial directions, respectively. ${{n}}$ is the unit vector pointing toward the boundary. 
下载: 导出CSV
表 2 等离子体模块边界条件
Table 2. Boundary conditions for the plasma model
boundary electrostatic condition species condition BC $\dfrac{{\partial \varPhi }}{{\partial {\textit{z}}}} = 0$ $\dfrac{ {\partial {n_{\rm{e} } } }}{ {\partial {\textit{z} } } } = \dfrac{ {\partial {n_{\rm{\varepsilon} } } } }{ {\partial {\textit{z} } } } = 0,\;\dfrac{ {\partial {n_{\rm{m} } } }}{ {\partial {\textit{z} } } } = 0,\;\dfrac{ {\partial {n_{\rm{i} } } }}{ {\partial {\textit{z} } } } = 0$ BJ， CIHD Eq.(13) Eq.(8)， (9)， (10)， (11) KG $\dfrac{{\partial \varPhi }}{{\partial r}} = 0$ $\dfrac{ {\partial {n_{\rm{e} } } }}{ {\partial r} } = \dfrac{ {\partial {n_{\rm{\varepsilon} } } } }{ {\partial r} } = 0,\;\dfrac{ {\partial {n_{\rm{m} } } }}{ {\partial r} } = 0,\;\dfrac{ {\partial {n_{\rm{i} } } }}{ {\partial r} } = 0$ AK V … GF 0 —— EF 0 $\dfrac{ {\partial {n_{\rm{e} } } }}{ {\partial r} } = \dfrac{ {\partial {n_{\rm{\varepsilon} } } } }{ {\partial r} } = 0,\;\dfrac{ {\partial {n_{\rm{m} } } }}{ {\partial r} } = 0,\;\dfrac{ {\partial {n_{\rm{i} } } }}{ {\partial r} } = 0$ ring 0 —— Note: “ring” is the high voltage electrode.
下载: 导出CSV
表 3 模拟中采用的化学反应
Table 3. Chemistry reactions used in the simulation
index reaction rate coefficients threshold energy/eV reference 1 e＋Ar→e＋Ar BOLSIG＋ / [17] 2 e＋Ar→e＋Ar* BOLSIG＋ 11.5 [17] 3 e＋Ar→2e＋Ar+ BOLSIG＋ 15.8 [17] 4 e＋N2→2e＋N2+ BOLSIG＋ 15.58 [17] 5 e＋N2→e＋2N BOLSIG＋ 13 [17] 6 e＋N2→e＋N2(C3π) BOLSIG＋ 11.03 [17] 7 e＋O2→2e＋O2+ BOLSIG＋ 12.06 [17] 8 e＋O2→e＋2O BOLSIG＋ 5.58 [17] 9 e＋O2→O2− BOLSIG＋ / [17] 10 Ar*＋Ar*→e＋Ar＋Ar+ 6.4×10−16 (m−3/s) / [17] 11 Ar*＋Ar→Ar＋Ar 2.09×10−21 (m−3/s) / [17] 12 Ar*＋N2→Ar＋2N 3.6×10−17 (m−3/s) / [17] 13 Ar*＋O2→Ar＋2O 2.1×10−16 (m−3/s) / [17]
下载: 导出CSV
-
[1] Belmonte T, Pintassilgo C D, Czerwiec T, et al. Oxygen plasma surface interaction in treatments of polyolefines[J]. Surface and Coatings Technology, 2005, 200(1/4): 26-30. [2] Baik K Y, Kang H L, Kim J, et al. Non-thermal plasma jet without electrical shock for biomedical applications[J]. Applied Physics Letters, 2013, 103: 164101. doi: 10.1063/1.4825206 [3] Kim K, Ahn H J, Lee J H, et al. Cellular membrane collapse by atmospheric-pressure plasma jet[J]. Applied Physics Letters, 2014, 104: 013701. doi: 10.1063/1.4861373 [4] Naidis G V. Modelling of streamer propagation in atmospheric-pressure helium plasma jets[J]. Journal of Physics D: Applied Physics, 2010, 43: 402001. doi: 10.1088/0022-3727/43/40/402001 [5] Yan Wen, Economou D J. Simulation of a non-equilibrium helium plasma bullet emerging into oxygen at high pressure (250–760 Torr) and interacting with a substrate[J]. Journal of Applied Physics, 2016, 120: 123304. doi: 10.1063/1.4963115 [6] Lu Xinpei, Naidis G V, Laroussi M, et al. Reactive species in non-equilibrium atmospheric-pressure plasmas: generation, transport, and biological effects[J]. Physics Reports, 2016, 630: 1-84. doi: 10.1016/j.physrep.2016.03.003 [7] 张冠军, 詹江杨, 邵先军, 等. 大气压氩气等离子体射流长度的影响因素[J]. 高电压技术, 2011, 37(6):1432-1438. (Zhang Guanjun, Zhan Jiangyang, Shao Xianjun, et al. Influence factor analysis on jet length of atmospheric pressure argon plasma jets[J]. High Voltage Engineering, 2011, 37(6): 1432-1438 [8] Zhang Bo, Zhu Ying, Liu Feng, et al. The influence of grounded electrode positions on the evolution and characteristics of an atmospheric pressure argon plasma jet[J]. Plasma Science and Technology, 2017, 19: 064001. doi: 10.1088/2058-6272/aa629f [9] Yue Yuanfu, Pei Xuekai, Lu Xinpei. Comparison on the absolute concentrations of hydroxyl and atomic oxygen generated by five different nonequilibrium atmospheric-pressure plasma jets[J]. IEEE Transactions on Radiation and Plasma Medical Sciences, 2017, 1(6): 541-549. doi: 10.1109/TRPMS.2017.2757037 [10] Xiong Zhongmin, Kushner M J. Atmospheric pressure ionization waves propagating through a flexible high aspect ratio capillary channel and impinging upon a target[J]. Plasma Sources Science and Technology, 2012, 21: 034001. doi: 10.1088/0963-0252/21/3/034001 [11] Maletić D, Puač N, Selaković N, et al. Time-resolved optical emission imaging of an atmospheric plasma jet for different electrode positions with a constant electrode gap[J]. Plasma Sources Science and Technology, 2015, 24: 025006. doi: 10.1088/0963-0252/24/2/025006 [12] Walsh J L, Kong M G. Contrasting characteristics of linear-field and cross-field atmospheric plasma jets[J]. Applied Physics Letters, 2008, 93: 111501. doi: 10.1063/1.2982497 [13] Yan Wen, Liu Fucheng, Sang Chaofeng, et al. Two-dimensional numerical study of an atmospheric pressure helium plasma jet with dual-power electrode[J]. Chinese Physics B, 2015, 24: 065203. doi: 10.1088/1674-1056/24/6/065203 [14] Judée F, Merbahi N, Wattieaux G, et al. Analysis of Ar plasma jets induced by single and double dielectric barrier discharges at atmospheric pressure[J]. Journal of Applied Physics, 2016, 120: 114901. doi: 10.1063/1.4961037 [15] Van Gaens W, Bruggeman P J, Bogaerts A. Numerical analysis of the NO and O generation mechanism in a needle-type plasma jet[J]. New Journal of Physics, 2014, 16: 063054. doi: 10.1088/1367-2630/16/6/063054 [16] Xu Han, Chen Chen, Liu Dingxin, et al. Contrasting characteristics of aqueous reactive species induced by cross-field and linear-field plasma jets[J]. Journal of Physics D: Applied Physics, 2017, 50: 245201. doi: 10.1088/1361-6463/aa7118 [17] Van Gaens W V, Bogaerts A. Corrigendum: kinetic modelling for an atmospheric pressure argon plasma jet in humid air (2013 J. Phys. D: Appl. Phys. 46 275201)[J]. Journal of Physics D: Applied Physics, 2014, 47: 079502. doi: 10.1088/0022-3727/47/7/079502 [18] Hagelaar G J M, Pitchford L C. Solving the Boltzmann equation to obtain electron transport coefficientsand rate coefficients for fluid models[J]. Plasma Sources Science and Technology, 2005, 14(4): 722-733. doi: 10.1088/0963-0252/14/4/011 [19] https://us.lxcat.net/data/set/data/set_type.php [20] Ellis H W, Pai R Y, McDaniel E W, et al. Transport properties of gaseous ions over a wide energy range[J]. Atomic Data and Nuclear Data Tables, 1976, 17(3): 177-210. doi: 10.1016/0092-640X(76)90001-2 [21] Breden D, Miki K, Raja L L. Computational study of cold atmospheric nanosecond pulsed helium plasma jet in air[J]. Applied Physics Letters, 2011, 99: 111501. doi: 10.1063/1.3636433 [22] Breden D, Raja L L. Computational study of the interaction of cold atmospheric helium plasma jets with surfaces[J]. Plasma Sources Science and Technology, 2014, 23: 065020. doi: 10.1088/0963-0252/23/6/065020 [23] Wang Lijun, Zheng Yashuang, Jia Shenli. Numerical study of the interaction of a helium atmospheric pressure plasma jet with a dielectric material[J]. Physics of Plasmas, 2016, 23: 103504. doi: 10.1063/1.4964482 [24] Yan Wen, Economou D J. Gas flow rate dependence of the discharge characteristics of a helium atmospheric pressure plasma jet interacting with a substrate[J]. Journal of Physics D: Applied Physics, 2017, 50: 415205. doi: 10.1088/1361-6463/aa8794 [25] Jánský J, Le Delliou P, Tholin F, et al. Experimental and numerical study of the propagation of a discharge in a capillary tube in air at atmospheric pressure[J]. Journal of Physics D: Applied Physics, 2011, 44: 335201. doi: 10.1088/0022-3727/44/33/335201 -
点击查看大图
计量
- 文章访问数: 1091
- HTML全文浏览量: 407
- PDF下载量: 80
- 被引次数: 0
